4
$\begingroup$

What is the name/type/search term I can use to find similar puzzles to the one below (so I can practice them, as I really struggle currently wrapping my head around them)?

Any type of question where you have to interpret an unsual way of presenting data

I have tried:

  • complex data representation (but this mainly shows business charts)
  • abstract reasoning (but this mainly yields "Spot whats next in the pattern")

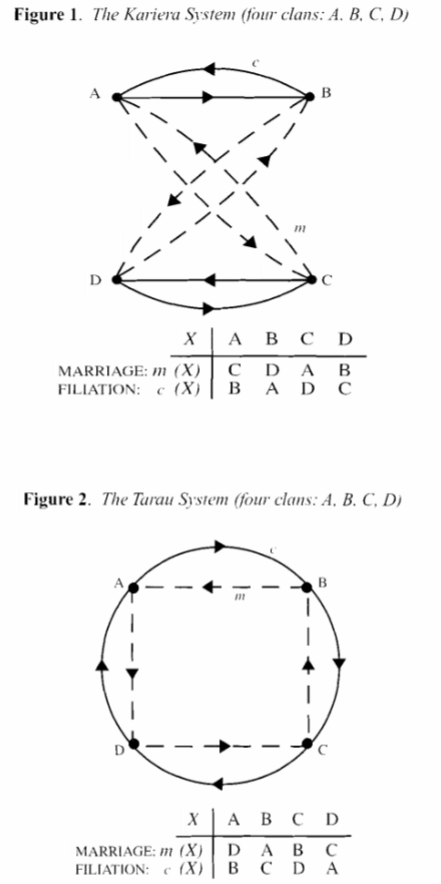
An example of the type of puzzle which I am seeking the name for is below:

  • Solid lines indicate a child of a father from the clan at the origin of the arrow becomes a member of the clan to which the arrow points.
  • Broken lines indicate allowed marriage relationships where any man from the clan at the origin of the arrow may marry a woman of the clan to which the arrow points.
  • A man of clan X has to marry a woman of clan m(X).
  • A child of a man of clan X will be of a clan c(X).

In both systems, individuals belong to the clan of:
A) one parent, B) their aunts, C) their uncles, D) their sibling

enter image description here

Improve this question
$\endgroup$
9
  • 2
    $\begingroup$ What's the puzzle? I can see the setup for a puzzle, but it's missing any statement of something to solve. $\endgroup$
    – Peter Taylor
    Commented Nov 24, 2016 at 6:53
  • 1
    $\begingroup$ The puzzle is "In both systems, individuals belong to the clan of: A) one parent, B) their aunts, C) their uncles D) their sibling" $\endgroup$
    – K-Feldspar
    Commented Nov 24, 2016 at 6:53
  • $\begingroup$ I thought you were making a joke first and cracked up. But if you are serious. Thank you! Though I didn't really mean problems relating to only family. More about problems where you need to interpret any unusual form of data representation. $\endgroup$
    – K-Feldspar
    Commented Nov 24, 2016 at 10:54
  • 1
    $\begingroup$ I don't know of a term for these (which is very little evidence that there isn't one), but they strike me more as the sort of thing you find in intelligence/aptitude tests, rather than puzzles as such. $\endgroup$
    – Gareth McCaughan ♦
    Commented Nov 24, 2016 at 12:32
  • 1
    $\begingroup$ Well, @wizzwizz4, it wasn't much of an answer. I didn't think this question really belonged here because it doesn't have anything to do with resolving or building puzzles, but I initially chose to give the information in an answer rather than a comment. $\endgroup$
    – can-ned_food
    Commented May 5, 2018 at 3:03

2 Answers 2

Reset to default
1
$\begingroup$

I did a bit of digging around on Bing, I believe they are called:

Prescriptive marriage systems

Some clues:

Further research has taken me further, and leads to an answer!

We discover a whole set of beliefs around kinship, generated by tribes who believe in a human spirit and a parallelism of people with animals.

Such as Australian-Aborigines. This article, which describes the broader ideas of kinship that are involved, uses images like the ones you used in your question (see Martuthunira and Pintupi).

One of the concepts (key to my answer) is that of parallel and cross cousins.

a parallel cousin or ortho-cousin is a cousin from a parent's same-sex sibling, while a cross-cousin is from a parent's opposite-sex sibling

The Kariera tribe gets its own page, while there is very little on the Tarau tribe anywhere. A link from references (Collected Works of James G. Frazer) produces:

with Chapter 4 being on Totemism.

Several names appear repeatedly, one being:

who appeared to have been the authority on these matters.

After taking all this in, your question begins to make sense.

The answer is:

D. Sibling

because:

The question is asking for the ONLY relationship that belongs to the same clan. Remember A can marry B and their child belongs to C (which removes one parent, aunts and unlces from the possibilites), BUT if they have more than one child (siblings), the siblings belong to the same clan, and so D.

Improve this answer
$\endgroup$
0
$\begingroup$

Topology is the study of relationships between objects in a network. It is a subset of set theory, which is an aspect of ontology.
More precisely, you are describing a ‘sociogram’.

So far as I understand, your question doesn't actually ask for anything that I would consider to concern the resolution or building of puzzles, but we could easily devise a form of logic puzzle which presented you with various people, qualified as nodes in a network, and their relationships to other people.

As an example, let's say that you are given a list of people. With each person you are given one typified relationship to one other person in that same list. You are tasked to classify each person in one of two sets: whether a person is eligible or ineligible to wed a select person X.
First, you would need to construct a topological graph — i.e. a sociogram — which allowed you to deduce the unknown relationships between persons.
Then, based on whatever rules the puzzle states for us as to what criteria make two people ineligible to wed, the puzzle can be solved if you can map every node to one and only one of those two classes for any arbitrarily selected person X — or, at the very least, to a only one certain person X given in the conditions of the puzzle.

Because I didn't really answer your question, I'll list where further information can be obtained by researching:

  • General topology
    If you research ‘network topology’ you are more likely to encounter the specialist usage of the word ‘network’ — pertaining to computer and signal technology — rather than the generalist, mathematical one.
  • Graph theory
    • Crossing number
  • Knot theory
  • Mathematical puzzles
  • Disentanglement, a.k.a. topological, puzzles
    E.g. the renowned tavern puzzles of topologically interlocked pieces of forged iron or bronze.
  • “Three utilities problem”
    You have three houses arranged as points on a straight line. On a parallel Euclidean line are three other points representing Water, Electricity, and Gas — or Heating Oil or Steam or whatever you please. Can each house be connected to each utility service by pipes and wires which neither cross nor leave the plane containing the two parallel lines?
Improve this answer
$\endgroup$
1
  • $\begingroup$ That's not related to what the question is asking at all. $\endgroup$
    – Deusovi ♦
    Commented Feb 23, 2017 at 17:48

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.