38
$\begingroup$

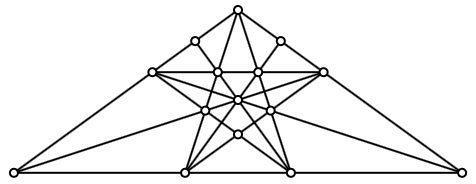
Alice enjoys placing magnets on a magnetized whiteboard.
This day, she placed all 16 magnets in her possession on the board in a rectangular fashion.

o  o  o  o
o  o  o  o
o  o  o  o
o  o  o  o

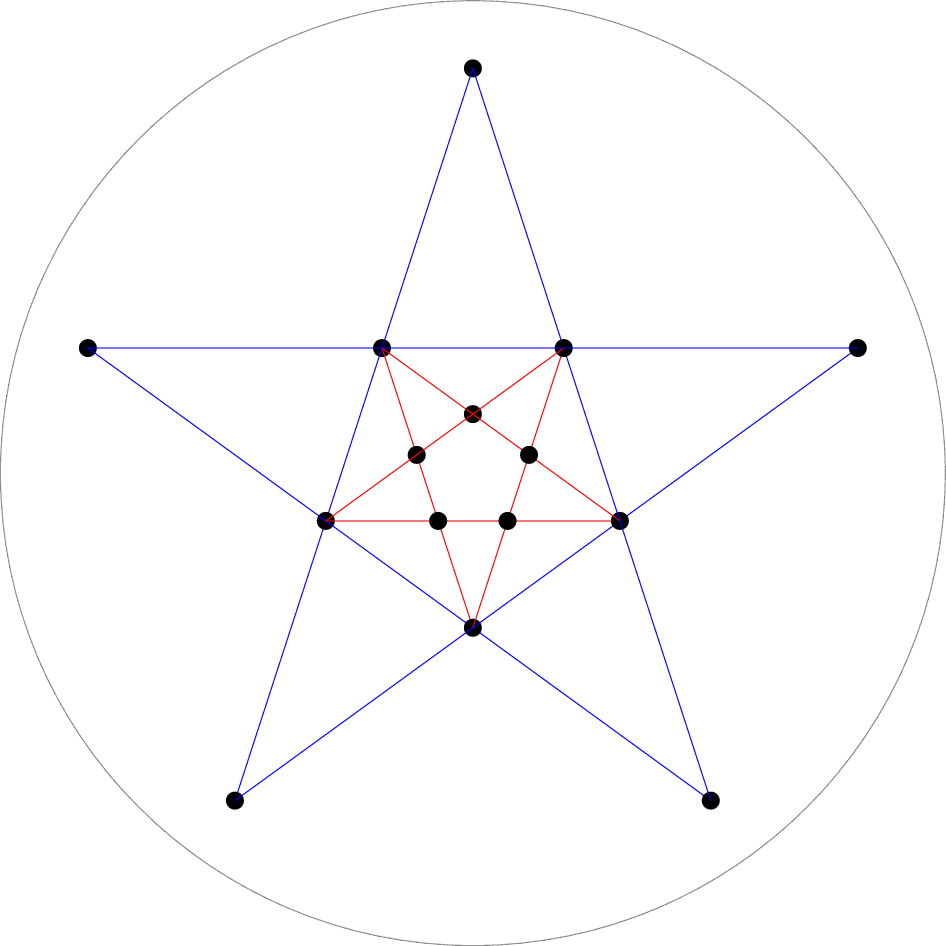
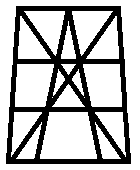
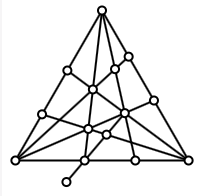
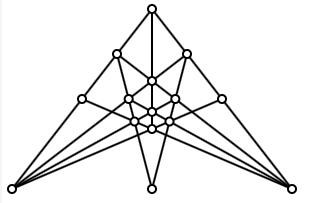
"Sweet, that makes exactly 10 lines of 4 magnets" said Alice.
But Xelia, her evil twin sister, showed up to remove one of the magnets.

"Hey, give the magnet back !" gasped Alice.
"I may do that ..." replied Xelia. "But only if you can make 10 lines of 4 magnets with what's remaining on the board."

Can Alice recover the lost magnet without resorting to violent ways ?

Improve this question
$\endgroup$
6
  • 3
    $\begingroup$ Don't know if this is a valid solution, but placing two parallell rows with $5$ magnets would give $2{5 \choose 4}$ possible lines (although the same lines) made up by $4$ magnets. The remaing magnets is placed so that they don't make a line of 4 with any other magnets. $\endgroup$
    – Carl Löndahl
    Commented Jul 5, 2016 at 11:50
  • 1
    $\begingroup$ @CarlLöndahl Nothing in the question forbids that, so why not. It's just that Xelia may not lend back the magnet in front of such cheapness ;) $\endgroup$
    – Red-Jeweled
    Commented Jul 5, 2016 at 11:54
  • 1
    $\begingroup$ Similar: puzzling.stackexchange.com/questions/29848, puzzling.stackexchange.com/questions/20163 $\endgroup$
    – f''
    Commented Jul 5, 2016 at 12:18
  • $\begingroup$ @Anton that was my concern :-) $\endgroup$
    – Carl Löndahl
    Commented Jul 5, 2016 at 12:22
  • $\begingroup$ @f'' The inspiration came from this question, which I'm still trying to solve : puzzling.stackexchange.com/questions/1867/… $\endgroup$
    – Red-Jeweled
    Commented Jul 5, 2016 at 12:23

5 Answers 5

Reset to default
43
$\begingroup$

This is a solution

using pentagons...
A solution

Improve this answer
$\endgroup$
3
  • 4
    $\begingroup$ Note that, by adding the stolen magnet in the middle, you create 5 additional lines, so it gives a solution to make 15 lines of four with 16 magnets. $\endgroup$
    – Xoff
    Commented Jul 5, 2016 at 13:11
  • 18
    $\begingroup$ Also, the resulting shape seems to suggest extra meaning to the "evil sister" part of the question. $\endgroup$
    – Bradley Uffner
    Commented Jul 5, 2016 at 14:11
  • 7
    $\begingroup$ @BradleyUffner It was destined to be : As she places the 15th magnet on the board, Alice unintentionally completes the ritual and is now a worshiper. $\endgroup$
    – Red-Jeweled
    Commented Jul 5, 2016 at 20:18
7
$\begingroup$

You can just line up all the magnets in a line and keep the extra ones in the next row.

By doing

o o o o o o o o o o o o o
o o

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ @Block Just curious, how does this fail to answer the question? There appear to be 10 lines of 4 in it starting from the left hand side of the long line and counting 4 to the right then moving over once and starting again. $\endgroup$
    – Lunin
    Commented Jul 5, 2016 at 20:46
  • 1
    $\begingroup$ Nothing in the question says that every line must be distinct. It's the cheapest way, but it's still a valid answer. $\endgroup$
    – Red-Jeweled
    Commented Jul 5, 2016 at 20:47
  • 4
    $\begingroup$ I see 25740 (coincident) lines of 4 magnets, not 10. $\endgroup$
    – JeffE
    Commented Jul 5, 2016 at 21:09
  • 4
    $\begingroup$ So Alice may be a bit of an overachiever, but it appears a valid solution of the problem as stated. $\endgroup$
    – Penguino
    Commented Jul 5, 2016 at 23:43
  • 2
    $\begingroup$ A line defined by two points is, by definition, infinite in both directions. So a line through four of the magnets in the top row will go through all of the magnets (in that row). The ten lines would therefore coincide. If the question would be about line segments, then the answer would be valid. $\endgroup$
    – Block
    Commented Jul 6, 2016 at 8:28
2
$\begingroup$

[edit: This solution is wrong, as one of my lines has only 3 magnets in it]

Here's a picture of one way to do it.

10 lines of 4 Draw the lines around and through the centre trapezoid first, then horizontal top and bottom lines, and then the sloping left and right lines. Each time you draw a line there are no more than 2 points already fixed that the line has to go through, so they can all be straight lines.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I'm not sure, but I think you only have 9 lines. $\endgroup$
    – Marius
    Commented Jul 5, 2016 at 11:30
  • 1
    $\begingroup$ Indeed, 1 line is made of 3 magnets, which invalidates the solution, unfortunately. $\endgroup$
    – Red-Jeweled
    Commented Jul 5, 2016 at 11:31
  • $\begingroup$ Oh dear. Never mind. $\endgroup$
    – Jaap Scherphuis
    Commented Jul 5, 2016 at 11:33
2
$\begingroup$

I can do ten lines. It starts nicely but ends funny.

enter image description here

Or eleven lines.

enter image description here

Or even twelve lines.

enter image description here

Xelia owes Alice three magnets now...

Improve this answer
$\endgroup$
1
0
$\begingroup$

1. Cut one of the magnets in half.
2. Recreate the original pattern.

Improve this answer
$\endgroup$
2
  • 9
    $\begingroup$ "without resorting to violent ways" - this is pretty violent as far as the magnet is concerned! $\endgroup$
    – SeanR
    Commented Jul 5, 2016 at 16:30
  • 1
    $\begingroup$ We're not here to teach violence to magnets or other people, animals, objects or beliefs! We're here to create puzzles in peace, not in violence. Cutting a magnet in half essentially kills it, which is considered a 1st degree felony. Also, if it survives, the two halves remain separate forever, because of magnetic force. Think that a magnet is your bff. Would you cut them in half for no reason? Would you want to unfriend them for no reason? If you answered yes to the first question, you're a criminal. If you answered yes to the second question, you're a loner. Save The Magnets™! $\endgroup$
    – EKons
    Commented Jul 10, 2016 at 7:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.