13
$\begingroup$

Goal of Hidato:

Fill in a grid with a series of consecutive numbers that connect each other horizontally, vertically, or diagonally.

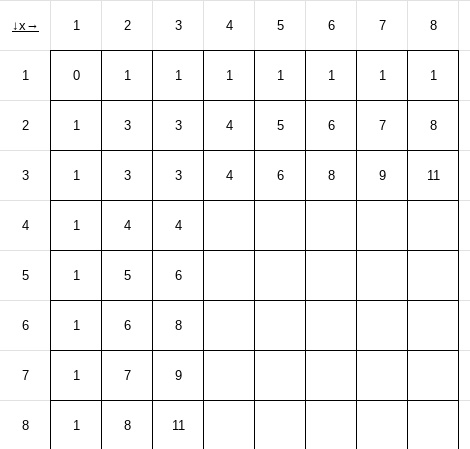
So I have been working on figuring out the minimum number of clues needed for an $n\times m$ Hidato puzzle such that it had a unique solution. (up to the $8\times8$ case)

Here is the table I have made which lists the lower bounds of clues needed for a Hidato puzzle of size $n\times m$ to have a unique solution:

enter image description here

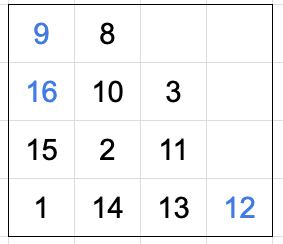
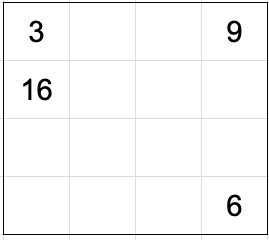
I am currently trying to figure out the lower bounds for $4\times m$ Hidato puzzles where $m\in\mathbb N$ and $4\le m\le8$, and would like to know the minimum amount of clues needed for a $4\times4$ Hidato puzzle to have a unique solution.

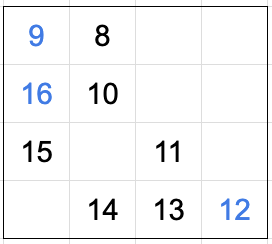
I have managed to create one with 8 clues

enter image description here

however I would like to know:

Is 8 is truly the minimum needed, or is it possible to have a uniquely solvable 4x4 Hidato puzzle with 7 clues?

Improve this question
$\endgroup$
10
  • 1
    $\begingroup$ Is the solution unique? It looks there are two solutions where '6' and '16' could be swapped to get from one to the other. $\endgroup$
    – hexomino
    Commented Mar 5 at 17:44
  • 1
    $\begingroup$ Wait a sec, does that mean I have accidentally answered my own question? because then we could remove the 5 to get a 4x4 Hidato puzzle with a unique solution with only 7 clues? $\endgroup$
    – CrSb0001
    Commented Mar 5 at 17:48
  • 2
    $\begingroup$ For $3\times3$, the minimum is $2$ (not $3$): \begin{matrix} . & . & . \\ . & . & 9 \\ 7 & . &. \\ \end{matrix} $\endgroup$
    – RobPratt
    Commented Mar 6 at 17:41
  • 2
    $\begingroup$ For $3\times4$, the minimum is $2$ (not $4$): \begin{matrix}.&.&.&.\\.&.&.&.\\.&.&11&6\\ \end{matrix} $\endgroup$
    – RobPratt
    Commented Mar 7 at 0:01
  • 2
    $\begingroup$ I'm pretty sure this works for 3 x 7: \begin{array} {c} . & . & 16 & . & 5 & . & . \\ . & . & . & . & . & . & . \\ . & 18 & . & . & . & . & 3 \\ \end{array} $\endgroup$
    – Nitrodon
    Commented Mar 7 at 15:05

3 Answers 3

Reset to default
23
$\begingroup$

Update: I think 3 is possible:

3 soln

Solving this is as follows:

First, add the diagonals and the 8 is then forced.
s1
Next, there's only one way to connect the 12 and the 16 without creating an island:
s2
Next, there's a deadend, that must have a 1
s3
And you're done
done

I think 4 is possible. I believe this has a unique solution. (Oh, just saw that someone posted something similar)

4 soln

Improve this answer
$\endgroup$
4
  • 3
    $\begingroup$ Both the 4-clue puzzle and 3-clue puzzle have a unique solution, good job! $\endgroup$
    – CrSb0001
    Commented Mar 5 at 18:55
  • 1
    $\begingroup$ We're trying for the minimum, right? Not specifically 7-clue. $\endgroup$
    – Dr Xorile
    Commented Mar 5 at 19:01
  • 1
    $\begingroup$ Yes, we are trying for the minimum. 7 clues was just my proposed lower bound. $\endgroup$
    – CrSb0001
    Commented Mar 5 at 19:02
  • 5
    $\begingroup$ That solution with three is very nice. Good explanation +1 $\endgroup$
    – hexomino
    Commented Mar 5 at 19:39
7
$\begingroup$

Here is a 4x4 Hidato with 6 clues which I believe has a unique solution

enter image description here

Improve this answer
$\endgroup$
0
5
$\begingroup$

What about this?

..A4
....
....
1..7

(A is 10)

For 3x4, what about this?

3..
c..
..1
...

Improve this answer
$\endgroup$
1
  • $\begingroup$ I have confirmed that both have a unique solution. $\endgroup$
    – CrSb0001
    Commented Mar 5 at 18:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.