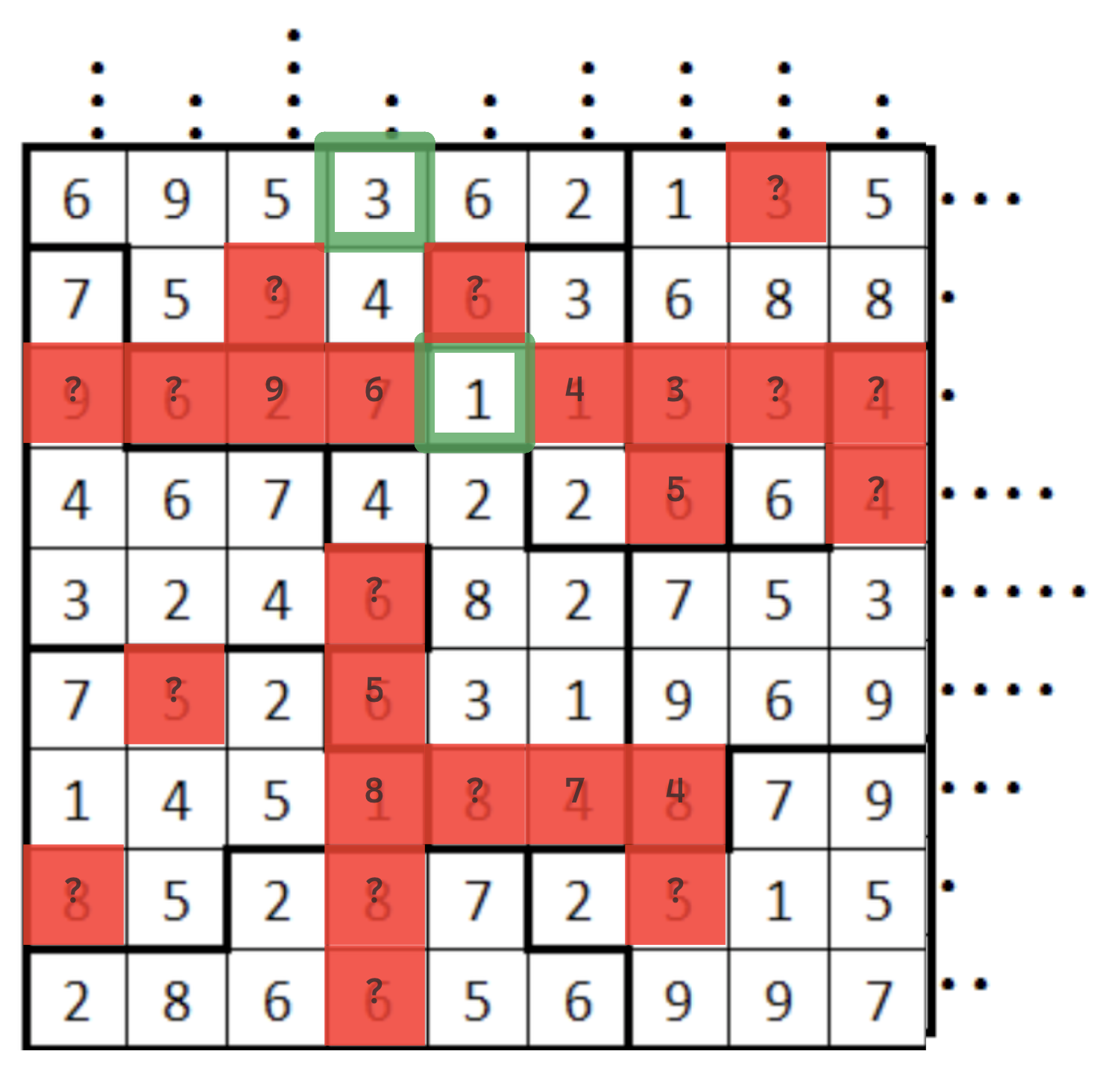
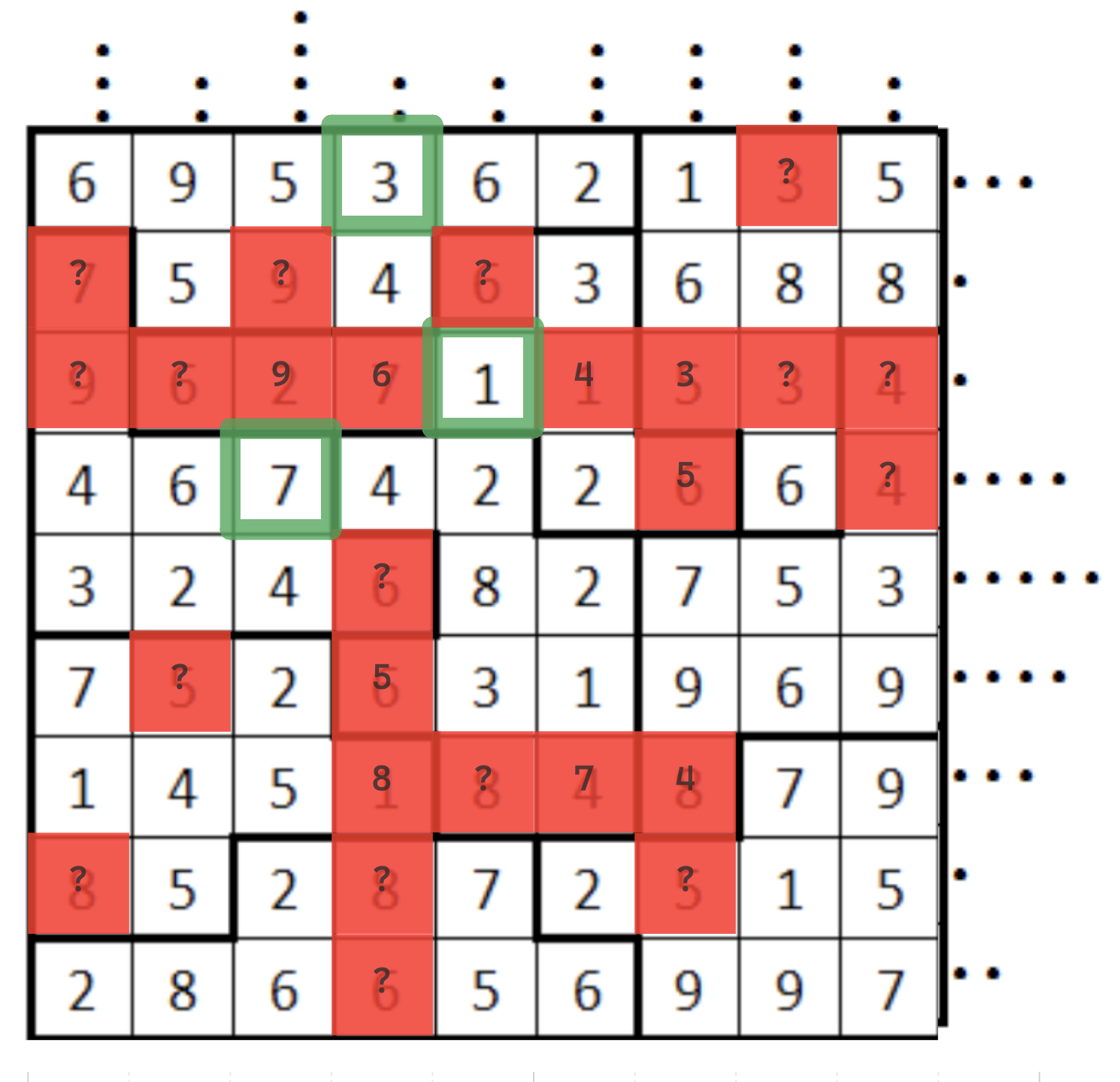
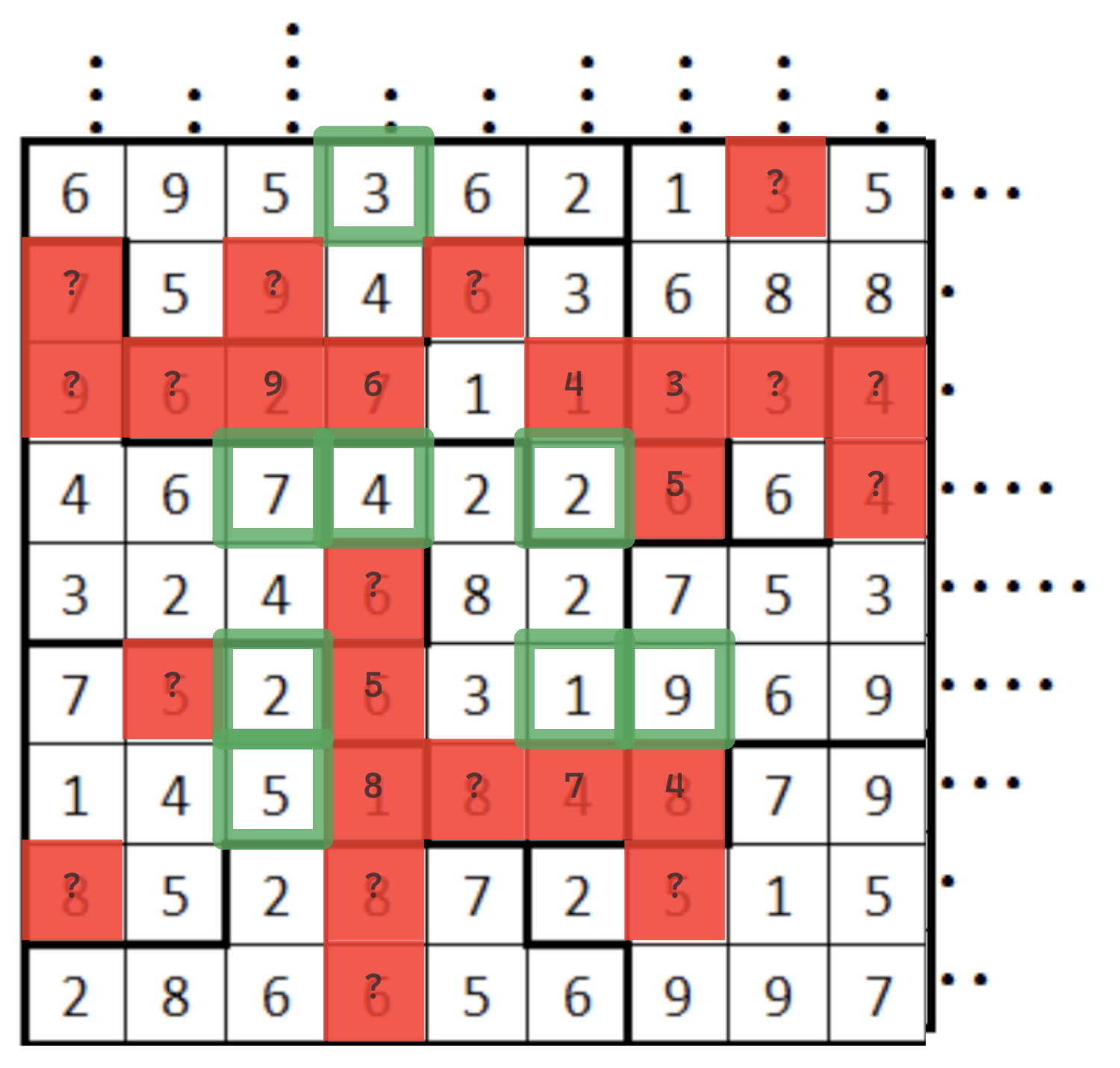
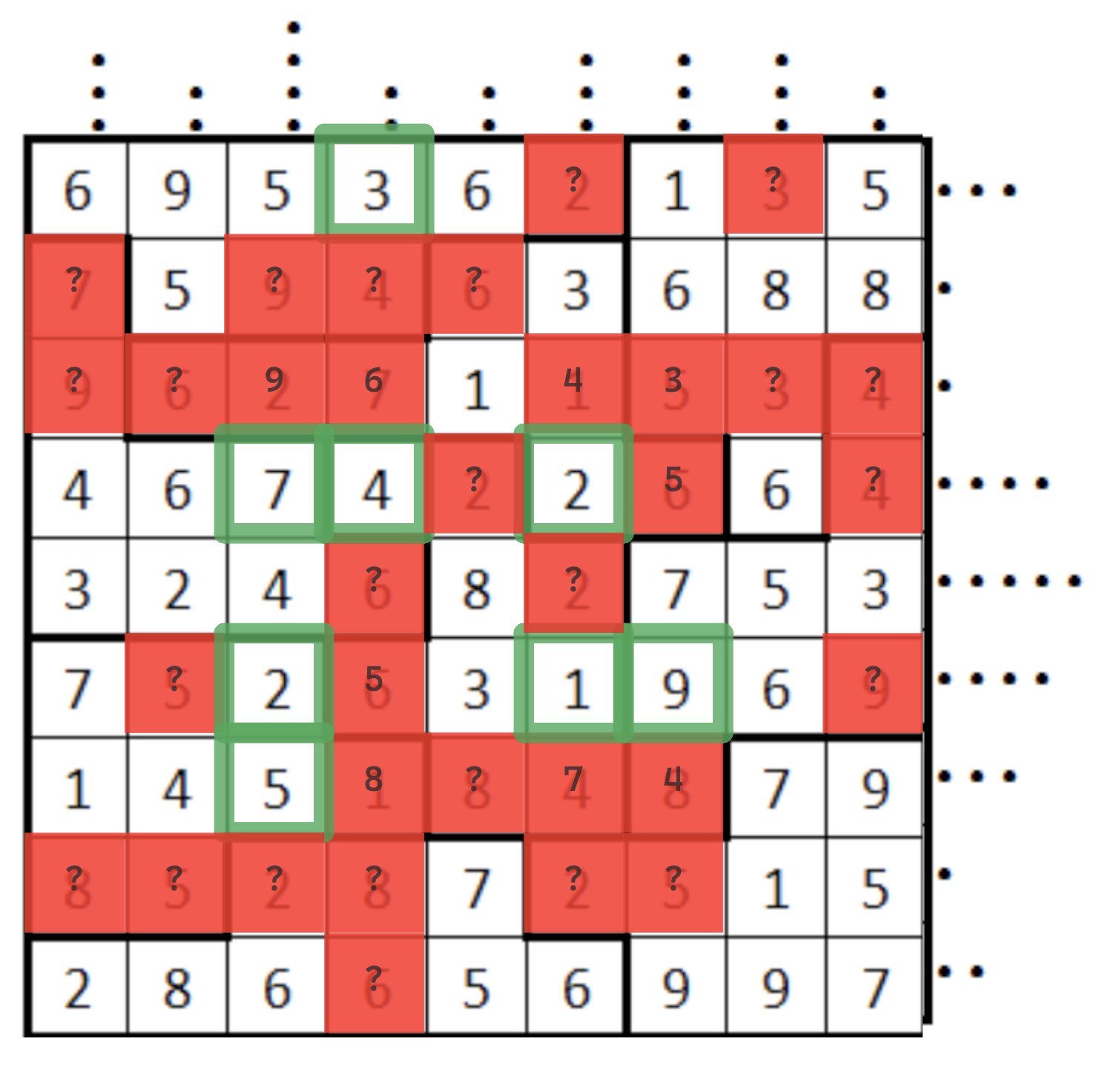
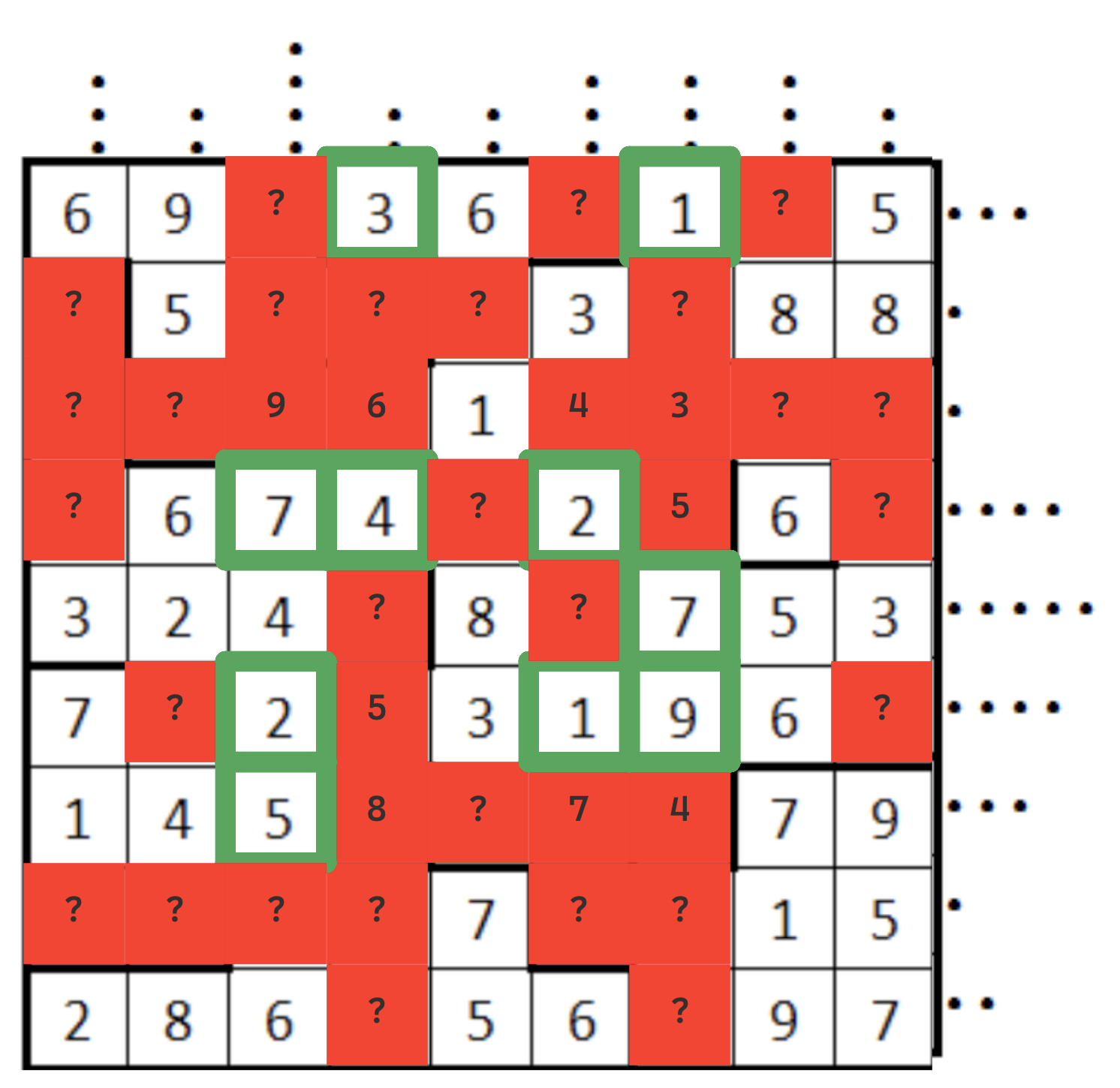
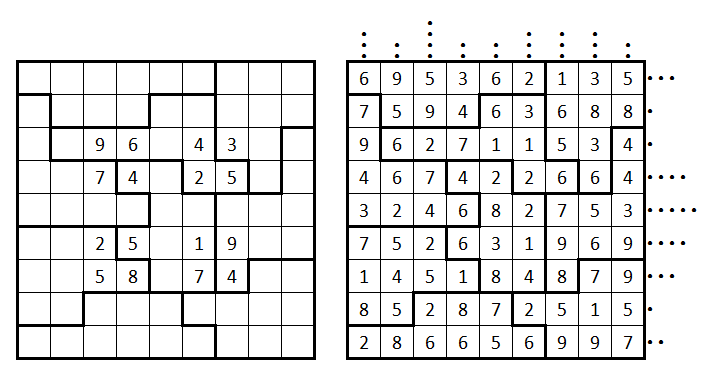
This is a Colombian Jigsaw Sudoku where the usual rules apply, but with irregular boxes. The dots in the right-hand scheme indicate how many of the numbers in a particular row or column are correctly placed in the corresponding row or column of the solved Sudoku on the left.
-
1$\begingroup$ Numbers? Don't you mean dots? $\endgroup$– BassCommented Jan 31 at 17:13
-
$\begingroup$ Also, is there a particular reason for having a different box layout for the right-hand side grid? It feels a bit annoying that I have to copy the actual box boundaries over myself in order to apply box login on that side. $\endgroup$– BassCommented Jan 31 at 17:28
-
$\begingroup$ Isn't the grid on the left commonly called a "Jigsaw Sudoku"? It's not "traditional Sudoku". $\endgroup$– Weather VaneCommented Jan 31 at 19:23
-
$\begingroup$ Is the puzzle solvable without the dot hints? What does "with four fours" mean? The grid given has 3 fours and the one on the right has 8, of course 9 are always needed. There are 3 hints with four dots. $\endgroup$– Weather VaneCommented Jan 31 at 19:34
-
2$\begingroup$ Dude, this is diabolical! I thought, "oh, cool, a sudoku nobody's solved yet! I can do that." ... It's been like 4 hours! $\endgroup$– StevishCommented Feb 2 at 21:20
2 Answers
Givens are indicated with <> and derived digits with **
We initially inspect all the second board and mark digit in cells with guaranteed mismatch with an x. We also mark digits in cells that match with parenthesis. The mismatches and matches are due to the givens. Derived matchings are indicated in the second board with [ ]. Derived mismatches are indicated with y. MC denotes matching conditions, NP denotes naked pair, T denotes Naked triple and HS denotes hidden single. Finally, | means OR.
.
. . . . .
. . . . . . . .
. . . ok . . . . .
.-----------------------------------. .-----------------------------------.
9 | *3* | | | 6 9 5x [3] 6 2x| 1 3x 5 | ...
|---+ +---+---+ | |---+ +---+---+ |
8 | | | | | | 7x| 5 9x 4x| 6x 3 | 6 8 8 | .
| |---+---+---+ | +---| | |---+---+---+ | +---|
7 | | <9> <6> *1 <4>|<3> | | | 9x| 6x 2x 7x [1] 1x| 5x 3x| 4x| .
| +---+---+---+---+ +---+ + | | +---+---+---+---+ +---+ + |
6 | <7>|<4> |<2> <5>| | | | 4x 6 (7)|(4) 2x|(2) 6x| 6 | 4x| ....
| +---+ +---+---+---+ | | +---+ +---+---+---+ |
5 | | | | | 3 2 4 6x| 8 2x| 7 5 3 | .....
|---+---+---+---+ | | |---+---+---+---+ | |
4 | <2>|<5> <1>|<9> | | 7 5x (2)| 6x 3 (1)|(9) 6 9x| ....
| +---+ | +---+---| | +---+ | +---+---|
3 |*1* <5> <8>| <7>|<4>| *9*| |[1] 4x (5) 1x| 8x 4x| 8x| 7x [9]| ...
| +---+---+---+---+---+ | | +---+---+---+---+---+ |
2 | | | | | 8x 5x| 2x 8x 7 | 2x 5x 1 5 | .
|---+---+ +---+ | |---+---+ +---+ |
1 | | | | 2 8 6 6x 5 6 | 9x 9y 7 | ..
'-----------------------------------' '-----------------------------------'
a b c d e f g h i a b c d e f g h i
STEP 1: e7 = 1 due to Row 7 of the second board, we have already 8 mismatches and need exactly one match.
STEP 2: Column d has 7 mismatches and only 1 match and we need 1 more match. So, it has to be d9=3.
STEP 3: Row 3 has 1 matching and 6 mismatches. Since we need a total of 3 matches, we must have a3=1, i3=9.
Due to <7>c6 and <7>f3, we infer that (7) must be true at one of the cells of ghi5.
. We cannot have (7)h5, because then g5,h5,d5,f5 would be mismatching cells and since (3)a5,(3)i5 in the second board imply that an additional mismatching cell exists at that row, this would give a total of 5 mismatches in that Row [impossible due to MC]. So, h5 cannot be 7.
. (7)i5 is also not possible, because the resulting mismatching cells d5,f5,g5,i5 are the only possible in Row 5, and so all other cells in that Row would be matching cells [(3)a5,(2)b5,(4)c5,(8)e5,(5)h5]. In this situation, (5)h5 and <5>g5 imply (by Jigwaw sudoku rules) that (5) must be true at i8 or i9. In Column i we have the mismatching cells: i1 (due to (7)i5),i2(due to (5)i89),i4,i5,i6,i7, that is, 6 mismatching cells. We need two matching cells and we have already the matching cell i3, so we must have (5)i9. Using Jigsaw Sudoku rules, we must have (5)b8 [due to (<5>)c3,(<5>)d4,(5)i9] and (5)a7 [HS]. Not having match at g5 implies (due to MC Column g), that (6)g8,(1)g9 would be true. By Jigsaw Sudoku rules, (6)h6 is false, and so (6)b6 must be true [MC at Row 6]. But (<1>)f4,(1)g9, (6)f5 [HS] and Jigsaw rules imply that (1)d5 and (6)f5 are HS in Row 5. However (see picture below)
.-----------------------------------.
9 | *3* |*1* *5*|
|---+ +---+---+ |
8 | |*5* | |*6* |
| |---+---+---+ | +---|
7 |*5*| <9> <6> *1 <4>|<3> | |
| +---+---+---+---+ +---+ + |
6 | *6* <7>|<4> |<2> <5>| | |
| +---+ +---+---+---+ |
5 |*3* *2* *4* *1*|*8* *6*| *5* *7*|
|---+---+---+---+ | |
4 | <2>|<5> <1>|<9> |
| +---+ | +---+---|
3 |*1* <5> <8>| <7>|<4>| *9*|
| +---+---+---+---+---+ |
2 | | | |
|---+---+ +---+ |
1 | | |
'-----------------------------------'
a b c d e f g h i
that would imply, in order to complete Row 5 that (9)g5. This is impossible due to (9)g4. So we cannot have (7)i5.
The conclusion is that we must have g5=7.
STEP 4: We write candidates at some cells of board one.
.
. . . . .
. . . . . . . .
. . . ok . . . . .
.--------------------------------------------. .-----------------------------------.
9 | *3* |1268 | | 6 9 5x [3] 6 2x| 1 3x 5 | ...
|-----+ +----+----+ | |---+ +---+---+ |
8 | | |378 38 |1268 | | 7x| 5 9x 4x| 6x 3 | 6 8 8 | .
| |-----+---+---+ | +----| | |---+---+---+ | +---|
7 |2578 |78 <9> <6> *1 <4> |<3> 2578|258 | | 9x| 6x 2x 7x [1] 1x| 5x 3x| 4x| ok
| +-----+---+---+--- + +----+ + | | +---+---+---+---+ +---+ + |
6 | <7>|<4> 3689|<2> <5> |1689|1368| | 4x 6 (7)|(4) 2x|(2) 6x| 6 | 4x| ....
| +---+ +----+----+----+ | | +---+ +---+---+---+ |
5 | 129| 3689|*7* | | 3 2 4 6x| 8 2x|[7] 5 3 | .....
|-----+-----+---+---+ | | |---+---+---+---+ | |
4 |3467 3467 <2>|<5> 368 <1> |<9> 368 368 | | 7 5x (2)| 6x 3 (1)|(9) 6 9x| ....
| +---+ | +----+----| | +---+ | +---+---|
3 |*1* 36 <5> <8>|236 <7> |<4> |236 *9* | |[1] 4x (5) 1x| 8x 4x| 8x| 7x [9]| ok
| +---+---+----+----+----+ | | +---+---+---+---+---+ |
2 |34679 34679| |3568 1268 | | 8x 5x| 2x 8x 7 | 2x 5x 1 5 | .
|-----+-----+ +----+ | |---+---+ +---+ |
1 | |1268 | | 2 8 6 6x 5 6 | 9x 9y 7 | ..
'--------------------------------------------' '-----------------------------------'
a b c d e f g h i a b c d e f g h i
NT (368)ehi4 => -36 ab4
NP (47)ab4 => -47 ab2
Locked candidates (9)ab2 => -9 d2, -9 e2
.
. . . . .
. . . . . . . .
. . . ok . . . . .
.---------------------------------------------. .-----------------------------------.
9 | *3* 5689|1268 | | 6 9 5x [3] 6 2x| 1 3x 5 | ...
|-----+ +----+----+ | |---+ +---+---+ |
8 | | 1279|378 38 |1268 | | 7x| 5 9x 4x| 6x 3 | 6 8 8 | .
| |-----+---+----+ | +----| | |---+---+---+ | +---|
7 |258 |78 <9> <6> *1 <4> |<3> 2578|258 | | 9x| 6x 2x 7x [1] 1x| 5x 3x| 4x| ok
| +-----+---+----+--- + +----+ + | | +---+---+---+---+ +---+ + |
6 | <7>|<4> 3689|<2> <5> |1689|1368| | 4x 6 (7)|(4) 2x|(2) 6x| 6 | 4x| ....
| +----+ +----+----+----+ | | +---+ +---+---+---+ |
5 | 129 | 3689|*7* | | 3 2 4 6x| 8 2x|[7] 5 3 | .....
|-----+-----+---+----+ | | |---+---+---+---+ | |
4 | 47 47 <2>|<5> 368 <1> |<9> 368 368 | | 7 5x (2)| 6x 3 (1)|(9) 6 9x| ....
| +----+ | +----+----| | +---+ | +---+---|
3 |*1* 36 <5> <8> |236 <7> |<4> |236 *9* | |[1] 4x (5) 1x| 8x 4x| 8x| 7x [9]| ok
| +---+----+----+----+----+ | | +---+---+---+---+---+ |
2 |369 369 | 127 |3568 1268 | | 8x 5x| 2x 8x 7 | 2x 5x 1 5 | .
|-----+-----+ +----+ | |---+---+ +---+ |
1 | 1279 |1268 | | 2 8 6 6x 5 6 | 9x 9y 7 | ..
'---------------------------------------------' '-----------------------------------'
a b c d e f g h i a b c d e f g h i
. Looking at matching conditions as Column g: only one of (6)g8,(1)g9 must be true.
. Looking at matching conditions as Row 6: only one of (6)h6,(6)b6 must be true, so:
(6)g8--(6)h6 false--> (6)b6 [MC Row 6],(8)f8[to avoid two matches at Row 8] -> (3)b3,(7)b7,(3)e8,(4)b4,(7)a4[matching],(9)b2,(6)a2 Then, (6)a2,(9)b2 and MC at Row 9 imply that (6)e9,(5)i9 [(1)g9 would be false due to (6)g8 and MC Column g]. As before, (5)i9 => (5)b8 [HS], that contradicts having only one match at Row 8. So, we cannot have (6)g8 and g9=1 [and (6)g8 is false => Bivalue cell (28)g8].
=> Locked Candidate (6)g12 => - 6 f2, -6 h3
STEP 5:
.
. . . .
. . . . . . .
. . . ok . . ok . .
.-----------------------------------------------. .-----------------------------------.
9 | *3* 5689|*1* | | 6 9 5x [3] 6 2x| [1] 3x 5 | ...
|-----+ +----+----+ | |---+ +---+---+ |
8 | | 1468 1279|378 38 | 28 | | 7x| 5 9x 4x| 6x 3 | 6y 8 8 | .
| |-----+-----+----+ | +----| | |---+---+---+ | +---|
7 |258 |78 <9> <6> *1* <4> |<3> 2578|258 | | 9x| 6x 2x 7x [1] 1x| 5x 3x| 4x| ok
| +-----+-----+----+--- + +----+ + | | +---+---+---+---+ +---+ + |
6 | <7> |<4> 3689|<2> <5> | 689|1368| | 4x 6 (7)|(4) 2x|(2) 6x| 6 | 4x| ....
| +----+ +----+----+----+ | | +---+ +---+---+---+ |
5 | 129 | 3689|*7* | | 3 2 4 6x| 8 2x|[7] 5 3 | .....
|-----+-----+-----+----+ | | |---+---+---+---+ | |
4 | 47 47 <2> |<5> 368 <1> |<9> 368 368 | | 7 5x (2)| 6x 3 (1)|(9) 6 9x| ....
| +----+ | +----+----| | +---+ | +---+---|
3 |*1* 36 <5> <8> |236 <7> |<4> |23 *9* | |[1] 4x (5) 1x| 8x 4x| 8x| 7x [9]| ok
| +-----+----+----+----+----+ | | +---+---+---+---+---+ |
2 |369 369 | 127 |358 268 | | 8x 5x| 2x 8x 7 | 2x 5x 1 5 | .
|-----+-----+ +----+ | |---+---+ +---+ |
1 | 1279 | 268 | | 2 8 6 6x 5 6 | 9x 9y 7 | ..
'-----------------------------------------------' '-----------------------------------'
a b c d e f g h i a b c d e f g h i
Now, (6)b6 -> (3)b3,(2)h3,(6)e3 [=> (6)e9 false],(9)b2, and (6)a2 =matching condition Row 9=> (5)i9 -> (5)b8,(5)h5 [(2)b5 false due to MC at Column b, and (3)i5 false due to MC at Column i]. Thus in Row 5 we must have (3)a5,(4)c4,(8)e5,(5)h5 to fulfill the MC. (5)b8 and the MC Row 8 imply that (8)f8 must be true, so (7)b7 too and (7)a4 too. But now the MC Column h cannot be satisfied [due to MC Row 4, (6)h4 is false; (6)h6 is false due to (6)b6, and (8)g8 too]. We conclude that h6=6 (next figure).
STEP 6:
.-----------------------------------------------.
9 | *3* 5689| *1* |
|-----+ +----+----+ |
8 | | 1468 1279|378 38 | 28 |
| |-----+-----+----+ | +----|
7 |258 |78 <9> <6> *1* <4> |<3> 2578|258 |
| +-----+-----+----+--- + +----+ + |
6 |389 1389 <7> |<4> 389 |<2> <5> |*6* |138 |
| +----+ +----+----+----+ |
5 | 129 | 3689|*7* 12358 |
|-----+-----+-----+----+ | |
4 | 47 47 <2> |<5> 368 <1> |<9> 38 368 |
| +----+ | +----+----|
3 |*1* 36 <5> <8> |236 <7> |<4> |23 *9* |
| +-----+----+----+----+----+ |
2 |369 369 | 127 |358 268 |
|-----+-----+ +----+ |
1 | 1279 |268 |
'-----------------------------------------------'
a b c d e f g h i
We cannot have h8=8 (or i8=8), because that imply f8=3 (Bivalue Cell), violating the MC at Row 8.
So, in Column h the remaining 2 needed matches are forced: h5=5 [taking out 5)h7,(5)i7], h2=1.
Also, since the matching at Row 2 is fulfilled, (7)e2 and (5)i2 are false.
Now in Row 7, we have only one place for number 5: a7=5.
STEP 7: We must have 5 at i8 or i9 due (5)h5, (<5>)g6.
Suppose (5)i9 is true (figure below). Then (5)b8 [HS and match] => f8=8 [MC Row 8].
.
. . .
. . . . .
. . . ok . . ok ok ok
.-------------------------------------------------. .-----------------------------------.
9 | 468 *3* 69 |*1* 24789 *5* | | 6 9 5x [3] 6 2x| [1] 3x [5]| ...
|-----+ +----+----+ | |---+ +---+---+ |
8 | |*5* 146 1279|37 *8* | 28 | | 7x|[5] 9x 4x| 6x 3y| 6y 8y 8y| ok
| |-----+-----+----+ | +----| | |---+---+---+ | +---|
7 |*5* |78 <9> <6> *1* <4> |<3> 278 |28 | | 9x| 6x 2x 7x [1] 1x| 5x 3x| 4x| ok
| +-----+-----+----+--- + +----+ + | | +---+---+---+---+ +---+ + |
6 |389 1389 <7> |<4> 389 |<2> <5> |*6* |138 | | 4x 6y (7)|(4) 2x|(2) 6x|[6]| 4x| ok
| +----+ +----+----+------+ | | +---+ +---+---+---+ |
5 | 13468 129 | 369 |*7* *5* | | 3 2 4 6x| 8 2x|[7] [5] 3 | .....
|-----+-----+-----+----+ | | |---+---+---+---+ | |
4 | 47 47 <2> |<5> 368 <1> |<9> 38 368 | | 7 5x (2)| 6x 3 (1)|(9) 6y 9x| ....
| +----+ | +------+----| | +---+ | +---+---|
3 |*1* 36 <5> <8> |236 <7> |<4> |23 *9* | |[1] 4x (5) 1x| 8x 4x| 8x| 7x [9]| ok
| +-----+----+----+----+----+ | | +---+---+---+---+---+ |
2 |369 369 | 27 |35 268 *1* | | 8x 5x| 2x 8x 7y| 2x 5x [1] 5y| ok
|-----+-----+ +----+ | |---+---+ +---+ |
1 | 1279 |268 | | 2 8 6 6x 5 6 | 9x 9y 7 | ..
'-------------------------------------------------' '-----------------------------------'
a b c d e f g h i a b c d e f g h i
We have now some singles:
g8=2, b7=7, h7=8, f2=5[HS], h4=3, h3=2, i7=2, (5)e1 [HS and match].
This implies that (8)e5 is false [MC Column 3], (6)e9 is false.
Also, NP(68)g12 takes out (68)i12, and
b4=4 [HS], a4=7 [match cell]. We must have (6)f1 [MC Column f], and so (6)c1 is false.
Due to MC at Column c, c5=4. We have a full house e8=3 => e3=6, b3=3,
(8)g2 [due to NP(69)ab2] => (6)g1.
.
. .
. . ok ok ok ok ok ok ok
.----------------------------------------------------. .-----------------------------------.
9 |24689 2689 468 *3* 24789 69 |*1* 479 *5* | | 6y 9y 5x [3] 6y 2x| [1] 3x [5]| ok
|-----+ +------+----+ | |---+ +---+---+ |
8 | 469 |*5* 1468 1279|*3* *8* |*2* 479 47 | | 7x|[5] 9x 4x| 6x 3y| 6y 8y 8y| ok
| |------+-----+----+ | +----| | |---+---+---+ | +---|
7 | *5* |*7* <9> <6> *1* <4> |<3> *8* |*2* | | 9x| 6x 2x 7x [1] 1x| 5x 3x| 4x| ok
| +------+-----+----+------+ +----+ + | | +---+---+---+---+ +---+ + |
6 | 389 1389 <7> |<4> 89 |<2> <5> |*6* | 18 | | 4x 6y (7)|(4) 2x|(2) 6x|[6]| 4x| ok
| +----+ +----+----+------+ | | +---+ +---+---+---+ |
5 |23689 123689 *4* 129 |269 369 |*7* *5* 168 | | 3 2 [4] 6x| 8y 2x|[7] [5] 3y| .....
|-----+------+-----+----+ | | |---+---+---+---+ | |
4 | *7* *4* <2> |<5> 68 <1> |<9> *3* 68 | |[7] 5x (2)| 6x 3y (1)|(9) 6y 9x| ok
| +----+ | +------+----| | +---+ | +---+---|
3 | *1* *3* <5> <8> |*6* <7> |<4> |*2* *9* | |[1] 4x (5) 1x| 8x 4x| 8x| 7x [9]| ok
| +-----+----+------+----+----+ | | +---+---+---+---+---+ |
2 | 69 69 |346 27 247 |*5* *8* *1* 347 | | 8x 5x| 2x 8x 7y| 2x 5x [1] 5y| ok
|-----+------+ +----+ | |---+---+ +---+ |
1 |23489 1289 138 1279 *5* 39 |*6* 47 347 | | 2y 8y 6y 6x [5] [6]| 9x 9y 7 | ok
'---------------------------------------------------' '-----------------------------------'
a b c d e f g h i a b c d e f g h i
Due to MC Row 5, we must have a5=3, b5=2.
We reached a contradiction, since 3 is not true at any cell of Row 6.
Conclusion: i8=5.
STEP 8:
.-------------------------------------------------.
9 | 468 *3* 5689|*1* 24789 |
|-----+ +----+----+ |
8 | | 1468 1279|378 38 | 28 *5* |
| |-----+-----+----+ | +----|
7 |*5* |78 <9> <6> *1* <4> |<3> 278 |28 |
| +-----+-----+----+--- + +----+ + |
6 |389 1389 <7> |<4> 389 |<2> <5> |*6* |138 |
| +----+ +----+----+------+ |
5 | 13468 129 | 3689|*7* *5* |
|-----+-----+-----+----+ | |
4 | 47 47 <2> |<5> 368 <1> |<9> 38 368 |
| +----+ | +------+----|
3 |*1* 36 <5> <8> |236 <7> |<4> |23 *9* |
| +-----+----+----+----+----+ |
2 |369 369 | 27 |358 268 *1* |
|-----+-----+ +----+ |
1 | 1279 |268 |
'-------------------------------------------------'
a b c d e f g h i
Since b8 cannot be 5, we must have f8=3 [MC Row 8].
We also have f2=5 (HS).
If (8)i7 then (2)h7 -> (8)g8 -> (3)f8,(7)e8 -> (8)b7 [contradiction: two 8s in the same Row]
So, i7=2.
Locked candidates (2)e35 imply that e2 is not 2.
.---------------------------------------------------.
9 | 468 *3* 689|*1* 24789 478 |
|-----+ +-----+----+ |
8 | | 1468 1279|78 *3* | 28 2479 *5* |
| |-----+-----+----+ | +-----|
7 |*5* |78 <9> <6> *1* <4> |<3> 78 |*2* |
| +-----+-----+----+-----+ +----+ + |
6 |389 1389 <7> |<4> 389 |<2> <5> |*6* |138 |
| +----+ +----+----+------+ |
5 | 13468 129 |23689 689 |*7* *5* 1368 |
|-----+-----+-----+----+ | |
4 | 47 47 <2> |<5> 368 <1> |<9> 38 368 |
| +----+ | +------+-----|
3 |*1* 36 <5> <8> |236 <7> |<4> | 23 *9* |
| +-----+----+-----+----+----+ |
2 |369 369 |3468 27 3468 |*5* 268 *1* 3478|
|-----+-----+ +----+ |
1 | 13468 1279 89 |268 23478 3478|
'---------------------------------------------------'
a b c d e f g h i
Let us investigate the consequences of each digit of e5 being true:
. (2)e5 -> b5 is not 2, so we have 4 mismatches in Row 5, forcing a5=3,c5=4,i5=3 [impossible]
. (3)e5|(6)e5|(9)e5 -> e5 not 2 -> (2)e3 [2 locked at e35] -> (3)h3 -> (8)h4 -> (7)h7,(8)b7,(7)e8
. (8)e5 -> (7)e8, (8)b7,(7)h7
So, in all cases we can assert that e8=7, h7=7, b8=7.
STEP 9:
.---------------------------------------------------.
9 | 468 *3* 689|*1* 2489 48 |
|-----+ +-----+----+ |
8 | | 1468 129 |*7* *3* | 28 249 *5* |
| |-----+-----+----+ | +-----|
7 |*5* |*8* <9> <6> *1* <4> |<3> *7* |*2* |
| +-----+-----+----+-----+ +----+ + |
6 |389 139 <7> |<4> 389 |<2> <5> |*6* |138 |
| +----+ +----+----+------+ |
5 | 13468 129 |23689 689 |*7* *5* 1368 |
|-----+-----+-----+----+ | |
4 | 47 47 <2> |<5> 368 <1> |<9> 38 368 |
| +----+ | +------+-----|
3 |*1* 36 <5> <8> |236 <7> |<4> | 23 *9* |
| +-----+----+-----+----+----+ |
2 |369 369 |3468 27 3468 |*5* 268 *1* 3478|
|-----+-----+ +----+ |
1 | 13468 1279 89 |268 2348 3478|
'---------------------------------------------------'
a b c d e f g h i
Now, due to MC at Column b, we must have b9=9, b5=2. We have a2=9 [HS], b6=1 [due to NP(36)b23]. We have singles: e6=9, i5=1 [so, mismatch at i5], d5=9, e9=5 [so mismatch at e1,e9], b1=5.
Due to MC Column i, we must have i1=7 [and d2=7, g2=2,h3=3,b3=6,b2=3,g8=8,g1=6 are HS].
Due to g1=6, we infer that (6)c1 is false, so c5=4 [MC Column c] and a1=2 [MC Row 1].
More singles: h4=8, i6=3, a6=8, i4=6, e4=3 [match] => (7)i4 is false => a4=4, b4=7, b8=4.
Now, i5=3 [MC at Column 1; completes MC in Row 5 too].
ok ok ok ok ok ok ok ok ok
.---------------------------------------------------. .-----------------------------------.
9 | *9* 68 *3* *5* 68 |*1* 24 48 | | 6y [9] 5x [3] 6y 2x| [1] 3x 5y| ok
|-----+ +-----+----+ | |---+ +---+---+ |
8 | |*4* 16 12 |*7* *3* |*8* 249 *5* | | 7x| 5y 9x 4x| 6x [3]| 6y 8y 8y| ok
| |-----+-----+----+ | +-----| | |---+---+---+ | +---|
7 |*5* |*8* <9> <6> *1* <4> |<3> *7* |*2* | | 9x| 6x 2x 7x [1] 1x| 5x 3x| 4x| ok
| +-----+-----+----+-----+ +----+ + | | +---+---+---+---+ +---+ + |
6 |*8* *1* <7> |<4> *9* |<2> <5> |*6* |*3* | | 4x 6y (7)|(4) 2x|(2) 6x|[6]| 4x| ok
| +----+ +----+----+------+ | | +---+ +---+---+---+ |
5 |*3* *2* *4* *9* |23689 689 |*7* *5* *1* | |[3] [2] [4] 6x| 8y 2x|[7] [5] 3y| ok
|-----+-----+-----+----+ | | |---+---+---+---+ | |
4 |*4* *7* <2> |<5> *3* <1> |<9> *8* *6* | | 7y 5x (2)| 6x [3] (1)|(9) 6y 9x| ok
| +----+ | +------+-----| | +---+ | +---+---|
3 |*1* *6* <5> <8> |236 <7> |<4> |*3* *9* | |[1] 4x (5) 1x| 8x 4x| 8x| 7x [9]| ok
| +-----+----+-----+----+----+ | | +---+---+---+---+---+ |
2 |*9* *3* | 68 *7* 468 |*5* *2* *1* 48 | | 8x 5x| 2x 8x 7y| 2x 5x [1] 5y| ok
|-----+-----+ +----+ | |---+---+ +---+ |
1 |*2* *5* 138 1279 89 |*6* 2348 *7* | |[2] 8y 6y 6x 5y 6y| 9x 9y [7]| ok
'---------------------------------------------------' '-----------------------------------'
a b c d e f g h i a b c d e f g h i
All matching conditions are satisfied.
FINAL STEP: Only singles remain
a9=7, a8=6, c8=1, d8=2, d1=3, h9=2, h1=4, h8=9, i2=8, i9=4,
c2=6, e2=4, d1=1 e1=8, f1=9, e5=6, f5=8, f9=6, c9=8 and e3=2.
The puzzle is solved. I enjoyed solving it. Thanks the OP for the puzzle (my first Jigsaw).
.---------------------------------------------------.
9 |*7* *9* *8* *3* *5* *6* |*1* *2* *4* |
|-----+ +-----+----+ |
8 |*6* |*4* *1* *2* |*7* *3* |*8* *9* *5* |
| |-----+-----+----+ | +-----|
7 |*5* |*8* <9> <6> *1* <4> |<3> *7* |*2* |
| +-----+-----+----+-----+ +----+ + |
6 |*8* *1* <7> |<4> *9* |<2> <5> |*6* |*3* |
| +----+ +----+----+------+ |
5 |*3* *2* *4* *9* |*6* *8* |*7* *5* *1* |
|-----+-----+-----+----+ | |
4 |*4* *7* <2> |<5> *3* <1> |<9> *8* *6* |
| +----+ | +------+-----|
3 |*1* *6* <5> <8> |*2* <7> |<4> |*3* *9* |
| +-----+----+-----+----+----+ |
2 |*9* *3* |*6* *7* *4* |*5* *2* *1* *8* |
|-----+-----+ +----+ |
1 |*2* *5* *3* *1* *8* *9* |*6* *4* *7* |
'---------------------------------------------------'
a b c d e f g h i
-
$\begingroup$ Can you write your answer in the orderly form? I don't understand the procedure very well $\endgroup$ Commented Feb 5 at 18:47
-
$\begingroup$ @Xavier Castillo I had trouble editing [new user] and had to cut the second board in many places to fit the requirement of 30000 characters [I am a bit under this]. I solve in a txt file with the two boards side by side, so that I can make use of the procedure described at the beginning. It is my way of solving with pencil and paper electronically. Please explain what you mean by orderly form. I have posted almost everything but some second boards (the ones with dots) that I had to cut. $\endgroup$– JCOCommented Feb 5 at 19:35
-
$\begingroup$ The boards have columns labels "a" to "i" from left to write and columns "1" to "9" from bottom to top ['chess notation"]. The two boards are updated during the solution procedure. Every time a new matching cell is found, I place a [ ] in the right board and the derived digit in the left-board is written between **. In no-matching cases, I label the cell with an y on the right board and place the digit between ** in the left board. The posted solution is the exact sequence that I followed while solving the puzzle. $\endgroup$– JCOCommented Feb 5 at 19:45
-
$\begingroup$ Regarding notation, for instance (6)b6 means digit 6 in cell b6 [column b, row 6]. $\endgroup$– JCOCommented Feb 5 at 19:54
-
1$\begingroup$ @JCO Excellent job! Hardest Colombian Sudoku on earth! $\endgroup$ Commented Feb 6 at 0:35
Partial for now
We start with
(R1C4 must be correct since C4 is 3,4,4)
Then we can apply the same logic to get that
R4C3 is correct in R4, so
Marking correct cells yields
Obvious deductions have that
Then