UPDATED WITH A COMPLETE REWRITE
A minimum velocity does
not exist.
First observe the hard lower bound
g > 3
This is straightforward to establish:
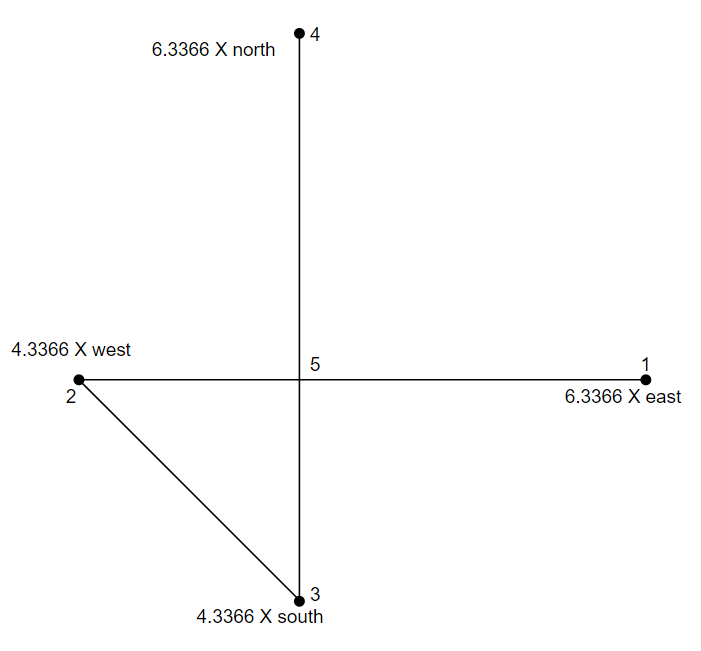
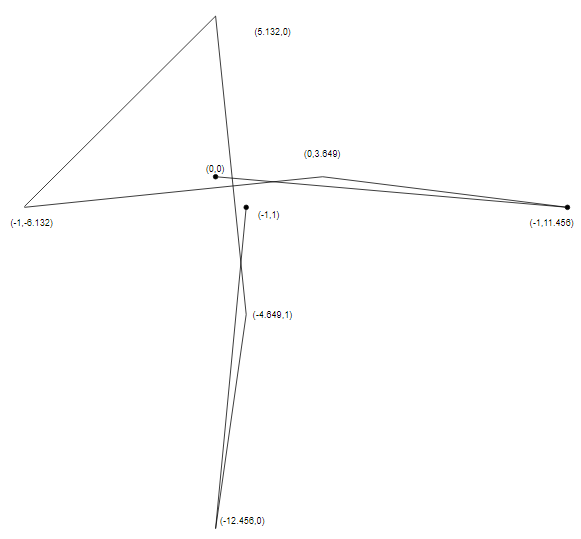
Consider the velocity vectors of the freely moving star. These are constant unit vectors representing the four principal winds. Now take scalar product of each with the corresponding star's position vector. For each star that is not in the immediate care of the guardian this product will grow at a rate of 1. Now consider the sum of these scalar products. When all stars meet its value is zero. In the initial configuration it is four, so to make the stars meet it must be shrunk. As at each time at least three stars contribute growth at rate 1 each the guardian can not compensate unless its speed is strictly above 3.
I will now show simulations of a strategy that strongly suggest that
any g > 3 is actually good enough:
The strategy has to phases:
Phase 1:
Cycle through the stars E->N->W->S->E->... always using the quickest intercept course to reach the next and always carry the just intercepted star all the way to the next (so we never travel empty).
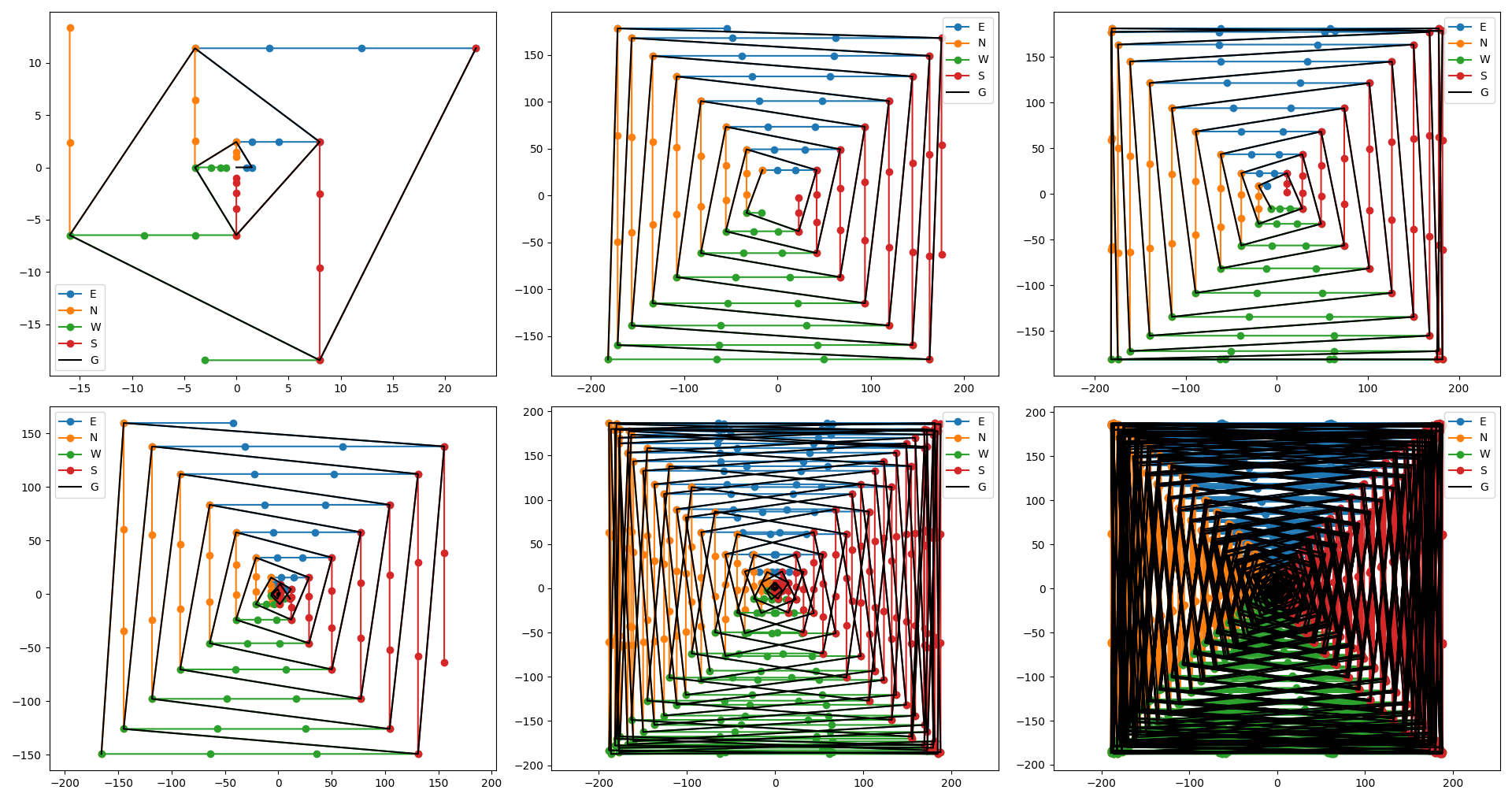
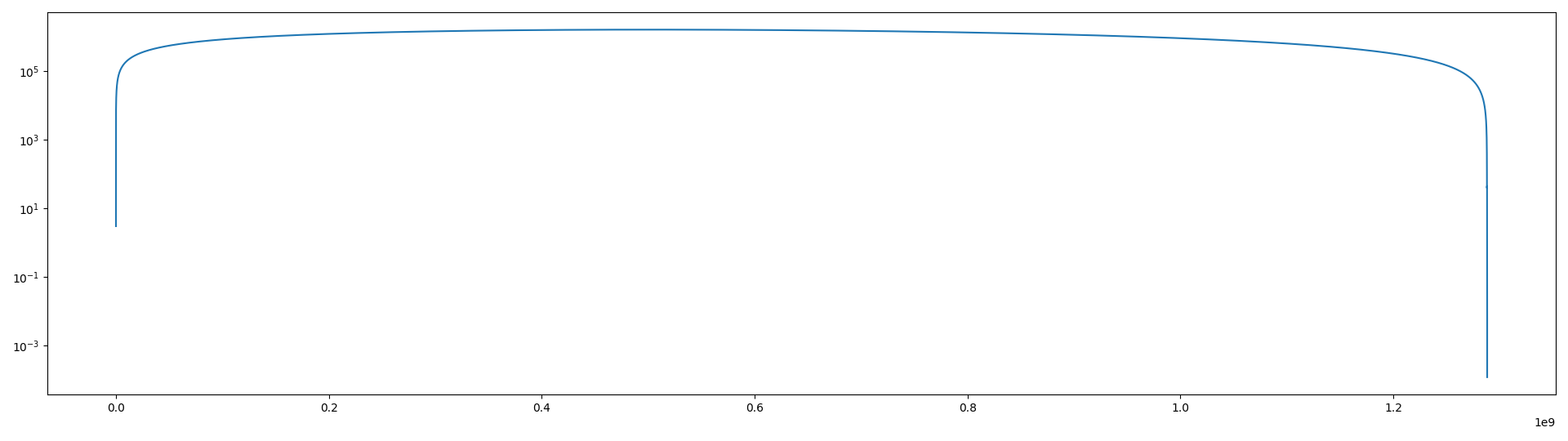
This is a simulation with g about 1% above 3. Values much closer to 3 give qualitatively the same picture.
Panel 1: At first the configuration appears to expand rather quickly.
Panel 2: But a spiralling pattern is quickly establishied with the rate of expansion shrinking over time ...
Panel 3: ... until it is actually reversed resulting in a collapsing spiral.
Panel 4: This, however, is reversed again at some point leading to renewd growth.
Panels 5 and 6: The trend keeps reversing resulting in perpetual oscillation.
Phase 2:
The second part of the strategy breaks this yoyo behaviour the instant the system first goes into shrinkage by tempering its rate. More precisely, as soon as the guardians steps are detected to reduce the lateral (wrt its current cargo's free velocity vector) displacement we reduce the reduction by 50% (smaller values resulting in faster convergence are also possible,see below for an example). In simulations this reliably prevented the next reversal.

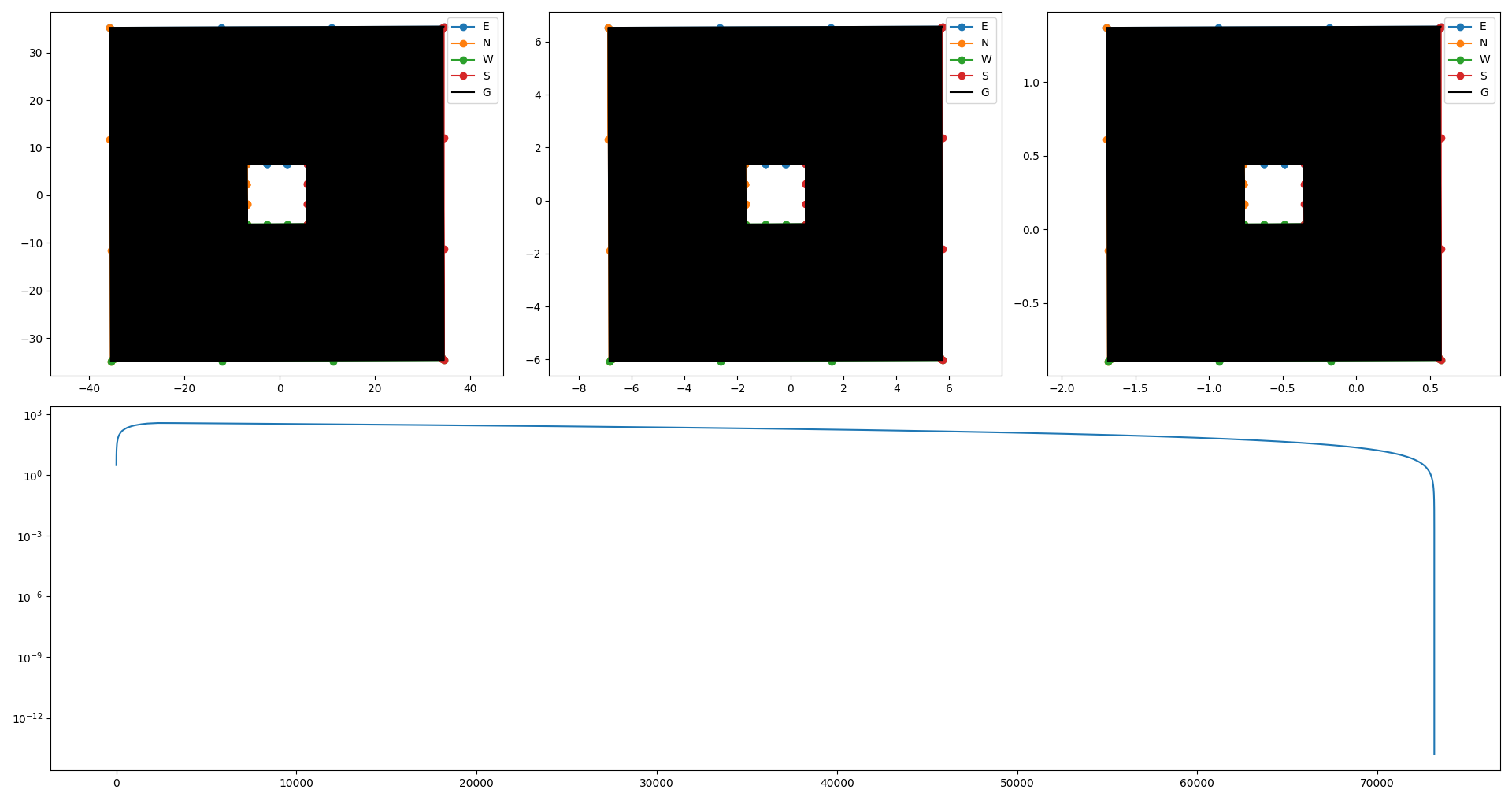
The last curve summarised the continual reduction of the configuration's diameter many orders of magnitude below its inital value in finite time.
Few more examples with
g < 3.001
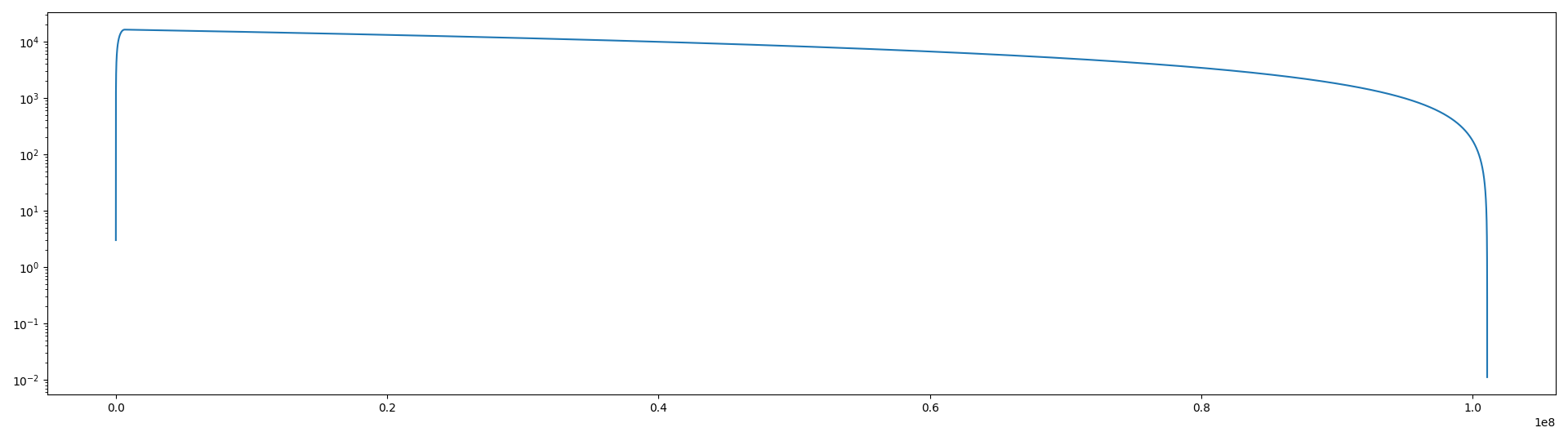
and the same with reduced shrinkage reduction (6% instead of 50%).
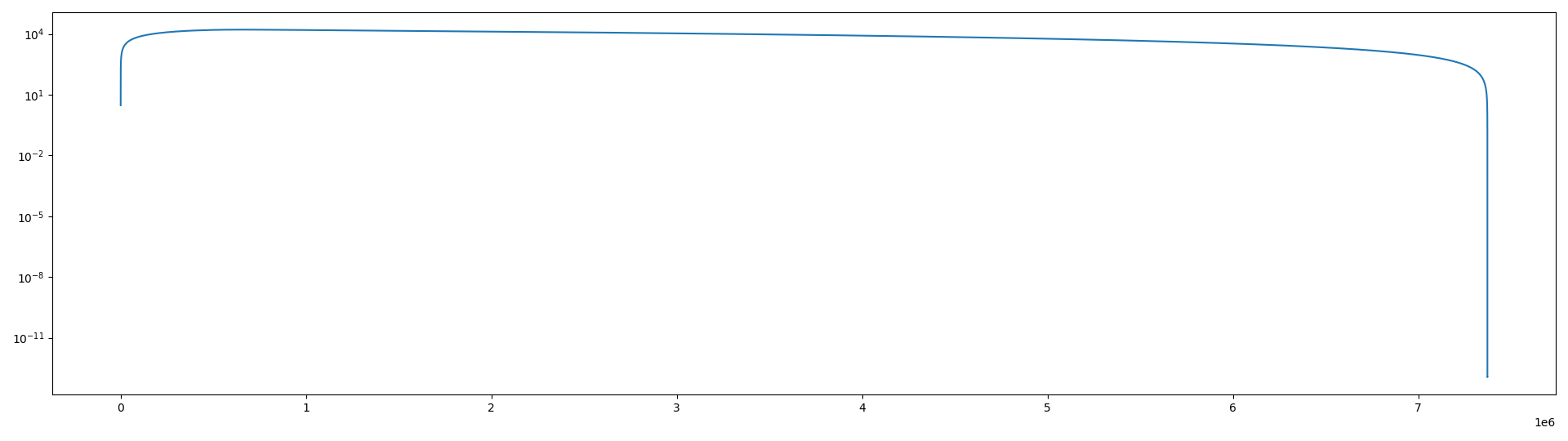
g < 3.0003 ; shrinkage reduction < 1%

g < 3.00003 ; shrinkage reduction < 1‰