Going off on multiple tangents

Question

Given two disjoint circles of diameters D₁ and D₂ draw all shared tangents. Find the intersection points of all pairs of tangents. Discard those collinear with the two circle centre points. Of the remaining points take all pairs which are not on the same tangent.

What is the product of the distances of these pairs of points?

Note 1: You may ignore the (boring) case of one circle being inside the other.

Note 2: Please back up your answer with a derivation.

Note 3: As usual, bonus points for brevity, originality, beauty and wisdom.

HINT:

The distances to be multiplied are indicated in red.

Answer 1

I'm going to show that the product is

$D_1D_2$.

This is obvious if $D_1=D_2$, so we will assume WLOG $D_1<D_2$.

Let's start by making another drawing:

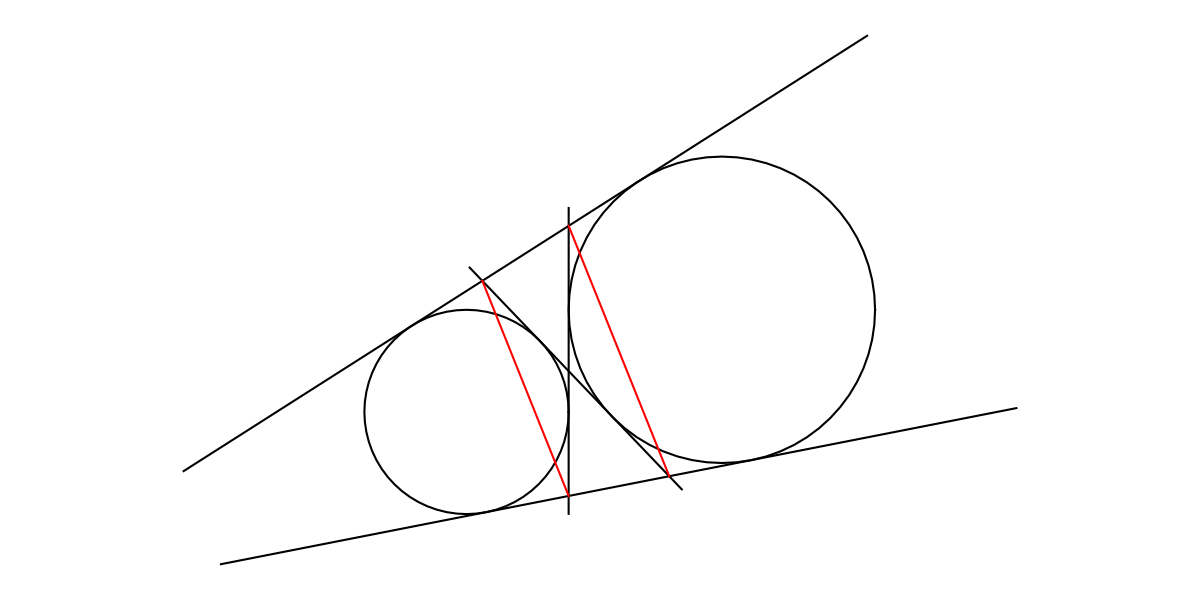
The 4 tangents intersect in points $B,C,B',C'$; there are two more intersection points which were discarded because they lie on the line through the circle centres. We have labelled the far of the two as $A$. Because the configuration is mirror symmetric the triangles $ABC$ and $AB'C'$ are congruent with sides $a=BC=B'C',b=CA=C'A,c=AB=AB'$ and the quadrilateral $BB'CC'$ is a trapezoid, in particular, it is cyclic; the circumcircle is indicated in orange. Let us call the sides of the trapezoid $x=CC',z=BB',y=BC'=CB'=c-b$.

Now it is time to call in the big boys

Ptolemy and Heron.

The desired product is by Ptolemy's theorem

(1) $xz = a^2-y^2 = (a+y)(a-y) = (a-b+c)(a+b-c)$.

The last product also occurs in Heron's formula for the area $S$ of $ABC$ (and $AB'C'$)

(2) $16S^2 = (a+b+c)(-a+b+c)(a-b+c)(a+b-c)$.

As the given circles happen to be the in- and one excircle of both $ABC$ and $AB'C'$ we can also express the area as $4S=D_1(a+b+c)=D_2(-a+b+c)$ or multiplied together

(3) $16S^2 = (a+b+c)(-a+b+c)D_1D_2$.

Equating the r.h.s.s of (2) and (3), cancelling common factors and then comparing with (1) yields $xz = D_1D_2$ as contended.