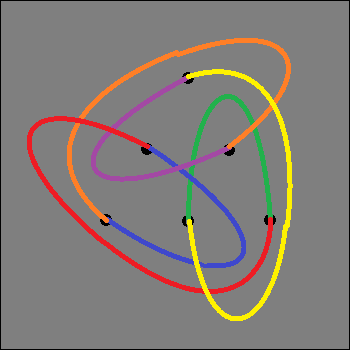
Can you connect 6 dots with 6 curves, such that every pair of curves touch each other exactly once? Curves can touch anywhere at their interior or at the dots, and the whole thing must lie in the plane.
-
$\begingroup$ By "touch" do you mean "tangent" or do you also include "intersecting"? $\endgroup$– justhalfCommented Jul 7, 2021 at 12:23
-
$\begingroup$ intersection is included $\endgroup$– Dmitry KamenetskyCommented Jul 7, 2021 at 12:40
-
1$\begingroup$ Can I pick what surface I do this on? $\endgroup$– msh210Commented Jul 7, 2021 at 13:49
-
$\begingroup$ @msh210 no it has to be on the plane $\endgroup$– Dmitry KamenetskyCommented Jul 7, 2021 at 23:29
2 Answers
-
1$\begingroup$ Correct and well done! You have rediscovered a 6 point thrackle. John Conway has offered $1000 for a solution to the following question: can a thrackle have more curves than dots? en.m.wikipedia.org/wiki/Thrackle $\endgroup$ Commented Jul 8, 2021 at 1:40
-
1$\begingroup$ @DmitryKamenetsky whilst I'm sure any problem that Conway offered money for was interesting in its own right, did Conway nominate anyone to judge this and other similar offers after his death, or has that $1000 offer now expired? $\endgroup$– SteveCommented Jul 8, 2021 at 8:27
-
$\begingroup$ @Steve no idea. I know the problem is still unsolved. Even without the money it is worth tackling it. $\endgroup$ Commented Jul 8, 2021 at 12:54
Here's something:
RGB and CMY form two "triangles", so each of the groups of three is touching its other members - the only tricky part is making every side of one triangle touch the other triangle.
Cyan is tangent to Green at the spot it's supposed to touch, Magenta and Yellow are tangent to Blue.
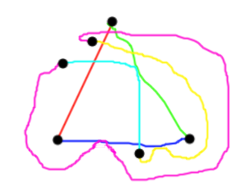
Alternatively, if you actually need connectedness,
connecting them in a cycle still works:
Here, white squares are "dots" and grey squares are interior intersections.
-
$\begingroup$ That is correct! Is it possible to make all interior touches as intersections? $\endgroup$ Commented Jul 7, 2021 at 12:55
-
$\begingroup$ No - in both the 6-cycle and the 3+3 (the only two options unless you allow degree-1 dots and thus certain exotic graphs) you have a distinguishable "interior". Since the number of interior touches (nine) is odd, you'd end up on opposite sides of the border if you had no tangencies. $\endgroup$ Commented Jul 7, 2021 at 13:01
-
1$\begingroup$ It is in fact possible ;) $\endgroup$ Commented Jul 7, 2021 at 13:14
-
$\begingroup$ @AxiomaticSystem a circular rubber band (when divided into 6 segements is a 6-cycle) can intersect itself once (odd) too without any problem. $\endgroup$– justhalfCommented Jul 8, 2021 at 8:37