Assemble lozenges

Question

You have a large number of 60° rhombi called "lozenges." Each lozenge has its edges marked with four distinct symbols drawn from an infinite alphabet. Lozenges may be rotated by 180° or reflected across either axis. A triple of lozenges forms a "hex" if the markings can be matched up in the following way:

     _____B_____
    /\          \
   /  \          \
  C    E          A
 /      \          \
/        \____D_____\
\        /          /
 \      /          /
  A    F          C
   \  /          /
    \/_____B____/,

where A, B, C, D, E, F are distinct symbols. You wish to assemble all of your lozenges into hexes. Here are the conditions:

• You have access to a machine that can be programmed to produce arbitrary hexes; these hexes can be broken into new lozenges
• The total number of lozenges is a multiple of three
• For lozenge-type that you have, you have an even number of that type

Is it always possible to assemble all of your lozenges into hexes?

Answer 1

I say:

Yes, it's possible.

Visual proof:

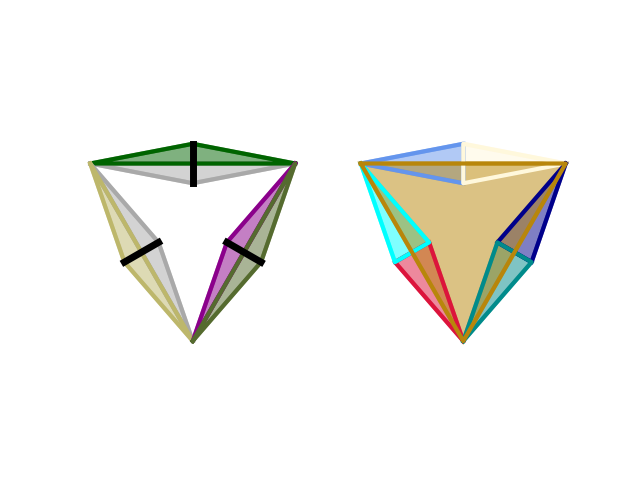
Short explanation
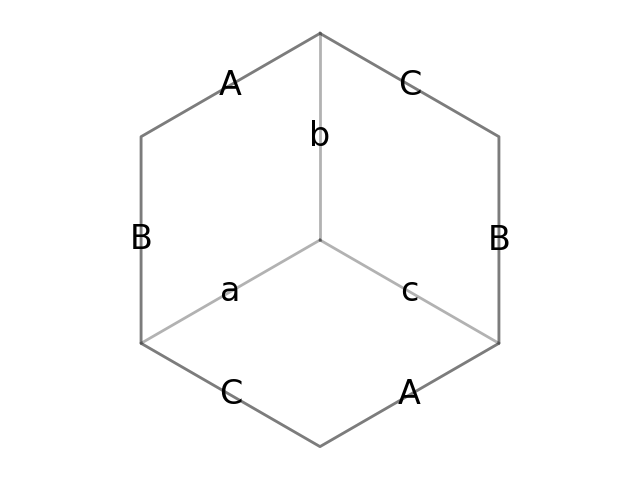
each edge is a lozenge, each point is a letter pair (opposite edges of lozenge), each triangle is a hex
left panel: black lines are pairs of identical orphans, the triangles are hexes you produce with the magical machine
right panel: this is how to rearrange the lozenges into hexes; there are two copies of the large regular triangle; no more orphans!

Longer explanation
consider a lozenge L with edges (ccw) a,b,c,d. Because we are allowed to flip and rotate this is the same as b,a,d,c ; c,d,a,b ; etc. Another lozenge M can be in the same hex with L if and only if it shares precisely one pair of opposite labels with L, i.e. M must look like x,a,y,c or x,b,y,d. It therefore makes sense to consider pairs of labels P = a,c ; Q = b,d ; R = x,y ; etc. and to characterize lozenges in terms of these: L = P,Q ; M = P,R or Q,R.
This lends itself to a graph representation, each pair of labels is a vertex, each lozenge is an edge connecting its two pairs of labels.
It is now easy to check that three lozenges can form a hex if and only if they form a triangle in the graph representation.
The left panel contains three pairs of lozenges (thick black lines) which represents part of what we are given. We can use the hex generating machine to fill in everything else. In more detail: We create three new pairs of labels and generate six hexes as indicated always using two of the new label pairs and one given pair. We then break up all six hexes add the obtained 18 lozenges to the 6 given ones and reassemble into 8 hexes (The hex containing only new label pairs is created two times) as indicated by the right panel.

One more detail:
It is important to use ordered pairs, because, for example for lozenges a,b,c,d (ccw starting at pointy end) ; a,e,c,f ; e,d,f,b or in pair notation ac,bd ; ac,ef ; ef,db no hex can be formed (bd != db) To see that with ordered pairs it will always work lets adopt the convention that pairs are always named aA,bB,cC, etc. Then all obtuse angles will between either two small letters or two caps which makes it obvious that everything is compatible.