7
$\begingroup$

How can 4 chess queens be placed on a 6x6 chessboard so that every empty square is attacked by at least one queen AND no queen attacks another queen.

Clarification: Although I did not mention it in my initial post, my intention was that 4 queens would be placed on the chessboard and no other pieces.

Improve this question
$\endgroup$

3 Answers 3

Reset to default
14
$\begingroup$

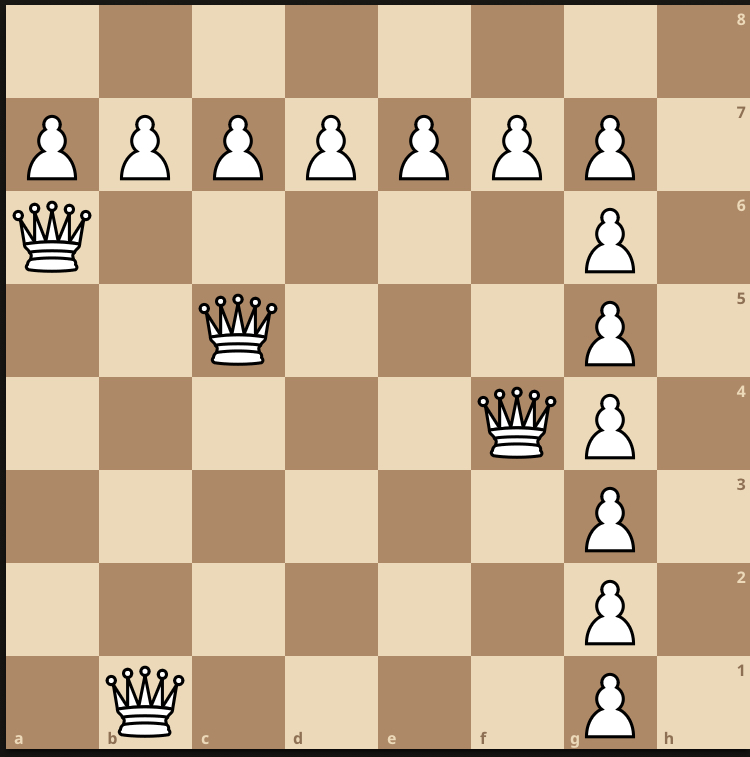
A simple symmetrical solution is

.Q....
.....Q
......
......
Q.....
....Q.

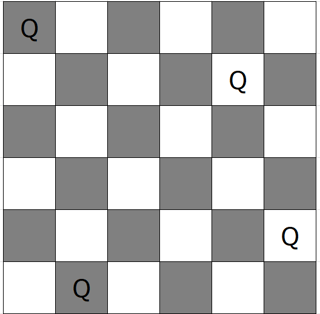
This can be found by looking at this specific placement of a queen

x...x.
x..x..
x.x...
xx....
Qxxxxx
xx....

and noticing a few properties:

  • The four rotations of this queen do not attack each other.
  • Since it attacks the first column, its four rotations attack the 1st and 6th rows and columns.
  • Since it attacks the 5th row, its four rotations attack the 2nd and 5th rows and columns.
  • This leaves the four cells at the center. The given queen attacks one of them (call it X), so its four rotations attack four rotations of X, which covers all four cells at the center.

With the help of a computer program, it can be found that there are

17

essentially different solutions:

Q.....    Q.....    Q.....    Q.....
.....Q    ....Q.    .....Q    .....Q
.Q....    ......    ......    ......
......    .Q....    .Q....    ......
......    ...Q..    ...Q..    .Q....
..Q...    ......    ......    ....Q.

Q.....    Q.....    ..Q...    ......
......    ......    Q.....    Q.....
...Q..    .....Q    ......    ....Q.
.....Q    ..Q...    .Q....    ......
......    ......    .....Q    .Q....
.Q....    ...Q..    ......    ...Q..

....Q.    ..Q...    ..Q...    ....Q.
Q.....    Q.....    Q.....    Q.....
......    ......    ......    ......
.....Q    ....Q.    ......    ......
.Q....    ......    .....Q    .....Q
......    .Q....    .Q....    .Q....

..Q...    ..Q...    ...Q..    ...Q..
Q.....    Q.....    Q.....    Q.....
......    ......    ......    ......
...Q..    ......    .....Q    .....Q
.....Q    .....Q    ..Q...    ......
......    ...Q..    ......    ..Q...

...Q..
......
Q.....
.....Q
......
..Q...

Improve this answer
$\endgroup$
0
10
$\begingroup$

I am pretty sure there is more than one way of doing it but here is one solution:

enter image description here

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Sniped by 18 seconds! :P (Although answers are a bit slightly different) $\endgroup$
    – Beastly Gerbil
    Commented Jul 21 at 20:47
  • 1
    $\begingroup$ My intention was that only 4 queens were allowed; no other pieces. $\endgroup$
    – Will.Octagon.Gibson
    Commented Jul 21 at 23:31
  • 18
    $\begingroup$ @Will.Octagon.Gibson I am pretty sure PDT only added pawns to restrict the board to 6x6, they aren't actually part of the solution and are supposed to just indicate the boundary $\endgroup$
    – Beastly Gerbil
    Commented Jul 21 at 23:45
  • 1
    $\begingroup$ @BeastlyGerbil That makes sense. $\endgroup$
    – Will.Octagon.Gibson
    Commented Jul 21 at 23:47
8
$\begingroup$

Place the queens like so:

enter image description here

Interestingly it only takes 3 if they are allowed to attack each other

Improve this answer
$\endgroup$
1
  • $\begingroup$ Ah damn just sniped me $\endgroup$
    – PDT
    Commented Jul 21 at 20:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.