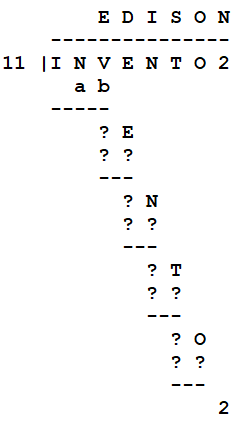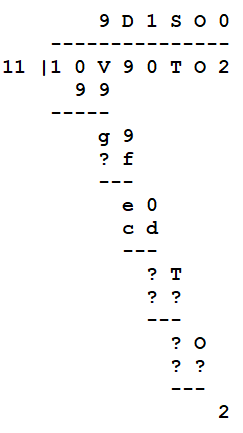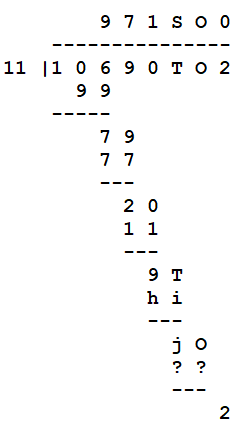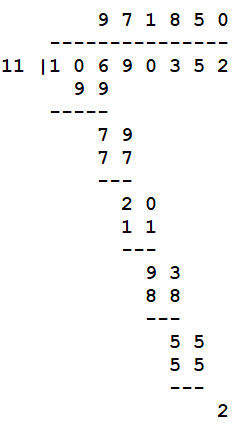Thomas Alva Edison was born on February 11, 1847.
https://en.wikipedia.org/wiki/Thomas_Edison
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
It is given to us that R = 2.
Now, INV - ab is a single-digit number and ab is a multiple of 11. Even if ab were as high as 88 and INV as low as 100, their difference would be 12 which has two digits. Therefore ab = 99 and 100 <= INV <= 108 (not 109 since 109 - 99 = 10, again two digits). But that implies E = 9, and IN will always be 10 (hence I = 1 and N = 0).
Also, I = 1 implies cd = 11. e0 - 11 is a single digit so e = 2. But that makes f = 7, meaning ?f = 77 since it has to be a multiple of 11, meaning D = 7. Also, g9 - 77 = 2 so g = 7. That results in 10V - 99 = 7, meaning 10V = 106 (so V = 6).
9T - hi is a single digit again. So hi would never be able to be as high as 77, since even if we make 9T as low as 90, 90 - 77 = 13. Also hi cannot be 99 since it is implied that the difference is nonzero, so hi = 88 which implies S = 8. Therefore T = 3, 4, or 5. Also jO is a multiple of 11 since jO - ?? is zero, so j = O.
If we make T = 4, then 9T - 88 = 94 - 88 = 6 = j = O, but O cannot be 6 since V already is. If we make T = 5, then j = O = 7, but O cannot be 7 since D already is. Therefore T = 3, and so j = O = 5. We can then complete the alphametic.
In conclusion:
N = 0, I = 1, R = 2, T = 3, O = 5, V = 6, D = 7, S = 8, and E = 9.
$\begingroup$
$\endgroup$
4
971840
_________
11 | 10690242
99
--
79
77
--
20
11
--
92
88
--
44
44
--
2
What about this solution?
What is wrong here?
-
$\begingroup$ Usually in alphametic puzzles, different letter stands for different digit. This answer fails at that point. Anyway, it is not stated in the original post $\endgroup$– ACBCommented May 26 at 15:53
-
$\begingroup$ Please I know that. Can you please show me witch letter/ digit failed this constraint. Thank you. $\endgroup$ Commented May 26 at 18:09
-
$\begingroup$ You have T=R=2, contrary to the standard restrictions of alphametic puzzles. $\endgroup$ Commented May 26 at 18:18
-
$\begingroup$ I'm very much thank you. that skipped my eyes. $\endgroup$ Commented May 26 at 18:20