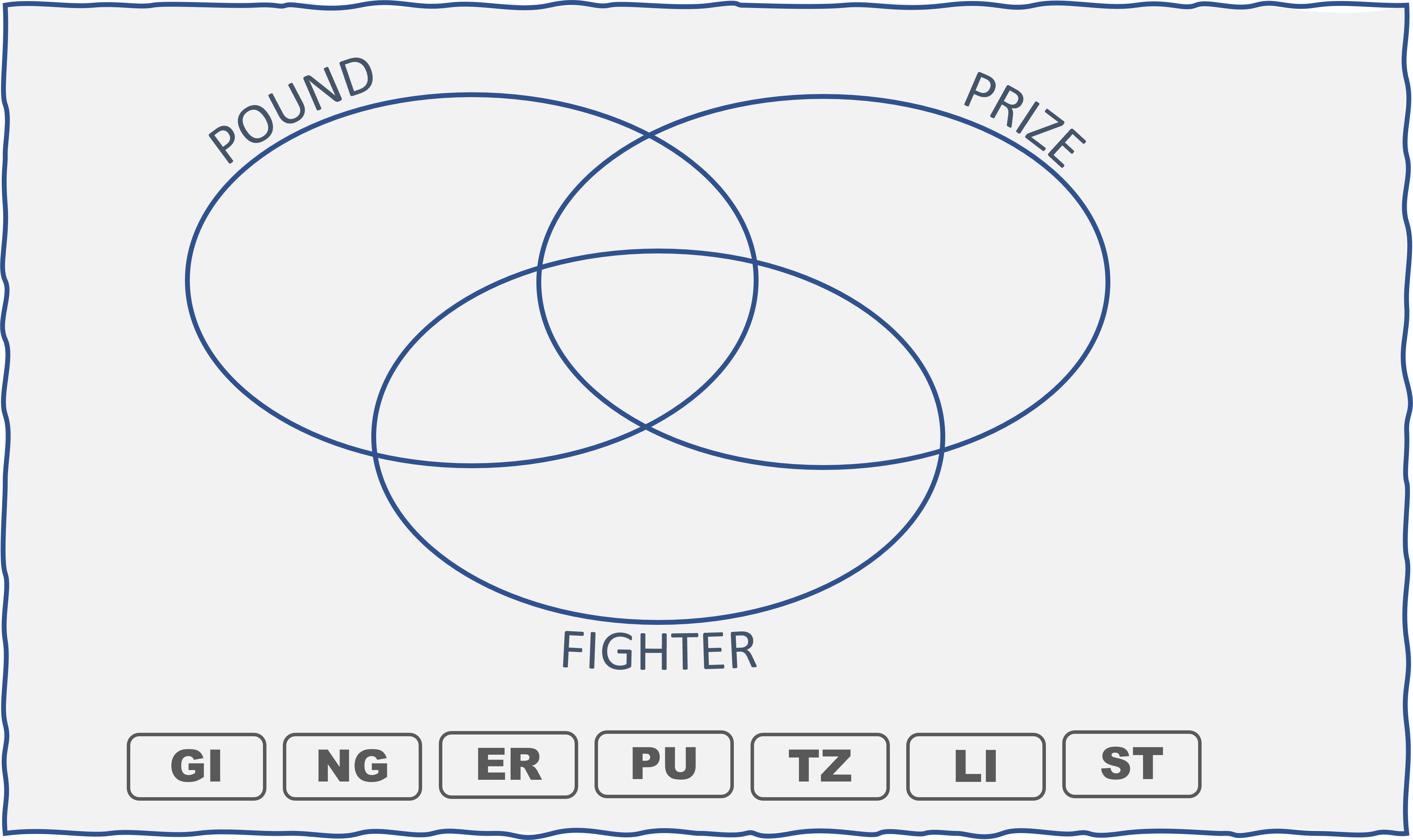
The three overlapping ellipses form seven curved regions - place one tile in each region so that the tiles in any one ellipse can be re-arranged into an eight-letter solution to the corresponding clue!
-
$\begingroup$ Do the letters in each two-letter tile have to be in order in the eight-letter word? Or can all eight letters be rearranged into any order? $\endgroup$– Rand al'ThorCommented Nov 5, 2022 at 21:31
-
$\begingroup$ Letters cannot be rearranged within tiles. (At least, in my answer they aren't.) $\endgroup$– Gareth McCaughan ♦Commented Nov 5, 2022 at 21:32
2 Answers
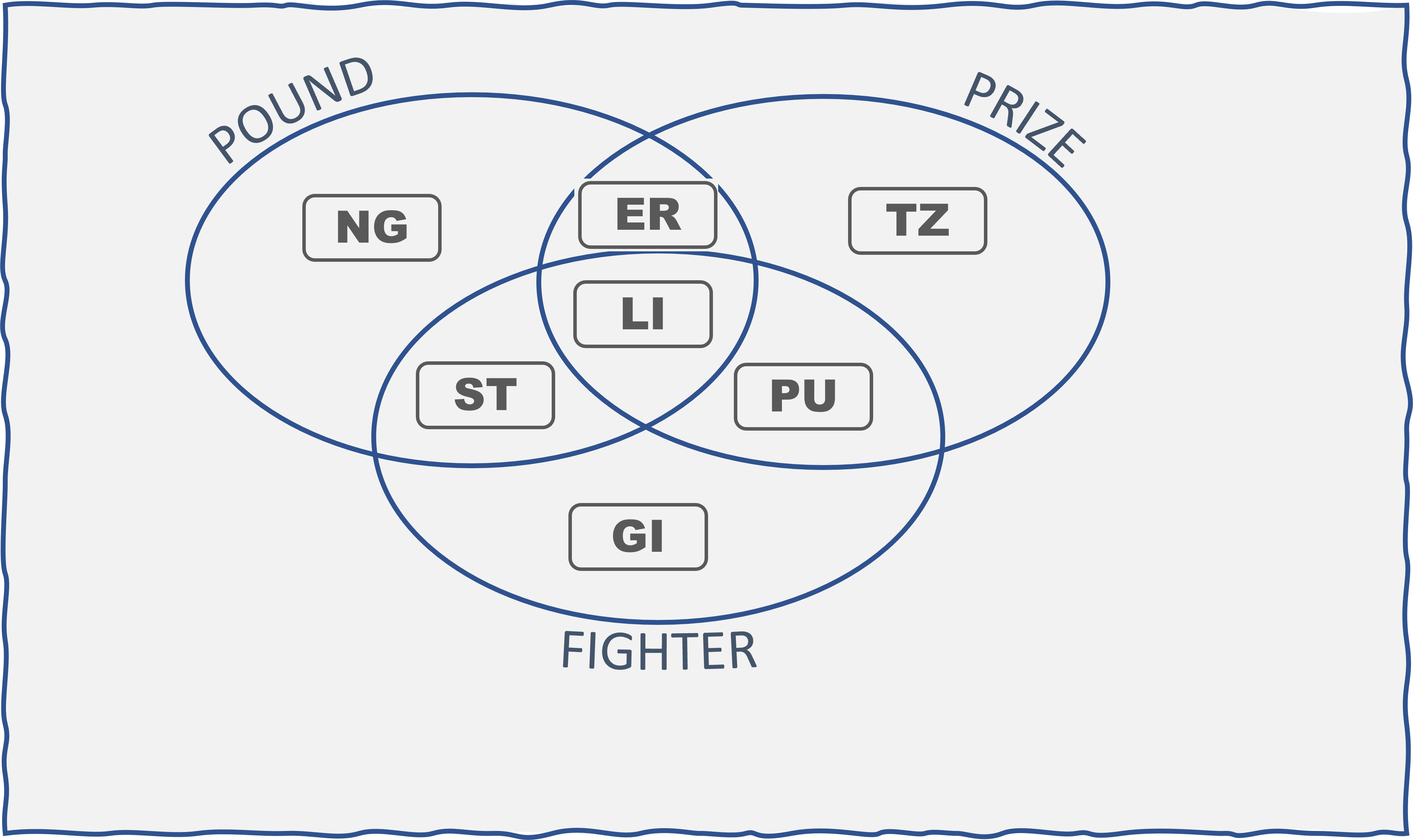
Complete solution:
The first word I got (perhaps surprisingly) was
fighter = PUGILIST.
Realising that "NG" is probably at the end of a word and preceded by a vowel, it didn't take much fiddling to get
pound = STERLING.
And then, by a process of elimination, the final word must be
prize = PULITZER.
Oh and the title?
"Beatboxer" means a boxer who beats people, not the other meaning.
The words are
PULITZER prize, PUGILIST fighter, pound STERLING
and the pieces are arranged as follows:
prize only: TZ; fighter only: GI; pound only: NG;
prize fighter only: PU; prize pound: ER; pound fighter: ST;
all three: LI.