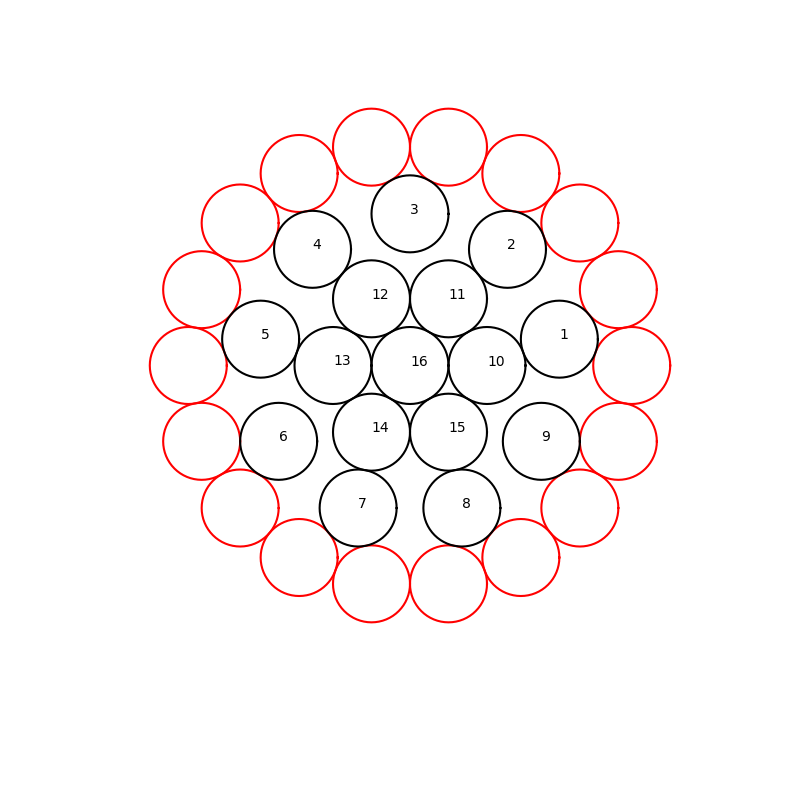
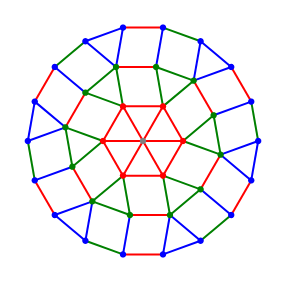
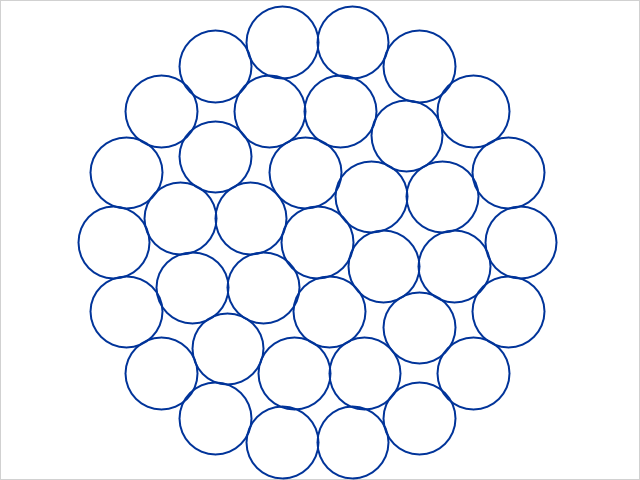
Two circles are touching if and only if the length of the line segment formed by connecting their centers has length $1$ (well technically, $d$, but I'm just going to set $d=1$ because the scale doesn't really matter).
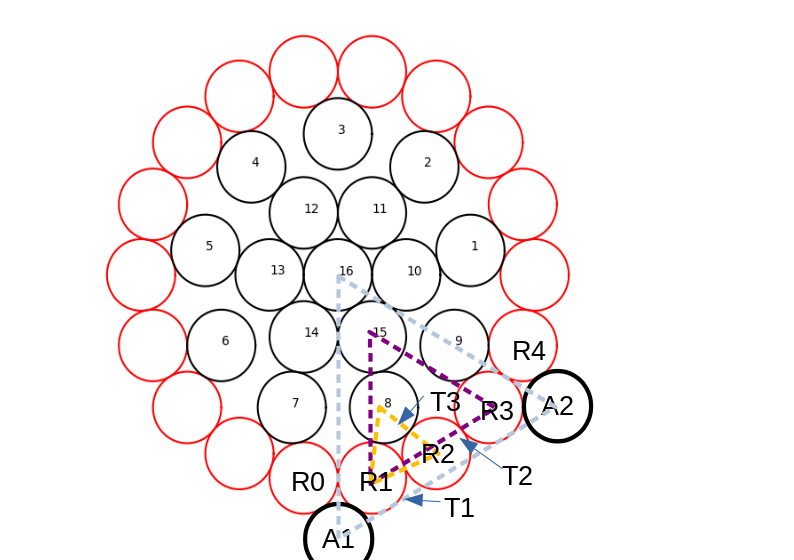
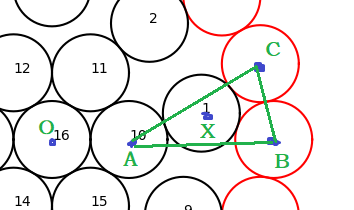
Let's only consider circles 16 (let this be $A$), 14 ($B$), 15 ($C$), 7 ($D$), the circle below it to the left ($E$), the other circle touching $D$ and $E$ ($F$), 8 $(G)$, the circle below it to the left ($H$), and the other circle touching $G$ and $H$ ($I$).
Thus, $AB=BC=AC=DE=EF=DF=GH=HI=GI=1$ because we know they are all touching. Also, $FH=1$.
The internal angle of an 18-gon is $\angle EFH=\angle FHI=\frac{16\pi}{18}$. The internal angle of an equilateral triangle is $\frac\pi3$ so we can determine that $\angle DFH=\angle EFH-\angle EFD=\frac{16\pi}{18}-\frac\pi3=\frac{5\pi}9$.
Connect $DG$ and draw an altitude from $F$ to this line. Then, label the connecting point $J$. $\angle DFJ=\angle DFH-\angle JFH=\frac{5\pi}9-\frac\pi2=\frac\pi{18}$. Note that $\cos\frac\pi{18}=\frac{\text{adjacent}}{\text{hypotenuse}}$. In this case, $\text{adjacent}=JF,\text{hypotenuse}=DF=1$, so $JF=\cos\frac\pi{18}$.
Now, using the apothem formula, $a=\frac s{2\tan\frac\pi n}$. The side length is $s=1$ and the number of sides is $n=18$ (this is for the entire 18-gon), therefore $a=\frac1{2\tan\frac\pi{18}}=\frac12\cot\frac\pi{18}$. This is the length of the altitude if we draw one down from $A$ to $FH$.
Now, from that, subtract the altitude from $A$ to $BC$ (this and the above altitude are clearly both vertical and thus if we subtract them, we get the height of the hexagon $BCGHFD$, which is true because $BC$ and $FH$ must be horizontal for this to be E-W symmetric). Using simple Pythagorean formula, that length is $\frac{\sqrt3}2$. Therefore, the height of this hexagon is $\frac12\cot\frac\pi{18}-\frac{\sqrt3}2$.
Note that due to E-W symmetry and that $BC=FH$, $B$ must be vertical to (above) $F$, and $C$ to $H$. Also, we have $JF$, which is the height of the lower half of the hexagon. Now it remains to prove that the upper half is the same height, and we can prove that this hexagon is symmetric.
$\begin{align*}\frac{\frac12\cot\frac\pi{18}-\frac{\sqrt3}2}{\cos\frac\pi{18}}&=\frac{\frac{\cos\frac\pi{18}}{\sin\frac\pi{18}}-\sqrt3}{2\cos\frac\pi{18}}\\&=\frac{\csc\frac\pi{18}-\sqrt3\sec\frac\pi{18}}2\\&=2\end{align*}$
(According to Wolfram Alpha. Hopefully that's acceptable :P)
Therefore, this proves that the vertical distance from $BC$ to $DG$ is the same as from $FH$ to $DG$. Also, $B$ and $F$, and $C$ and $H$ are vertical to each other. Therefore, hexagon $BDGHFD$ is symmetric across $DG$. Therefore, $BD=DF$ and $CG=GH$. These equal $1$ (or $d$, technically).
Therefore, circles 7 and 14 touch, and 8 and 15 touch (the second could be given by symmetry based on the first, anyway). Since this is rotationally symmetric at 120 degrees, therefore 1/10 and 2/11 touch, and finally, 4/12 and 5/13 touch.
QED