If i understand the problem corectly, it seems your reasoning is that you cant go "up" in your diagram, nor can you start at the second row.
if you start with all the fives of the second row, or start with the middle 5 of row two, go up then down, that is two additional ways for each extremity of the second row, if you find other ways for the middle 5 of row 2, you might end up with blue numbers being 5-6-5, which then double for each row, and eventually en up at 256 when summed
As you wanted a view of all the 5 possible paths for the leftmost 5 :
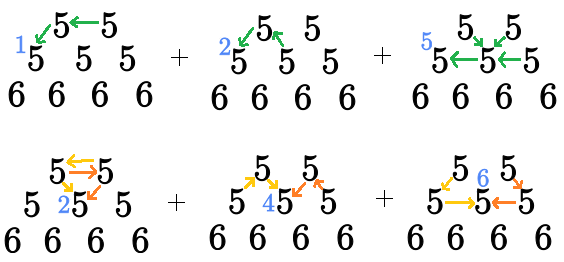
as for a generalization, i don't really know, pascal triangle is good if your number can only be read from top to bottom, but as soon as you go up, or if you can start on another row than the first (think palindromic numbers, or a number composed oly of 10 times the same digit), then the numbers are going to be much greater.
