I fully copied below answer from http://math.stackexchange.com/questions/227285/constructing-the-midpoint-of-a-segment-by-compasshttps://math.stackexchange.com/questions/227285/constructing-the-midpoint-of-a-segment-by-compass
Some Googling revealed the following comments to this answerthis answer:
- I know it is possible, but is there an easy way to divide a segment in half with only a compass? – robjohn♦ May 20 at 3:46
- I don't know if that's "easy", but here's one method:
- Find the point $C$ on the ray from $A$ through $B$ such that $|AC|=2|AB|$ using my previous comment [The relevant part: "To double the distance along a ray, use the construction of a regular hexagon with vertex $A$ and center $B$".]
- Intersect the circle with center $C$ through $A$ with the circle with center $A$ through $B$ to find $D_1,D_2$.
- The midpoint of $AB$ is the second point of intersection of the two circles with center $D_i$ through $A$. – t.b. May 20 at 9:28
- Here is a picture of what I have in mind: - t.b. May 20 at 12:38
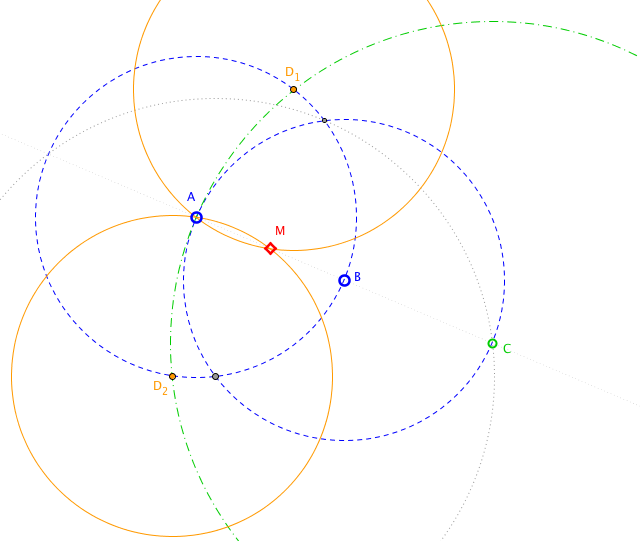
The dotted line is not used in the construction.
Added:
The triangles $\Delta ACD_1$ and $\Delta AMD_1$ are isosceles by construction and they share a common angle, hence they are similar. Therefore $AM : AB = AM : AD_1 = AD_1 : AC = AB : AC = 1 : 2$.
