How many balls can fit in the box? [closed]

Question

I think the answer isn't as simple as the question. So, I need your help. :)

Assumed I have a box, sized 100cm X 100cm X 100cm (width X length X height). How many balls can fit in that box (the ball is 1cm in diameter)? Thanks.

Answer 1

Quoting from wikipedia:

Experiment shows that dropping the spheres in randomly will achieve a density of around 65%. However, a higher density can be achieved by carefully arranging the spheres as follows. Start with a layer of spheres in a hexagonal lattice, then put the next layer of spheres in the lowest points you can find above the first layer, and so on – this is just the way you see oranges stacked in a shop. At each step there are two choices of where to put the next layer, so this natural method of stacking the spheres creates an uncountably infinite number of equally dense packings, the best known of which are called cubic close packing and hexagonal close packing. Each of these arrangements has an average density of $\frac\pi {3\sqrt2} = 0.740480489...$. The Kepler conjecture says that this is the best that can be done—no other arrangement of spheres has a higher average density.

So your answer is

$\frac{\text{box volume}}{\text{ball volume}} \times {\text{packing density}} =$

$\frac{100^3}{\frac43 \times \pi \times \left(\frac12\right)^3} \times {\frac\pi {3\sqrt2}} =$

$\frac{100^3}{\left(\frac12\right)^3\times 4 \times \sqrt2} =$

$\frac{100^3}{\frac12 \times \sqrt2} = 100^3 \times \sqrt2 \approx 1414213$

Answer 2

Since this is supposed to be a puzzle (not just a math question), I assume any loophole can be used. So, I'll say an infinity of balls can fit in the box.

The trick is that the description doesn't explicitly require all the balls to fit at the same time in the box. Since any 1cm diameter ball can fit the box, there's an infinity of existing and hypothetical balls that can fit in the box.

Answer 3

1401611

The optimal pattern of packing spheres is pretty well known, not as widely known is the fact that this pattern has 7 different orientations where the spheres are all organised in layers of simple patterns. In four of these orientations the spheres are aligned in a triangular pattern, in the three other planes they are aligned in a square pattern. The key to this puzzle is to rotate the pattern so that the wasted space along the sides of the box is minimised.

An orientation where the three square planes are aligned with the walls of the box happens to fit extremely well, as a 71 ball wide configuration is $99.995\text{ cm}$ wide. In this configuration the bottom of the box is filled like so:

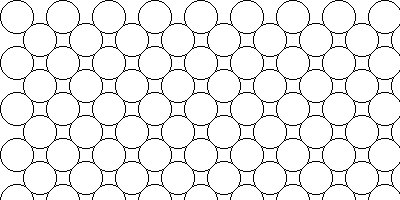
With 71 balls bordering each edge, for a total of $71^2+70^2=9941$ balls in the bottom layer, the layer on top of it will use the same pattern, but be shifted so that the balls lie in the holes between the balls in the bottom layer, this will allow one ball less. These two layer configurations are then repeated until there are 71 configurations like the first layer, and 70 like the second layer. This gives a total of $71\times9941+70\times9940=1401611$ balls.

Answer 4

I don't have the exact value or formula to how to calculate this. But I want to show how you should think.

So if this question was in some simple elementary school test, answer should be obviously 100x100x100=10 ⁶ in total. Because it uses simple square packing.

However there are few other more packing styles like hexagonal packing, you can actually use empty spaces that occurs on square packing.

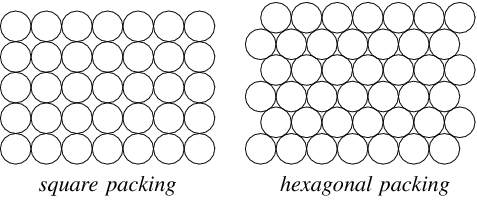
As one can see, in square packing height of two lines is 2cm. But in hexagonal packing it is less then 2cm. You can try and use much more space with different packing types. After finding right packing, you can calculate exact value.

Answer 5

If the structure of the spheres does not need to be maintained, you could melt down the spheres and they would occupy $.52\ \text{cm}^3$ (from the volume of a sphere: $\frac43 \pi r^3$) each resulting in:

$1909859 = \frac{100^3}{\frac43 \pi (.5^3)}$

Answer 6

Well, assuming each ball occupies ~1 cm ³ of space, you have fit in 1,000,000 balls just by having them in a square lattice.

Answer 7

Two loopholes:

1. We can deflate the balls, which will decrease the volume taken up by them. In this case the amount will depend by the width of the "skin" of the ball.
2. We take "the ball" that is 1cm in diameter and many other balls that are of smaller sizes. Since we can potentially mathematically assume them to be as small as possible the answer will approach infinity. In practice we are limited by the plank volume, but i am unsure if we can call that truly a "ball" at all, so the question becomes how few plank volumes do we need, to be able to claim that something is a ball at all?

Answer 8

We will assume that we want to find the largest number of balls that can fit in the box when they are hexagonal close packed. The other answers have ignored the edge effects, using the density of balls in an infinite lattice. Let the balls be aligned in the $x$ direction, so each alternate horizontal line on the first layer will hold $100$ and the ones between will hold $99$ balls. The vertical spacing between the rows is $\frac {\sqrt 3}2 \approx 0.8660254$, so we get $\left \lfloor \frac {99 \cdot 2}{\sqrt 3}\right \rfloor +1=115$ rows. That gives us $58 \cdot 100 + 57 \cdot 99=11443$ on the bottom layer. The next layer also has $115$ rows, but they alternate the other way and we only get $ 11442$ balls We get $115$ layers, so in total we get $58*11443+57*11442=1315888.$ We can probably get closer to the density limited $1414213$ by using a smarter configuation, but I am sure we won't get all the way there.