The full list of possibilities that each prime can be adjacent to is as follows:
2: $3,7,11,23,47$
3: $2,7,13,19$
5: $11,31,41$
7: $2,3,11,23,29,43$
11: $2,5,7,47,53$
13: $3,17,23,29$
17: $13,19,47,53$
19: $3,17,23$
23: $2,7,13,19,41$
29: $7,13$
31: $5,47$
37: $41,53$
41: $5,23,37$
43: $7,47$
47: $2,11,17,31,43,53$
53: $11,17,37,47$
The key ones to notice are
$29,31,37,43$, each of which has only two others it can be adjacent to. Three of these even join up to each other, so we get a string of adjacent numbers $13,29,7,43,47,31,5$.
Now there are only two possibilities for the other number next to $5$, namely $11$ or $41$.
If it's $41$, then next to that must be $37$ and then $53$, while now $11$ can only be adjacent to $2$ and $53$. Now there's only four numbers remaining (between $13$ and $2$ on the circle), namely $3,17,19,23$. Considering the list that $17$ can be adjacent to, we see that next to $13$ must be $17$ and then $19$, leaving $3$ and $23$ adjacent, contradiction.
So it must be $11$ next to $5$, then next to that must be either $2$ or $53$.
-
If it's $53$, then next to that must be $37$ and then $41$ and then $23$, leaving only four numbers ($2,3,17,19$) left to place between $13$ and $23$ on the circle. Looking at the options for $17$, we see that next to $13$ must be $17$ and then $19$, then $3$ and finally $2$ next to $23$.
-
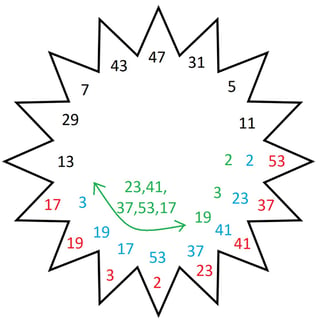
If it's $2$ next to $11$, then we know the string $23,41,37,53,17$ (in either direction) but not where to place it. Apart from that string of length five, only $3$ and $19$ are left to place, all seven numbers somewhere between $13$ and $2$ on the circle. Now $19$ can't be next to either $13$ or $2$, so either we start next to $13$ and place $3$ then $19$ then $17,53,37,41,23$, or we start next to $2$ and place $3$ then $19$ then the string of length five in either possible order.
So altogether we have
four possibilities, which means Gaby has three children.
I've tried to illustrate this as follows: