Wrap-up: the making of Honeydripping around the clock
This is not a solution to the puzzle but provides notes from its poser. This type of answer has been approved by the community.
Caution: This post contains information about the solution.
This is the third experiment with self-defining hexagonal-tiling mazes inspired by Two honeycomb hints and, especially, Two honeycomb hints, part two by Yuriy S.
The first experiment was a simple compact maze with unwieldy sums: H-one-one-oneycomb. Next came a maze with almost-trivial sums and additional guiding features: How to fill a honeymoon.
So it was time to explore structures whose traversals could feel more like journeying than like space-filling. The conceptual point of departure was to simply have a hexagon made of hexagons made of hexagons, which led to a variety of possible forms.
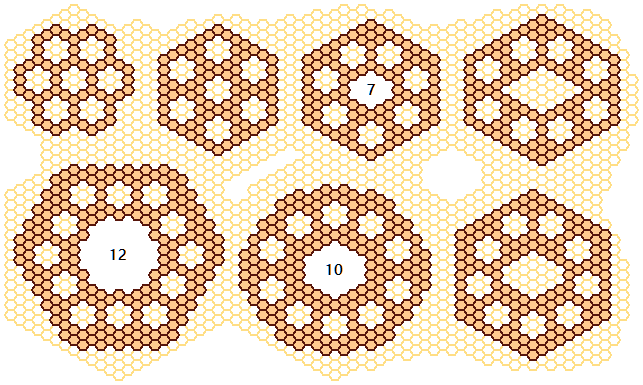
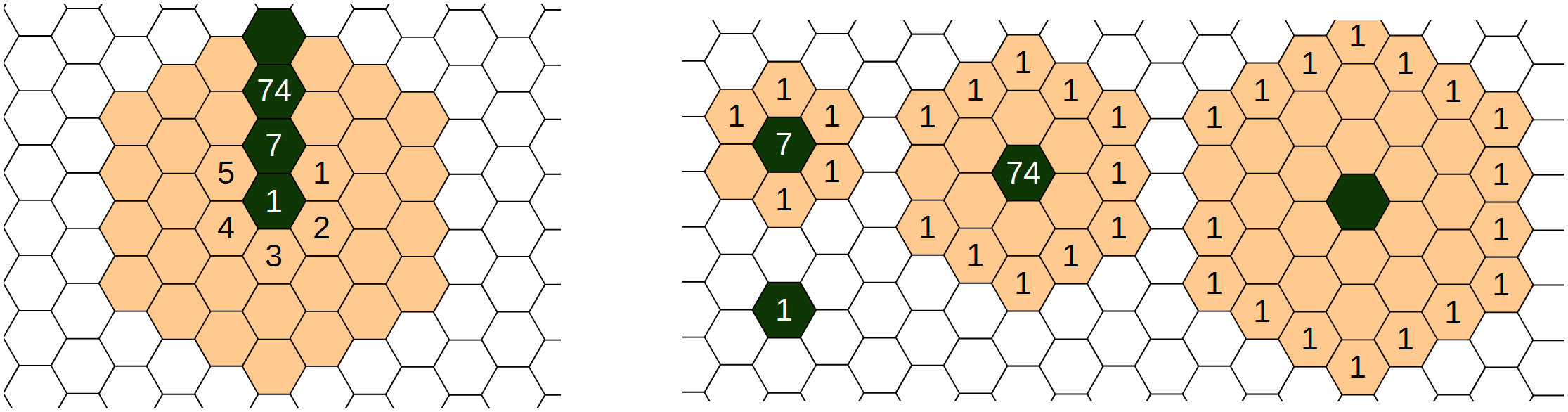
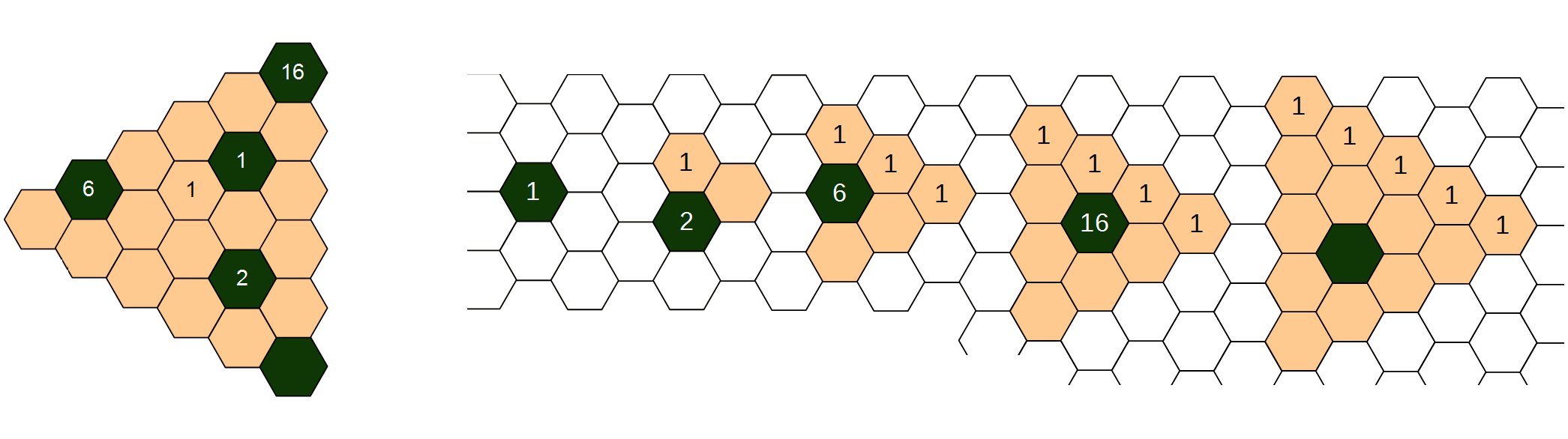
The purest version of the hexagonal concept, marked 12 here, turned out to be too repetitive to be interesting. Version 7 felt like a discovery more than a construction, with it’s beautifully paradoxical harmonization of 6-fold and 7-fold symmetries, as it naturally includes many different local configurations. Version 10 wound up with rounded corners, to avoid unnecessarily many small-scale decisions, and that suggested the final clock format.
Would have been descriptively clean to begin the maze at 1 o’clock and end at 12, but that allowed two very different overall routes, one that was an all-too-regular zig-zag and another much more interesting route that included a sneaky counterclockwise inner loop along with some zig-zags. Both routes had the lucky feature of forcing the hours to be visited in order. Hah, beginning at 12 would allow only the more interesting route.
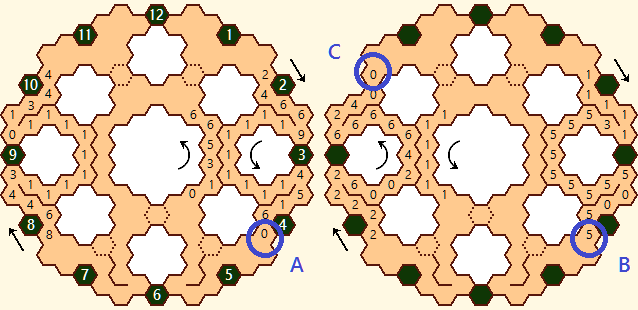
Now to check if this is plain too easy to solve — or, more likely, too tedious, especially as arithmetic mistakes could require redoing a lot of work. A few sloppy failed test solutions were enough to realize that it wasn't too easy and that, surprise, most arithmetic mistakes had only local effects. Some analysis revealed three particularly forgiving lucky features, labeled A, B and C.
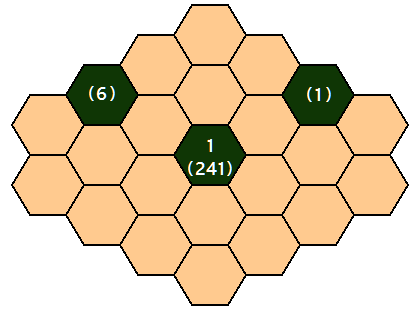
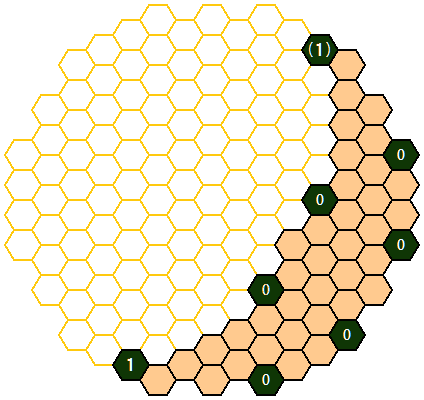
A demonstrates that 2, 3 and 4 o'clock add a combined 0 to the running total. B demonstrates that any sum (or mistake) arriving at 2 or 8 o'clock will be multiplied by 5, which turns all even sums$\scriptsize\raise.2ex/$mistakes into 0 and prepares odd sums$\scriptsize\raise.2ex/$mistakes to become 0 at their inevitable next doubling. C demonstrates that the clock’s inner loop has no net effect on the peripheral loop at 9 o'clock—whew!
More fun than anticipated to analyze and test solve, for hours and hours, this was ready to be posed.