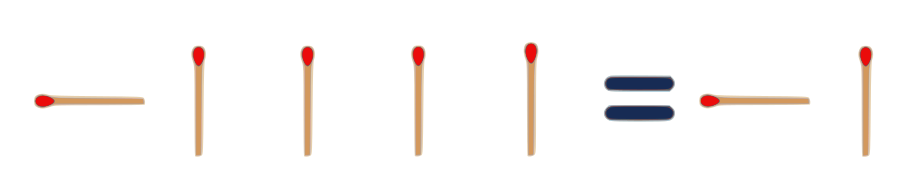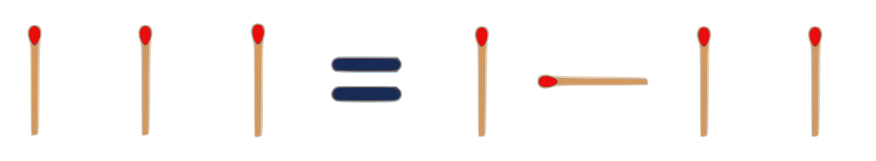Yet another a bit "special" solution, but I would say it is not against the (current) rules, it's just a matter of interpretation.
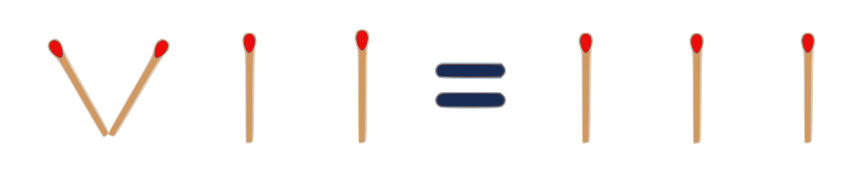
$ VII = 111 $
Roman $7$ = Binary $7$
And another one...
$$ | -11 | = 11 $$ $$ abs(-11) = 11 $$
...and another one...
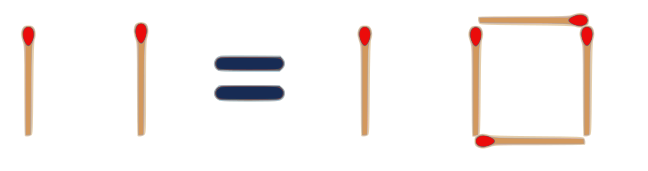
$ II = 10 $
Roman $2$ = Binary $2$
... and another "new" concept...
$$-iiii = -1$$ with the imaginary unit $i^2 = -1$ $$-i*i*i*i = -1$$
...although the "game is over"...
...a new combination from known principles...
Can be interpreted as $|1| = |-1|$ or $|i| = |-i|$ or $|1| = |-i|$ or $|i| = |-1|$
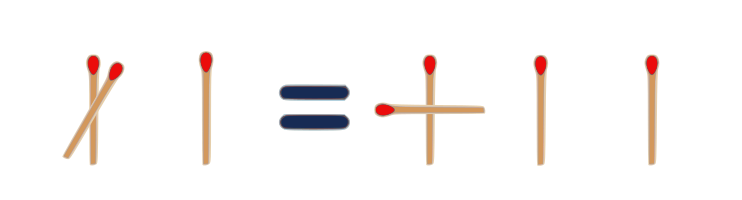
... and a slightly "odd" one ... (maybe I should stop now...)
$ XI = +11 $
Roman $11$ = $+11$
... yet another unconventional "rot90" version...
Roman $2$ = 90° clockwise rotated $2$