Here is a less rigorous but maybe more intuitive answer:
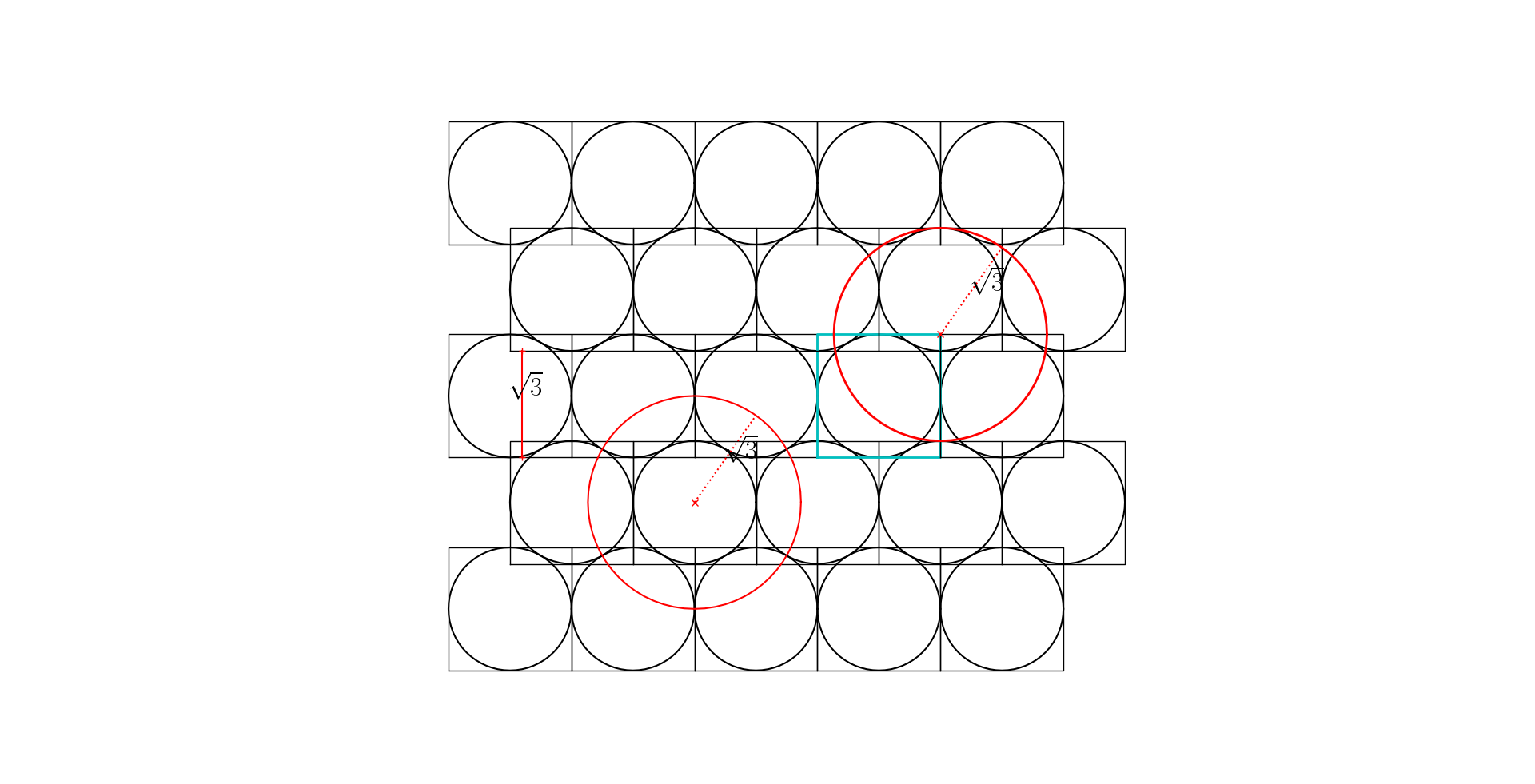
First of all it is easy to see that the small half circles are compatible with a hexagonal packing, so we have the familiar grid with units 1 and $\sqrt 3$. If we insert a circle with radius the larger grid unit and we center it at a small circle center then it is easy to parse the picture.
The puzzle is constructed around an "off center" copy of that circle (fat red circle). What one needs to grasp is that by aligning a side of the circle with the corresponding side of a small circle (top in the visualization) we will also have the center on a corner of a grid square (teal square). 45° symmetry as regards intersections with the small circle in the teal cell follows immediately and from there it is a straight-forward calculation to confirm that the large circle cuts the small one at opposite points.
