Here's a grid of shapes:
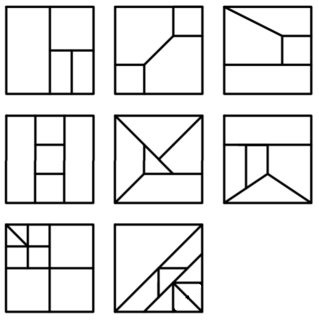
Which of the following shapes should go in the missing cell, and why? There is only one correct answer, and the explanation should not be very complicated.
This is my own puzzle.
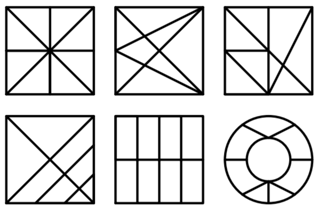
Here's a grid of shapes:

Which of the following shapes should go in the missing cell, and why? There is only one correct answer, and the explanation should not be very complicated.

This is my own puzzle.
The missing shape is
the circle.
Reasoning:
In each row the shapes are topologically equivalent.
More formally:
For any two shapes in a given row, there's a self-homeomorphism on the plane that maps one shape to the other.
From what I can see...
the circle. The first row has 4 outer intersection points and 2 inner intersection points each. The second row has 4 outer intersection points and 4 inner intersection poitns each. the two ont eh third row both have 7 outer intersection points. The first has 4 inner intersection points and the second 5, but that discontinuity goes away if we consider the X in the lower-right-hand corner to be passing by each other and not actually crossing. The circle has 7 outer intersections and 4 inner intersections as well... and is the only one of the options that has an odd number of outer intersection points.
and after the recent edit...
each row is topographically equivalent.
the circle
because
count the number of neighbours each region has. This is the same by row. Equivalently, the number and degree of each vertex, along with the degree of each adjacent vertex, are the same. Corners on the outer border aren't vertices, unless they are connected to a third edge.