Number Guessing (Part 1)

Question

I thought up two positive integers with product less than $500$. I told their product to Penny, and their sum to Sandy, and told both of them the constraints and they are both perfect logicians.

They have the conversation below:

Penny: I don't know what the numbers are, but their sum must be a multiple of $24$.

Sandy: I don't know what the numbers are either.

Penny: I still don't know what the numbers are.

Sandy: I know what the numbers are now!

Penny: Me too! I know what are the numbers now!

What are the numbers I thought up?

The quote above is the problem. Is it hard? I will give out tips if the problem isn't solved yet.

Answer 1

The numbers are:

5 and 19

Step 1:

From being told the product, Penny knows the two numbers sum to a multiple of 24, but doesn't know exactly what they are.
From this information, the only possibilities for the product, and the two numbers, are:
95 (1,95 or 5,19)
119 (1,119 or 7,17)
143 (1,143 or 11,13)
215 (1,215 or 5,43)
287 (1,287 or 7,41)
335 (1,335 or 5,67)
407 (1,407 or 11,37)
455 (1,455 or 5,91 or 7,65 or 13,35)

Step 2

If the sum were any of 120, 144, 216, 288, 336, 408, or 456, Sandy would now exactly know the pair. She doesn't, so the sum must be 24, 48, 72, or 96.

Step 3

If the product were any of 119, 143, 215, 287, 335, or 407, Penny would now exactly know both numbers. She doesn't, so the product must be 95 or 455.

Step 4

If the sum were 96, Sandy would still NOT know the pair with certainty. But she now says she does know, so the sum is not 96. The sum is 24, 48, or 72.

Step 5

If the product were 455, Penny would still not know the pair. But she says she knows, so the product must be 95, and the pair are 5 and 19.

Answer 2

I could get to the second last step. Penny's last statement is logically inconsistent. She cannot say that based on information available to her till that point

Answer 3

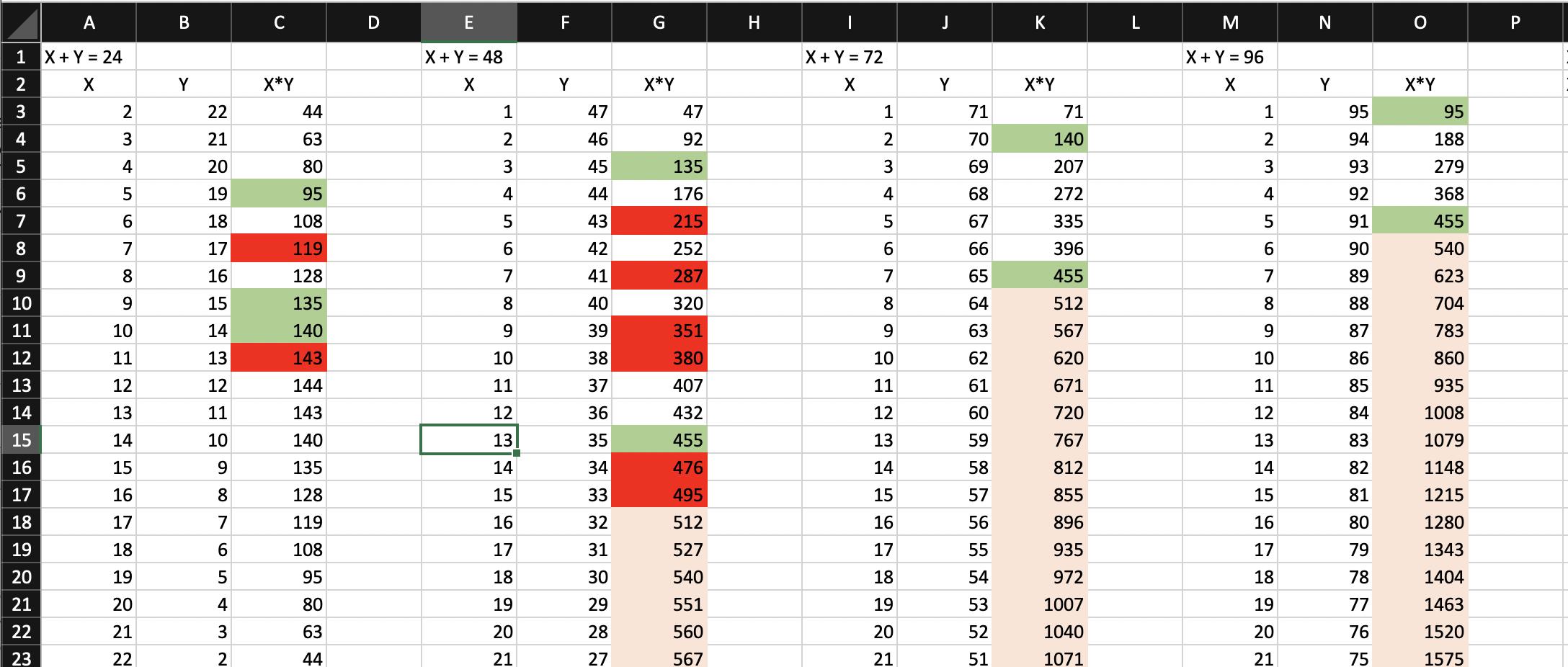
I don't think a definitive answer can be reached, I did see Lanny's answer but I don't agree with the logic above to find (9, 15) as the pair and this is my answer. Lanny's answer does not include 135, pairs being (9, 15) and (3, 45), or 140 with the pairs being (10, 14) and (2, 70). I don't see the reasoning to eliminate these. I think the right logic is to figure out that pairs of products that make it ambiguous for Penny to know what the numbers are, otherwise he would know right away. Sandy can eliminate all sums that only have one product pair correspond to it, because she doesn't know the solution so there must be at least two possibilities for each sum.

Because this leaves only one option for a product like 215 because (1, 215) is the only product pair for the sum 216, (5, 43) gets eliminated too. Several other pairs get eliminated (shown in red in the picture) from this logic. When you come to Sandy being able to say she knows the numbers, there is an issue. Sandy can't know the numbers because there are two pairs for each sum of 24, 48, 72, 96. Sandy can't say she knows the answer yet then, because no matter what sum he knows, there are two viable options, shown in green.

Following that, Penny can't know which pair is correct because each product (95, 135, 140, 455) still has at least two options. Thus, I do not see a logical step forward to eliminate numbers with 135 and 140 pairs factored in. If 135 and 140 were not options, the answer could be solved. Otherwise, I don't think it can with the given information. Thanks for any feedback addressing this.