5
$\begingroup$

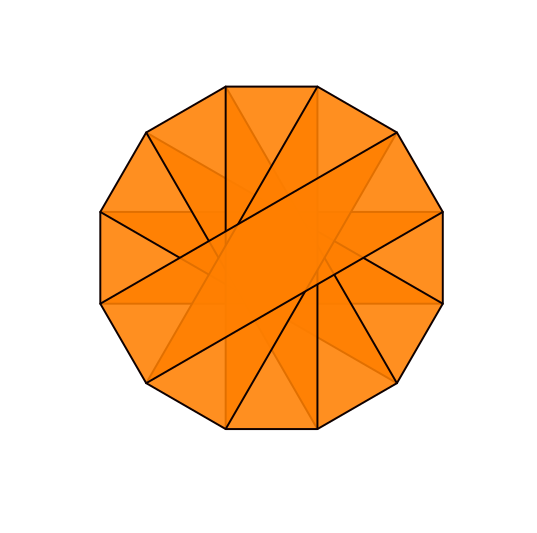
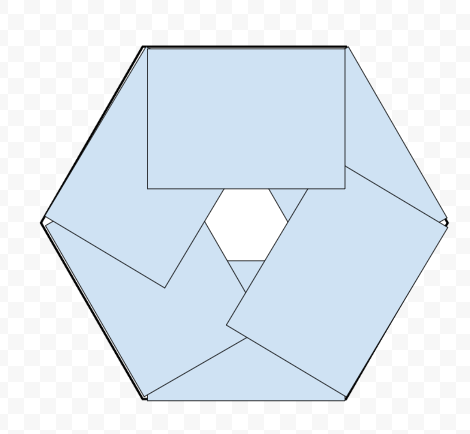
Let's say you have 6 equal business cards.
How can you place them on a table to create these shapes:

  • regular hexagon
  • regular dodecagon

Edit. Feel free to propose an answer for options:
a) you have 50-by-90 units rectangles,
b) the sizes of rectangles doesn't have matter.

Improve this question
$\endgroup$
5
  • 2
    $\begingroup$ What are the dimensions of these cards? $\endgroup$
    – Deusovi ♦
    Commented Mar 20, 2020 at 1:00
  • $\begingroup$ @deusovi, it does not matter, for instance, 90 by 50 mm. $\endgroup$
    – Nick
    Commented Mar 20, 2020 at 1:55
  • $\begingroup$ Do we need to cover the whole interior of the polygon? Or is it enough to create a boundary that has the shape? $\endgroup$
    – sedrick
    Commented Mar 20, 2020 at 2:29
  • $\begingroup$ @sedrick, boudary for 6-gon, and vertecies for 12-gon $\endgroup$
    – Nick
    Commented Mar 20, 2020 at 2:37
  • $\begingroup$ @dooper, could you please add answer with a sketch? $\endgroup$
    – Nick
    Commented Mar 20, 2020 at 3:13

2 Answers 2

Reset to default
13
$\begingroup$

With the right sized rectangles, a hexagon can be made with just three cards, otherwise with 6 cards a hexagon is quite easy.
The dodecagon requires 12 points, so each card has to provide two points to the shape. So half of the cards for the hexagon can simply be 'rotated' 30°.
The ratio for the 'perfect rectangle' for making a hexagon with three cards is 1:√3 enter image description here
With the right sized rectangles, a dodecagon can be filled perfectly too.
enter image description here

Improve this answer
$\endgroup$
6
  • $\begingroup$ Your answer is very good. But how one can define 30°? Also I see the solution in bottom figure includes rectangles which size are not business cards. Could you please give some extensions? $\endgroup$
    – Nick
    Commented Mar 20, 2020 at 7:38
  • 1
    $\begingroup$ @Nick You said in a comment on the question that the size/dimensions doesn't matter. $\endgroup$
    – Rand al'Thor
    Commented Mar 20, 2020 at 20:49
  • 1
    $\begingroup$ The four initial diagrams use cards 90 * 50. The last diagram was more of an extra thing, since it's not business card size. (I think it's about half business card size) $\endgroup$
    – Matthew Jensen
    Commented Mar 20, 2020 at 23:03
  • $\begingroup$ @randal'thor, you are right. In the comment I wrote that the size does not matter because I have the solution for a regular 6-gon and doesn't have the solution for a regular 12-gon. I am omitting the size's requirement. But after the Matthew Jensen's answer I hope that someone can solve the puzzle for business cards too. $\endgroup$
    – Nick
    Commented Mar 21, 2020 at 0:24
  • $\begingroup$ @matthewjensen, if a=50, b=90, then tan(alpha)=a/b. And alpha approximately equals 30 degrees. $\endgroup$
    – Nick
    Commented Mar 21, 2020 at 1:05
2
$\begingroup$

I hopped onto google drawings to make the sketch you asked for. If all you want is the boundary than making a hexagon is very simple. I feel like I am missing some aspect of this...

enter image description here

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ @dooper, how to proof that 6-gon is regular? $\endgroup$
    – Nick
    Commented Mar 20, 2020 at 7:11
  • 2
    $\begingroup$ All sides are equal. This is given because all the cards have the exact same dimensions. To be regular, all that is left is to make all the angles equal. This cannot be proven, since it is possible to construct a hexagon with equal sides but different angles. Thus, it is up to the person constructing this to actually make the angles equal. $\endgroup$
    – Falc
    Commented Mar 20, 2020 at 10:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.