The following sequence has been encrypted:
1-0
3-0
6-1
126-2
373378-2
20532303792-2
24311068187968-3
Can you find the pattern and the next 3 elements?
Hint a - 2nd April 2020
I'm here giving you the next 2 elements. The pattern and the third element remain for your :)
7356612131551389558912-4
651915846947455861534362510912-5
Hint b - 10th April 2020
Factorials are exceedingly used
Hint c - 15th April 2020
I really thought this puzzle will be solved quickly and without hints ^^, it's among the easiest I posted here! Alright, giving you another hint, but it is becoming like... so easy!
At step $n$, there are $n+1$ factorials used!
Hind d - 3rd May 2020
I'm removing the encrypted part of this puzzle with this hint!
The sequence's numbers are:
1
3
60
12600
37337800
2053230379200
24311068187968000
And so on :)
Hint e - 6th May 2020
Giving you an equivalent function ;)
Defining $H(n)$
This puzzle is about $n+1$ factorials so I told myself, why not use Stirling's formula?
$$n! \sim \sqrt{2 \pi n}\left(\frac{n}{e}\right)^n$$
Suppose that $P(n)$ is the sequence we want to understand. $P$ for Puzzle
$P(1) = 1$
$P(2) = 3$
$P(3) = 60$ and so on.
I'm here going to define $H(n)$, $H$ for Hint, using Stirling's formula and such as $P(n)\sim H(n)$ where the sign $\sim$ means that the two quantities are asymptotic: their ratio tends to 1 as n tends to infinity.
Let $T_n = \dfrac{n(n+1)}2$ and $\displaystyle K(n) = \prod_{k=1}^{n}k^k$ ($K$ is the hyperfactorials) I computed for you: $$H(n) = \dfrac{\pi^{\frac{1-n}2}\sqrt{(n+1)}T_n^{T_n}}{\sqrt{(n-1)!}K(n)}$$
Computing $H(n)$
The following Julia script gave me the first elements of $H(n)$
|+--------------------+-------------------------------+
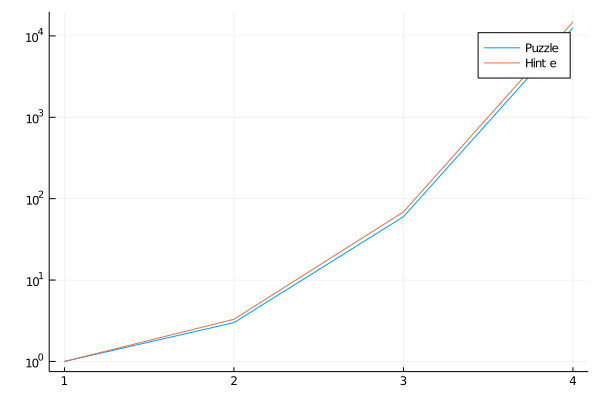
| P(n) | H(n) rounded at one decimal |
|+--------------------+-------------------------------+
| 1 | 1 |
| 3 | 3.3 |
| 60 | 68.8 |
| 12600 | 14823.8 |
| 37337800 | 45388978.9 |
| 2053230379200 | 2501368479610.3 |
| 24311068187968000 | 3000139818683595001.3 |
|+--------------------+-------------------------------+
function T(n)
$~~~~$n*(n+1)/2
end
function K(n)
$~~~~$k = 1
$~~~~$for i in 1:n
$~~~~~~~~$k = k*i^i
$~~~~$end
$~~~~$k
end
function Hint(n)
$~~~~$pi^((1-n)/2)*sqrt(n+1)*(T(n)^T(n))/(sqrt(factorial(n-1))*K(n)sqrt(2)^n)
end
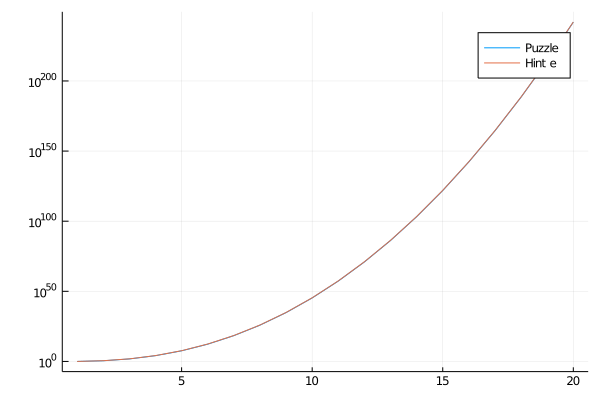
Plots
And I even made some plots for you :)
Warning!
The fact that the definition of $H(n)$ is really (really) complicated doesn't implies $P(n)$ is also complicated! It's even the opposite, $P(n)$ is simple to express. Think of it like the Stirling's formula, $n!$ is really simple to express and $\sqrt{2 \pi n}\left(\frac{n}{e}\right)^n$ is a little less simple to write.
Note
Please note that I've changed the values of my sequence after Hint d to make it even easier :)