2
$\begingroup$

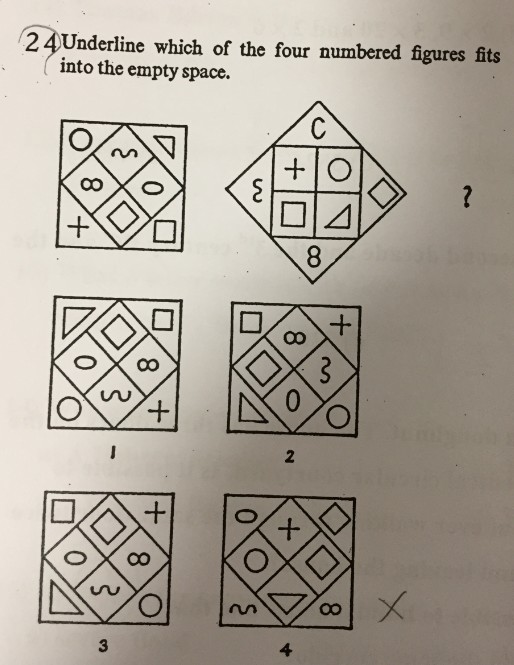
everyone. I encountered this puzzle in a primary school booklet for selective high school exams in Australia. Please help me find out Which of the numbered figures fits into the empty space marked by the question mark, as shown in the picture below. Thanks a lot! enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

I think the answer is probably

enter image description here

Reasoning

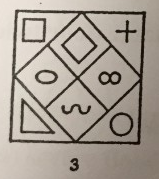
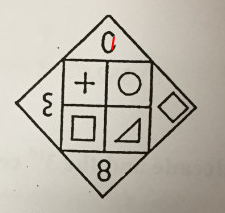
Going from image A to B it looks as though the four symbols on the outside of image A are rotated clockwise 90$^o$ and put into the centre of image B. Similarly, the four symbols at the centre of image A are rotated anti-clockwise 90$^o$ and put on the outside of image B.

The probable next step is to rotate the central symbols of image B 90$^o$ clockwise and put them on the outside of image C and, correspondingly, rotate the outer symbols of image B anti-clockwise and put them in the centre of image C. This corresponds to image 3.

Note here that I have assumed there has been a printing error in image B enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you, hexomino. Your explanation is quite visual and easy to understand but the underlying logic in your solution is essentially the same as the logic in the solution by JMP. I appreciate very much both of your help. $\endgroup$
    – Michael May
    Commented Oct 11, 2019 at 22:30
2
$\begingroup$

3

because:

View the triangle from the right-angle to the hypotenuse. Swap the contents of the triangle with the square on the left of the hypotenuse (i.e. circle/squiggle, plus/8, triangle/oval, square/square). Rotate the outer square (and the inner square) $45^\circ$ anti-clockwise, and then just the inner square $90^\circ$ clockwise. This gives stage 2.

Then:

Swap the contents of the triangles with the contents of the squares on the right of the hypotenuse, rotate the outer + inner square $45^\circ$ clockwise, and the inner square alone $90^\circ$ anti-clockwise. You get 3.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you, JMP, for your solution and explanation which may be reasonable but may also be a little difficult to understand for me. I can't figure out what you were referring to in your first sentence. Would you please to further clarify that? Thanks a lot. $\endgroup$
    – Michael May
    Commented Oct 11, 2019 at 22:28

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.