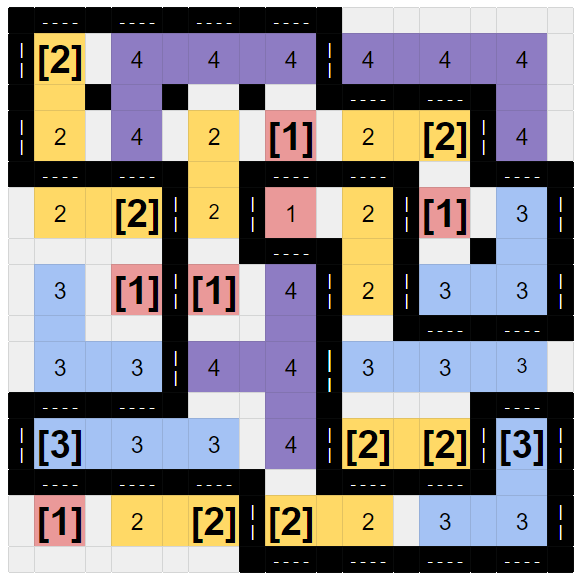
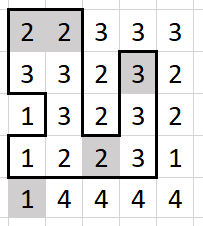
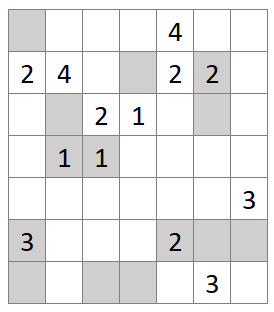
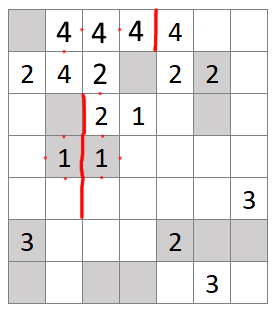
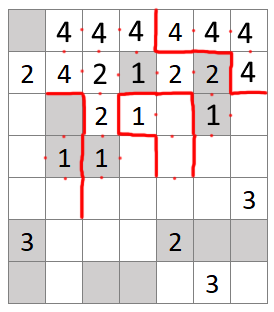
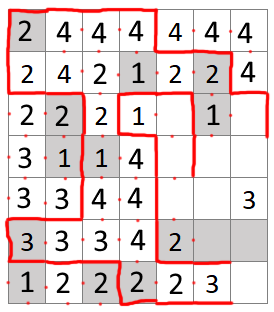
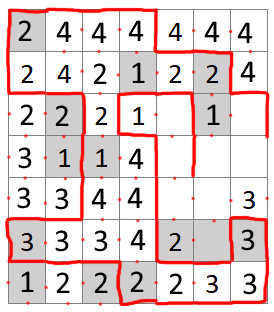
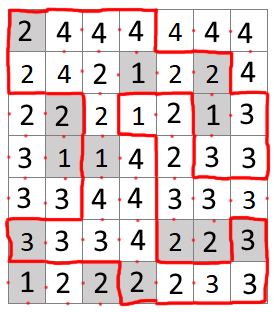
In this answer, I use "RxCy" for the cell in the x-th row (from the top) and the y-th column (from the left).
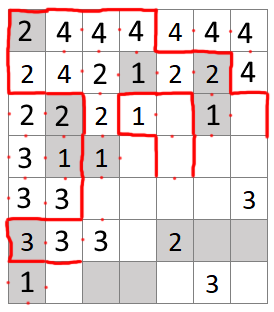
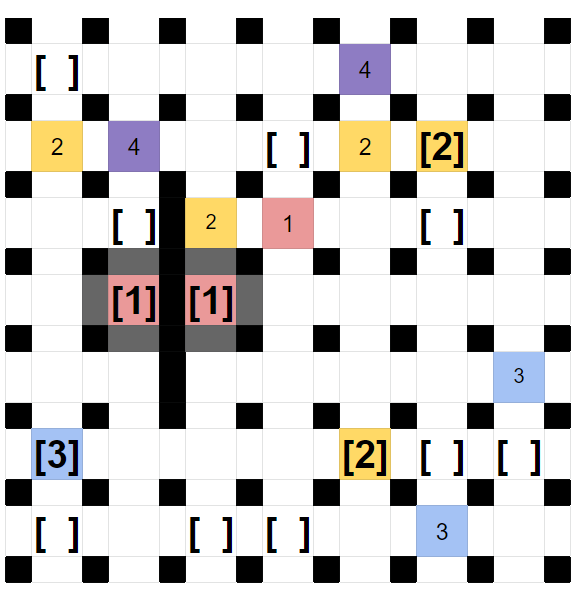
First:
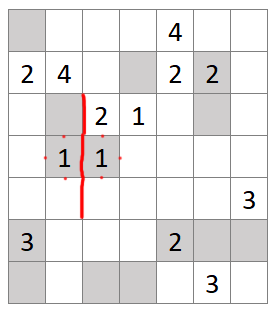
The two 1s must be separated by the loop. Since they're both Slitherlink clues, this gives a few loop segments:
Second:
The 2 in R3C3 must extend upwards. Then the 4 in R2C2 must extend upwards and to the right (because it cannot use any Slitherlink clue cells); this gives another loop segment to the right of that, because otherwise it would touch the other 4 clue.
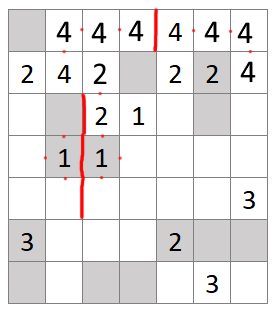
Then, the other 4 clue can be extended right and downwards.
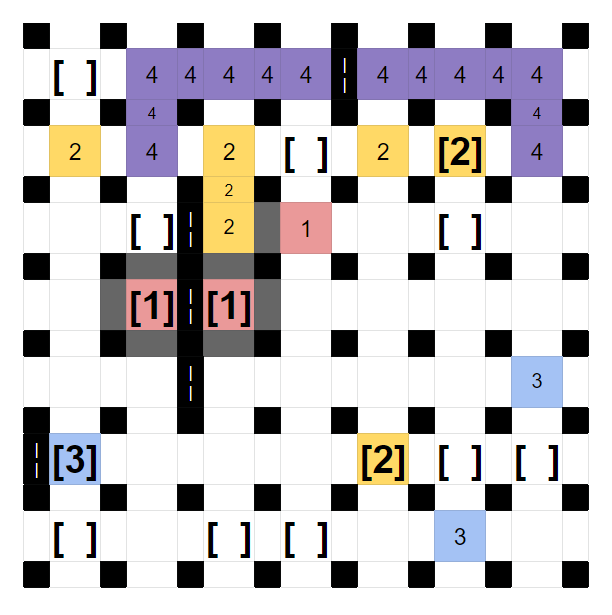
Following directly from that,
take a look at the 2 in R2C6. To not leave an endpoint stranded in the top right, that 2 needs to have its edges at either the top and right, or the bottom and left. But if the bottom and left are used, that traps the Fillomino region. So it must be the top and right, and therefore that Fillomino region extends leftwards.

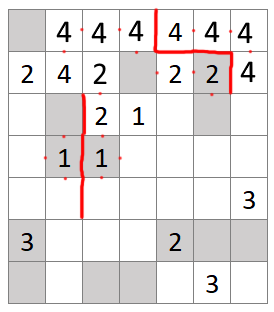
And then:
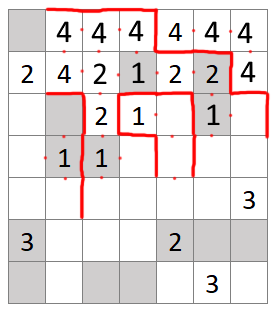
The cell in R2C4 must be a 1, since it cannot be part of any of the 4 groups around it. This gives a couple more loop segments - specifically, the endpoint above the 1s from the start must go leftwards. This tells us that the unknown clue in R2C3 is at least a 2, and so it extends left in Fillomino. And this tells us that the clue is exactly 2, and so we can resolve the top left area.
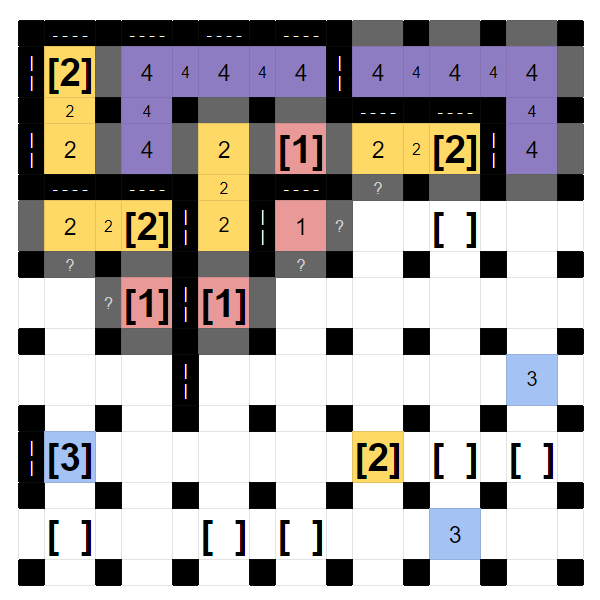
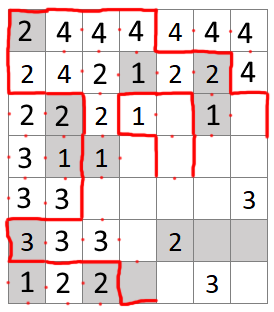
Now that the top left is resolved,
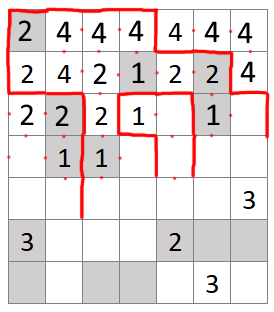
which way can the 3 in R6C1 extend? It can't go upwards, since the left endpoint would be trapped. It can't go downwards, since the clue below would be a 3 as well, and that would force a tiny loop in the bottom left. So it must go right. And then R4C1 must be a 3 as well.
Also, the segment near the 1 in R3C4 can be extended as well (with two more regions partially decided).

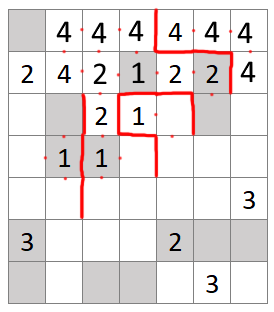
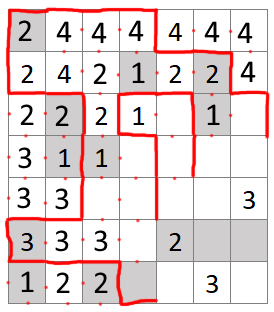
Now we move to the top right:
The clue in R3C6 cannot be a 2, because it touches a 2. It cannot be a 3, because that would make a U shape, blocking off the Fillomino region. So it must be a 1, giving more of the loop.
Then, the B region cannot extend to the right, because it would complete a small loop in the center. So it must extend downwards.
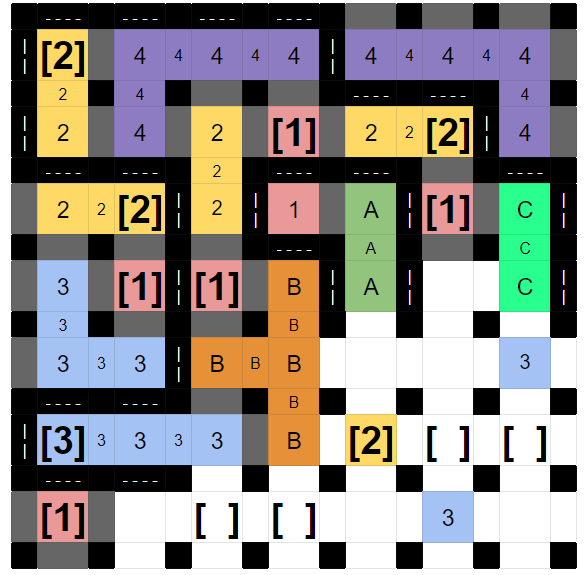
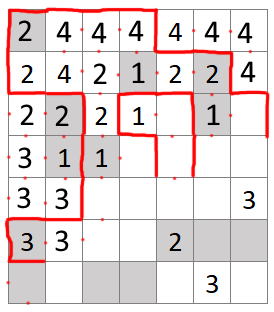
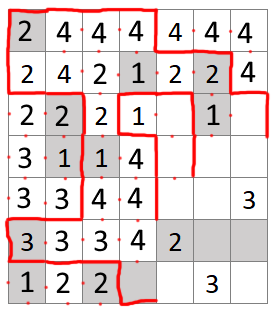
Finally, we've reached the bottom!
What can the clue in R7C3 be? It can't be a 3, since it would trap an endpoint in the bottom left. It can't be a 1, because R7C2 would be unfillable. So it must be a 2. By similar logic, so must R7C4, and that forces B to be 4.
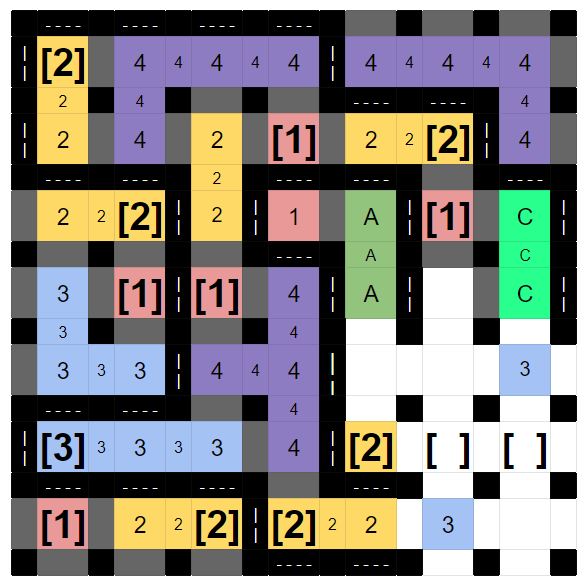
And finally:
The 2 in R6C5 cannot go upwards, since it would force region A to also be 2. So it must go right. This pushes the 3 in R7C6 right and up, and lets us complete the loop in the bottom right. Finally, the rest of the loop can be finished, and the other regions can be resolved.
The solution: