8
$\begingroup$

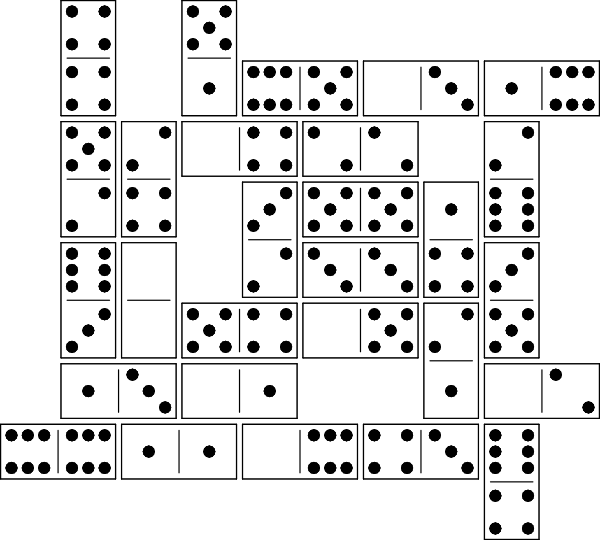
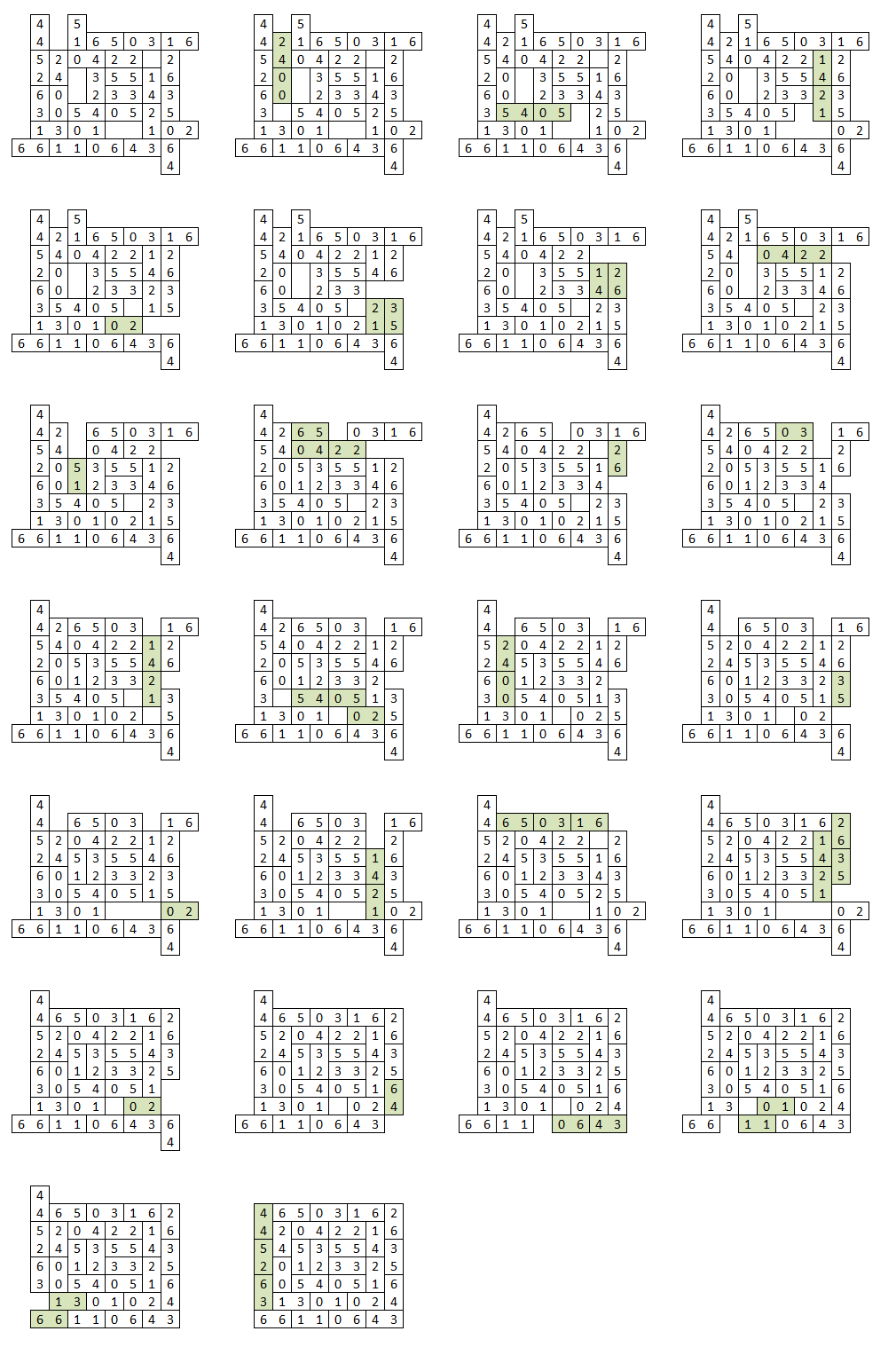
I've designed a set of dominoes puzzles that I call Donimoes. You slide the dominoes like the cars in Nob Yoshigahara's Rush Hour puzzle, always along their long axis. The goal is to slide all the dominoes into a rectangle, without sliding any matching numbers next to each other. See Monday's problem for complete rules and and an example solution. Tuesday's, Wednesday's, and Thursday's problems would make a good warmup.

Today's problem is the grand finale, using a complete set of double-six dominoes! Good luck, and post your solution as an answer.

starting layout

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

This was tricky! (The top right corner is particularly difficult to manoeuvre, avoiding putting the 2's and 1's together. The 0's and 1's at the bottom also pose similar problems...)

A winning set of moves is as follows (move sets in brackets can be carried out in more than one order):

24U, 00U, [54L, 05L, 14U, 21U,] 02L, 02L, 02L, [21D, 35D, 14D, 26D,] 22R, 04R, 51D, 51D, 51D, [04L, 65L, 22L,] 26U, 03L, 14U, 21U, 02R, 05R, 54R, 00D, 24D, 35U, 02R, 02R, 21D, 14D, 65L, 03L, 16L, 16L, [26U, 35U, 14U, 21U,] 02L, 02L, 64U, 64U, 43R, 06R, 01R, 11R, 66R, 13R, 63D, 52D, 44D.

In diagram form (read along each row):

enter image description here

56 moves in total!

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Congratulations, @Stiv! Thanks to everyone who tried out my Donimoes puzzles this week. $\endgroup$
    – Don Kirkby
    Commented Jul 27, 2019 at 19:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.