$\begin{array}{ccc}6&7&4\\ 4&11&14\\ 13&2&11\\ 6&8&1\\ 15&3&?\end{array}$
Own attempt at building a puzzle, find the missing number.
I divided the table into three columns and summed up the cross sums of their entries. This results in 26 for the first column and 22 for the second one.
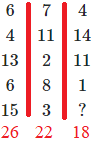
The difference between those is 4, so maybe it should be 4 for the next difference, too. Thus, column three should have a cross sum sum of 18.
? = 6
I think it's unlikely that this is really the solution, since you could make up several similar ways of "solving" the puzzle, but maybe it's worth a try.
Here's the closest I get
13
Reasoning
if we sum the numbers in an X Formation, they turn out to be equal. Like: \begin{array}{cccccc}6&&7&&4\\& + &&& \\4&&11&&14\\&&& + & \\13&&2&&11 & =28\\\\6&&8&&1\\\\15&&3&&?\end{array}- \begin{array}{cccccc}&6&&7&&4\\&&&&+& \\&4&&11&&14\\&&+&&&\\28 = &13&&2&&11\\\\&6&&8&&1\\\\&15&&3&&?\end{array} and if we use the same rule on the lower X formation like this \begin{array}{cccccc}&6&&7&&4\\\\&4&&11&&14\\\\&13&&2&&11&\\&&&&+&\\&6&&8&&1\\&&+&&&\\34 =&15&&3&&?\end{array}- \begin{array}{cccccc}6&&7&&4\\\\4&&11&&14\\\\13&&2&&11&\\&+&&&\\6&&8&&1\\&&&+&\\15&&3&&?& = 34 \end{array} so "?" should be 13 to maintain this rule
6+4+13+6=29
7+11+2+8=28
4+14+11+1=30
30-29=1
29-28=1
27-15=12
15-3=12
The answer is 27