15
$\begingroup$

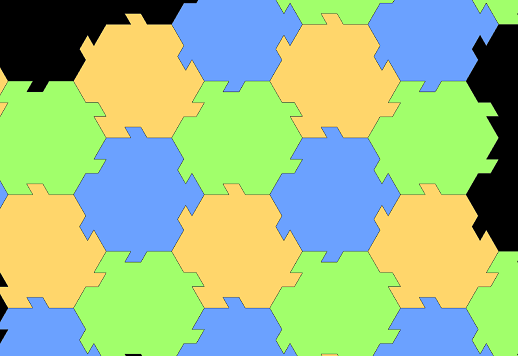
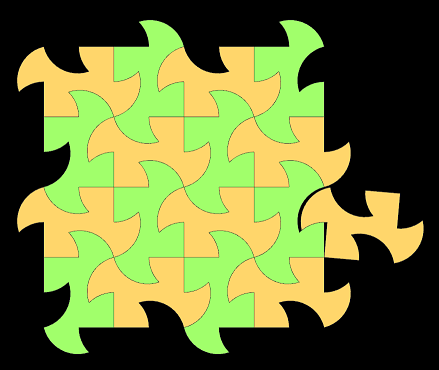
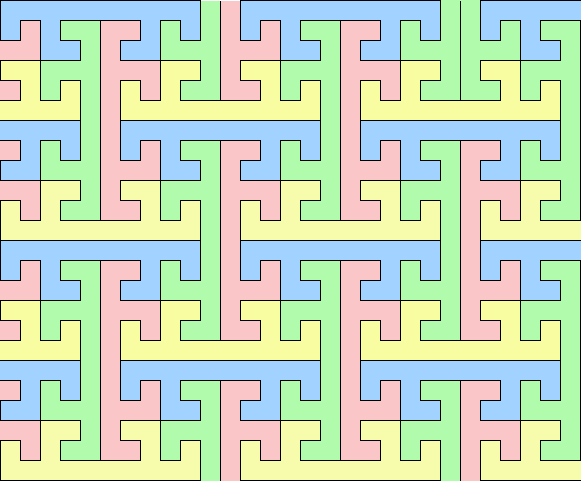
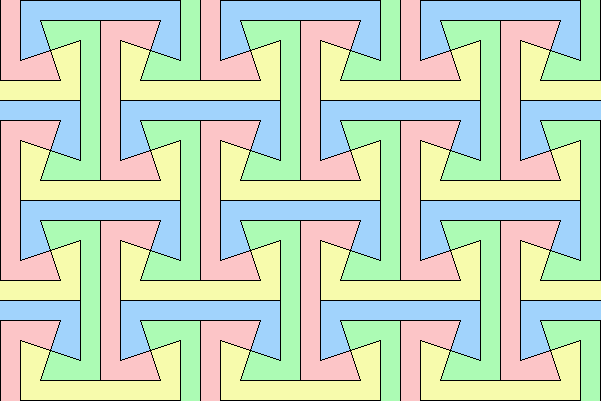
Assume you have a jigsaw puzzle that is also a tessellation. This means every piece has an identical shape and can be assembled into a 2D pattern that fills the plane with no gaps. Such a jigsaw puzzle might look like this:

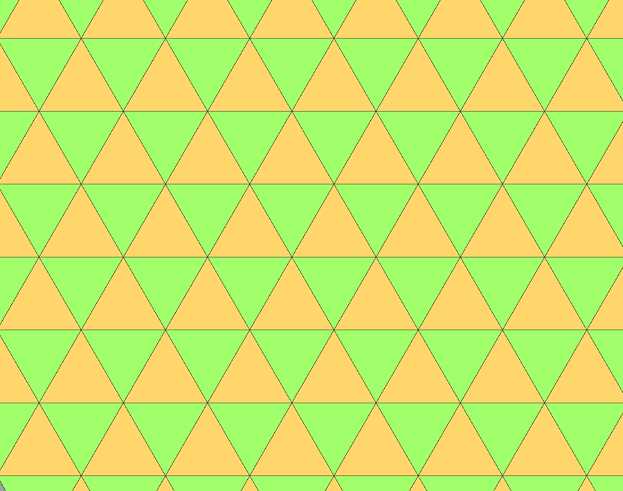
enter image description here

Or, by a weak definition, even this:

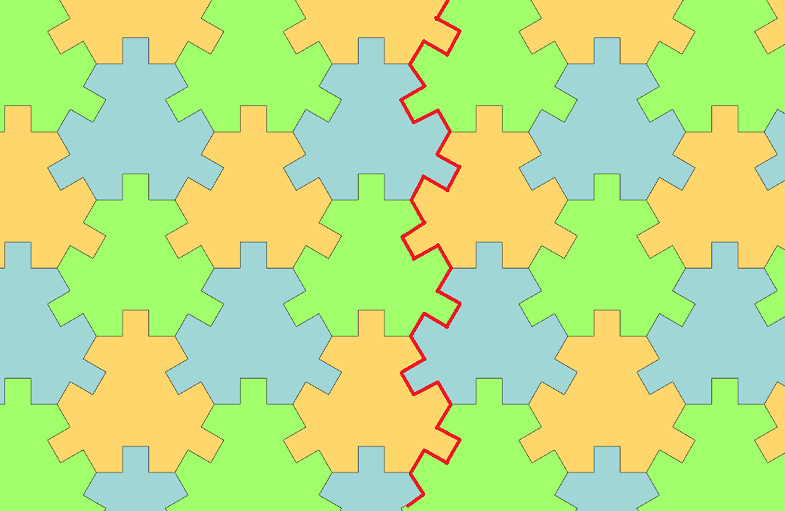
enter image description here

Now most people don’t recognize that triangle grid to be a “jigsaw puzzle” because none of the pieces are interlocking. Before anybody starts trying to create a definition for what geometries constitute “interlocking”, let’s take a look at a third example:

enter image description here

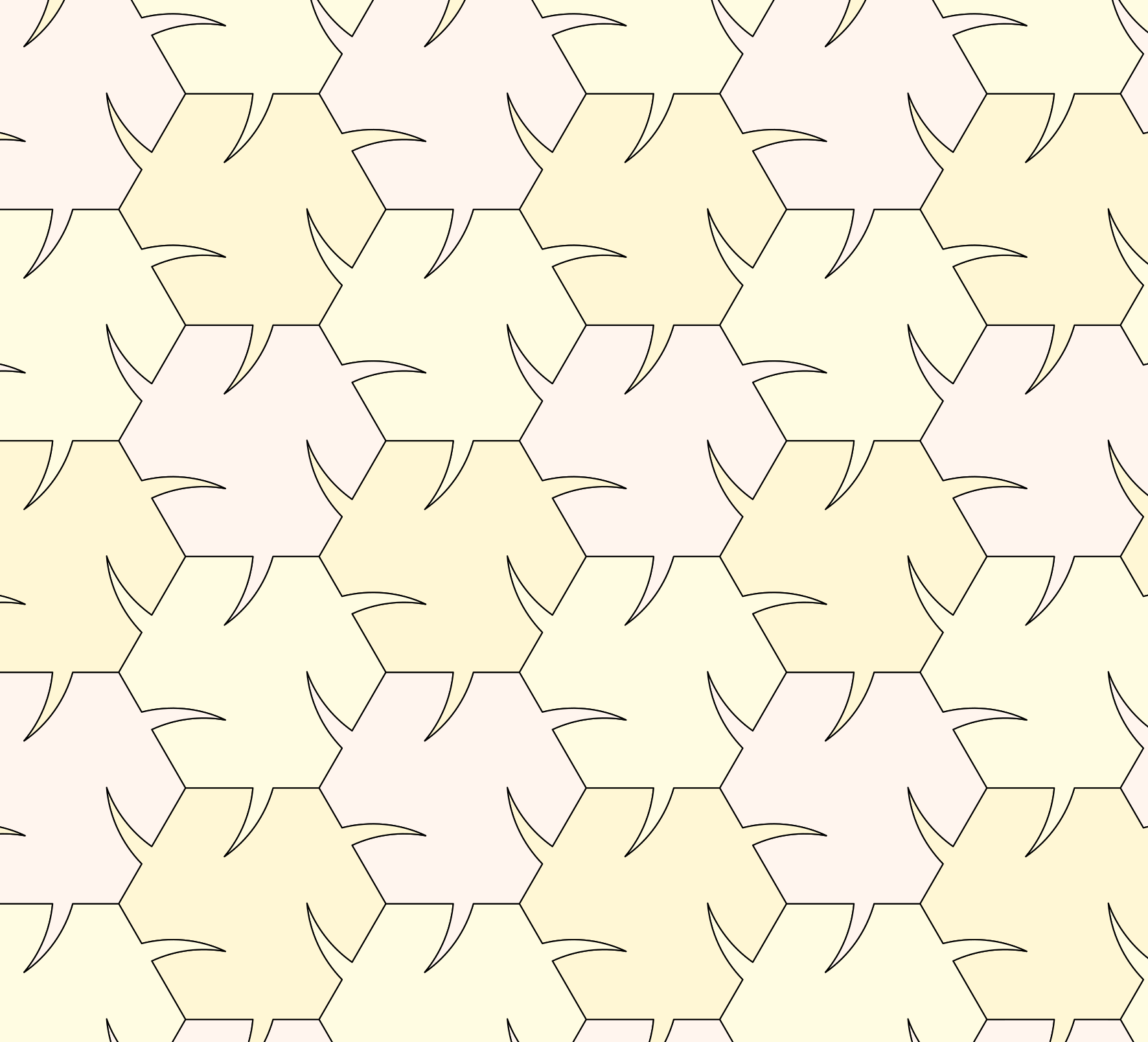
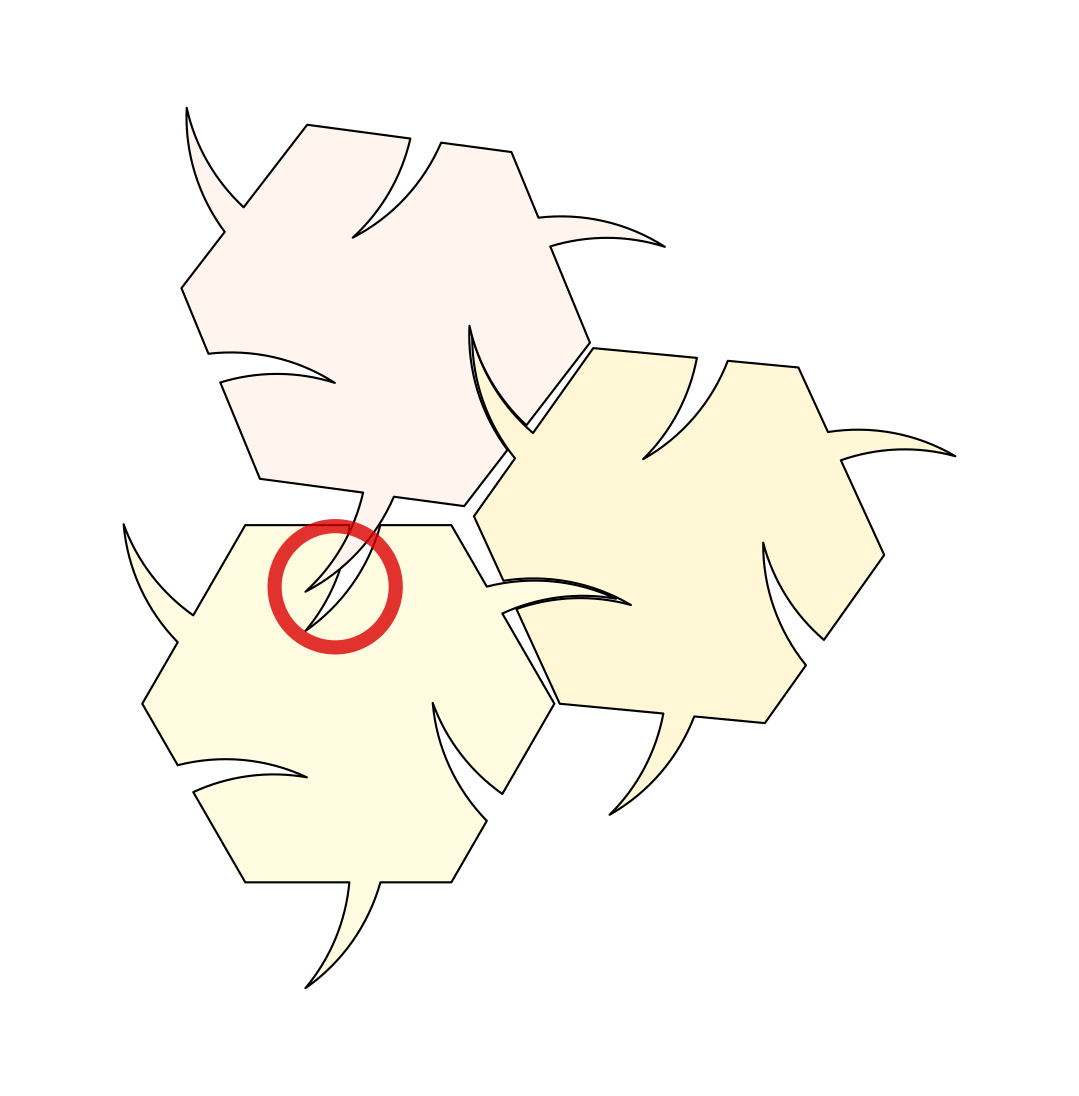
You will first note that any two adjacent pieces do not actually lock together, they can be simply slid together or apart. Now look at the red “fault line” I drew in the overall pattern. If I try to separate the puzzle along that line, you will notice that pieces zig-zag enough where they are locked together and won’t move apart. You can, however, still pull this puzzle apart.

The teaser question:

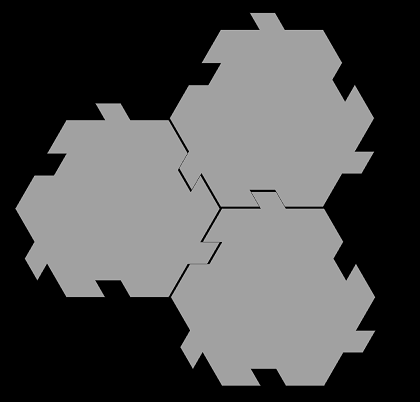
How can you pull apart the above puzzle? The pieces must stay on the plane (or table, I suppose) and for sake of simplicity, let's just assume there is one piece in the center and it has 6 adjacent neighbors

But the real question is:

Can you design a single shape that satisfies the following:

It can be tessellated (cover an infinite plane with no gaps)

Any two adjacent pieces are not interlocking by themselves

By assembling some number of pieces together (beyond two), the pieces become interlocking and thus cannot be slid apart in any way

This is intended to be a real-life question, but the math needs to check out too.

ALSO: I used CAD software when I designed this problem. I'm assuming most of you don't have that, so I'll try and help people out by providing feedback. More than once I thought I had a good idea that didn't pan out so don't feel bad if I tell you you're wrong. Have fun!

This question has been answered by @Florian F but there are other approaches that could solve it. I came up with two and neither were like that one. I'll maybe post mine eventually but for now anybody can keep trying. (I've added the open-ended tag)

Improve this question
$\endgroup$
2
  • $\begingroup$ is the third example the answer to the question then? $\endgroup$
    – Omega Krypton
    Commented May 18, 2019 at 3:51
  • $\begingroup$ The teaser question has been answered now, so if you want to see why the third example can be disassembled, see below. That is the reason it cannot be the answer to the bigger question. $\endgroup$
    – Skosh
    Commented May 18, 2019 at 11:21

6 Answers 6

Reset to default
13
$\begingroup$

To a real-life problem I had to give a real-life answer:

enter image description here

But you asked for an actual tiling, without gaps, so here it is.

enter image description here

PS: there is a simpler pattern where pairs disassemble with a single translation:

enter image description here

Improve this answer
$\endgroup$
6
  • 4
    $\begingroup$ Great pattern! Where is that? I never actually found evidence of an existing pattern that solved this puzzle. Anyway, this pattern works by forcing the pieces to assemble using two translations. Most single-translation assemblies don't work, even ones at a funny angle, which is why this problem can be hard to solve. $\endgroup$
    – Skosh
    Commented May 19, 2019 at 13:31
  • $\begingroup$ @DarkThunder what's yours then? Florian F: +1 in admiration! $\endgroup$
    – Omega Krypton
    Commented May 19, 2019 at 13:43
  • 1
    $\begingroup$ may i ask where is the real life pic taken? $\endgroup$
    – Omega Krypton
    Commented May 19, 2019 at 13:44
  • $\begingroup$ The picture was taken in Japan, I don't know the exact place. If I remember well it was a documentary about Fukushima. I made a screenshot because I liked the structure. $\endgroup$
    – Florian F
    Commented May 19, 2019 at 13:51
  • $\begingroup$ Oh, nice edit! My single translation solution needed to use a complicated Escher-inspired tiling. I'm gonna make a patio using one of your patterns, though, they are much cleaner. @Omega Krypton I'll post something in a few days, I'm hoping more answers might come forward before then. $\endgroup$
    – Skosh
    Commented May 19, 2019 at 16:32
6
$\begingroup$

This is just for the teaser question (do not know the answer for the real one). I think you can pull the puzzle apart by

simultaneously pulling all six pieces surrounding the center. Each piece moves along the vector connecting the center of the middle tile with the center of the moving tile. Something like this:

enter image description here

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ is it possible to pull the six pieces apart at the same time, since they are interlocking each other? nevertheless, have an upvote! $\endgroup$
    – Omega Krypton
    Commented May 18, 2019 at 10:31
  • 2
    $\begingroup$ Yes, this is the answer to the teaser. I call it the "expanding universe" technique because every piece moves away from every neighbor at the same rate. This is what we observe distant galaxies doing, and might help you visualize why things far away enough end up moving away faster than the speed of light $\endgroup$
    – Skosh
    Commented May 18, 2019 at 10:46
6
$\begingroup$

Although I was too lazy to get the angles exact, the idea should hold in principle if the picture isn't quite right.

Tiling

They interlock.

Cannot be separated due to rotation

Improve this answer
$\endgroup$
1
  • $\begingroup$ Awesome! Yeah this was one of the two ideas I had, using rotation instead of translation. It looks like you have the smaller arc centered on the hexagon corner which probably isn't necessary but that's what I had so I'm betting this works perfectly as is. Great pictures, too. $\endgroup$
    – Skosh
    Commented May 19, 2019 at 19:48
4
$\begingroup$

Florian F's 2nd pattern is far and away my favorite, but if anyone was curious, I'll post my answers.

First I wanted to show an example of something that doesn't work but really seems like it should:

![enter image description here]

It's just like the third example from the question, but it uses joinery such that the pieces slide together at an angle. It comes apart in the same way, though, each piece spinning slightly in place to account for that angle connection. You can fix this, however, by disrupting the piece's rotational symmetry.

![enter image description here

This shape still tessellates, and I knew that thanks to M.C. Escher. I never found a pattern he did that happened to solve my question exactly, but his "Reptiles" takes advantage of a similar tessellation. My other solution, that I was very happy to see Veedrac come up with, was the idea of using rotation instead of translation to bring the pieces together.

![enter image description here

Sliding the pieces together requires them to touch at a corner and then rotate in. There's better ways to constrain the rotation but the shark fin still works and it looks cool. I've never seen anything quite like that before but I'm guessing that's just because nobody had a reason to want it until now.

Improve this answer
$\endgroup$
3
$\begingroup$

How about the

Voderberg tiling?

Any two adjacent pieces can be slid apart, but I don't think the whole thing can be split by sliding from any direction.

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ Quite interesting, I've never seen this before. However, "infinity" can't be the reason that the puzzle holds together. Any real version of this tiling is finite and can be slid apart, starting at the outside and working your way in. $\endgroup$
    – Skosh
    Commented May 18, 2019 at 15:50
  • 1
    $\begingroup$ I believe you can separate the tiling in 2 along a roughly horizontal fracture line. $\endgroup$
    – Florian F
    Commented Nov 29, 2020 at 15:22
0
$\begingroup$

Maybe this works?

enter image description here

Note: I am using MS Excel to finish this. The grids are not perfect squares, so they, when analysed using this image, may not be accurately identical. Yet I hope you all get the idea. Thanks!

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ I could be mistaken, but I guess we may slid apart gold-gray-gold-gray with their left side (orange-darkblue-orange-darkblue) $\endgroup$
    – athin
    Commented May 18, 2019 at 3:13
  • $\begingroup$ The yellow/orange pieces can be separated from the blue/gray pieces by a simple vertical push. I like the pattern, though, I might have that in my sock drawer. $\endgroup$
    – Skosh
    Commented May 18, 2019 at 10:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.