4
$\begingroup$

I have decided to make a little bit of theme for this next chess question. And that theme is foxholes in war. Makes sense to me since chess is a technically a war-themed game.

I have two puzzles for you centralizing around this theme. They are both different.

Puzzle 1

Assuming that both sides cooperate, find a mate in 29 from this position.

It is white to move first, and black to checkmate white.

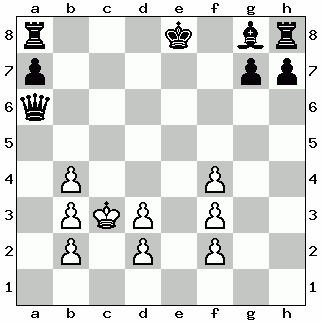
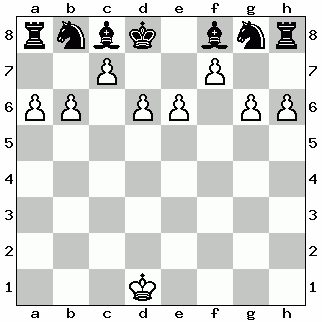
enter image description here

Restrictions:

  1. Black only has two pieces left at the end of the puzzle.
  2. All black pawns promote
  3. Black is never checked
  4. The white king gets checkmated on d4
  5. White pawns do not promote at all
  6. White pawns never move straight

Puzzle 2

Construct a 28 move game, with white checkmating black on their last move, ending in this position.

enter image description here

You shall be given this information:

  1. No white rooks, knights, or bishops, along with no black rooks or bishops, can be moved at all.

  2. Only one black knight can move, and it must move exactly twice.

  3. All black pawns make the same move throughout the entire game.

  4. All white pawns make the same move throughout the entire game. Other moves they make are the same as the black pawns, but here we focus on another pawn move they can make. Black does not make this one. (CLARIFICATION: For those who do not understand this, this is about how pawns move. See here if you still don’t understand.)

  5. White pawns must be doubled exactly four times.

You may do one or both puzzles. It is optional. Good luck!

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ GIF might be hard to analyze, IMO $\endgroup$
    – Brandon_J
    Commented Apr 3, 2019 at 23:40
  • 2
    $\begingroup$ Well, the rest of the puzzle was understandable to me, so that's why I was confused. $\endgroup$
    – Brandon_J
    Commented Apr 3, 2019 at 23:46
  • 5
    $\begingroup$ @RewanDemontay Your clarification didn't make sense. Also, I'm not exactly a fan that we "have to" use Apronus. I personally think it's easier to post the PGN $\endgroup$
    – Prince North Læraðr
    Commented Apr 4, 2019 at 0:24
  • 3
    $\begingroup$ OK, the whole apronus thing has been nagging at me for a while. I think I've come to the following conclusion: If you want to use apronus (or any particular site), that's cool, no reason necessary. If you want us to use a particular site, I think that's when we deserve an explanation. Also, I still don't understand you clarification. $\endgroup$
    – Brandon_J
    Commented Apr 4, 2019 at 0:58
  • 1
    $\begingroup$ Puzzle #2 can be presented on apronus in the following way bit.ly/2uVX2CN --- (1) it states the problem, (2) it shows the solution when necessary, (3) it can be used to record an alternative solution, all in one interface. Let me know if this tool needs any improvement because I am the author. $\endgroup$
    – user58390
    Commented Apr 5, 2019 at 12:52

2 Answers 2

Reset to default
3
$\begingroup$

Attempt 4 (#1)

I think I finally got it:

1. Kd4 Qc6 2. Ke3 Qc3 3. dxc3 a5 4. Kd4 a4 5. Ke3 a3 6. Kd4 a2 7. Ke3 a1=Q 8. Kd4 Qa3 9. bxa3 Rc8 10. Ke3 Rc4 11. bxc4 Bd5 12. Kd4 Be4 13. dxe4 g5 14. Ke3 g4 15. Kd4 g3 16. Ke3 g2 17. Kd4 g1=Q 18. Ke3 Qe1+ 19. Kd4 Qe3+ 20. fxe3 h5 21. Kd3 h4 22. Kd4 h3 23. Kd3 h2 24. Kd4 h1=Q 25. Kd3 Rg8 26. Kd4 Rg4 27. fxg4 Kd7 28. Kd3 Kd6 29. Kd4 Qd1#

I guess here the foxhole is the columns of white pawns that were meant to protect the king, until one of black’s pieces came from behind and did a sneak attack. Also, I still think there’s a shorter solution that satisfies all the requirements, but for now this’ll do.

Attempt 3

For #2, is this what you were looking for?

1. d4 Nh6 2. d5 c5 3. dxc6 Qc7 4. b4 Qf4 5. b5 a5 6. bxa6 Qxc1 7. f4 Qxb1 8. f5 e5 9. fxe6 Qxa1 10. h4 Qd4 11. h5 g5 12. hxg6 Qxg1 13. a4 Qxh1 14. a5 b5 15. axb6 Qxf1+ 16. Kd2 Qxd1+ 17. Kxd1 Ng8 18. c4 Kd8 19. c5 d5 20. cxd6 Ke8 21. e4 Kd8 22. e5 f5 23. exf6 Ke8 24. g4 Kd8 25. g5 h5 26. gxh6 Ke8 27. f7+ Kd8 28. c7# (Apronus link)

All the white pawns do en passants to capture the black pawns, which is the “additional move” that black doesn’t get to do, since black’s pawns never escape the 5th rank. I’m not sure how the foxhole/war theme fits this puzzle, however...

As for #1, I’m convinced that it’s too broad without additional restrictions, because

I found a shorter solution that ends the same as my previous attempt and the black king never gets into check:

1. f5 Kd7 2. f4 Qg6 3. fxg6 Bf7 4. gxf7 Kc6 5. f8=B Kb5 6. Bxg7 a5 7. Bxh8 Re8 8. f5 Ka6 9. f6 Kb5 10. f7 Ka6 11. fxe8=B h5 12. Bxh5 Kb5 13. Bd1 Ka6 14. Bd4 Kb5 15. Bc2 axb4#

and I’m pretty sure I can find at least two more “solutions” using the same theme

Attempt 2

OK, second try for #1:

I can deliver selfhelpmate in 18 moves:

1. f5 Qe6 2. fxe6 Bf7 3. exf7+ Kd7 4. f8=B Kc6 5. Bxg7 Kb5 6. Bxh8 a5 7. Bd4 Re8 8. f4 Rf8 9. f5 Re8 10. f6 Rf8 11. f7 Re8 12. fxe8=B+ Ka6 13. Bg6 Kb5 14. Bxh7 Ka6 15. Be4 Kb5 16. Bf3 Ka6 17. Bd1 Kb5 18. Bc2 axb4# (No Apronus link unfortunately because I couldn’t get it to underpromote the pawns)

Again, if 29 moves have to be made, we can probably modify the sequence so that the final Kb5 occurs on an even move, then just simply stall around until move 28, whereby you play 29. Bc2 axb4#. And I think this solution fits with the theme of failed foxholes, since the white king is surrounded by his own pieces in a sort of defensive scheme, but ultimately it doesn’t protect him from checkmate. Perhaps this could be even better if the extra pawn promoted and smothered the king some more...

And second try for #2:

1. a4 a5 2. b4 c5 3. c4 Qc7 4. d4 Qf4 5. e4 Qxc1 6. f4 e5 7. g4 g5 8. h4 Qxb1 9. bxa5 Qxa1 10. dxc5 Qd4 11. fxe5 Qxg1 12. hxg5 b5 13. axb6 d5 14. cxd6 f5 15. exf6 h5 16. gxh6 Qxh1 17. g5 Qxf1+ 18. Kd2 Qxd1+ 19. Kxd1 Nd7 20. a5 Nb8 21. c5 Kd8 22. e5 Ke8 23. g6 Kd8 24. e6 Ke8 25. a6 Kd8 26. c6 Ke8 27. f7+ Kd8 28. c7# (Apronus link)

I’m still terribly confused by the pawn move rule, so this solution includes en passant moves by white only, in addition to two squares forward, one square forward, and capture moves.

Original answer

For #1, if both sides are cooperating,

we can simply cut to the chase and do a 1-move mate: 1. d4 Rc8#. If you insist on 29 moves, we can simply stall with white king moves for 28 turns to reach the original position, then do 29. d4 Rc8# as before. I’m not too sure how the “foxhole” theme fits in to this, so perhaps there’s a more thematic solution that I’m missing.

For #2, I believe this satisfies all the constraints:

1. a4 a5 2. b4 b5 3. c4 c5 4. d4 Qc7 5. e4 Qf4 6. g4 Qxc1 7. f4 d5 8. h4 e5 9. bxa5 f5 10. axb5 g5 11. dxc5 h5 12. cxd5 Qxb1 13. fxe5 Qxa1 14. exf5 Qd4 15. hxg5 Qxg1 16. gxh5 Qxh1 17. a6 Qxf1+ 18. Kd2 Qxd1+ 19. Kxd1 Nd7 20. b6 Nb8 21. c6 Ne7 22. d6 Ng8 23. e6 Kd8 24. f6 Rh7 25. g6 Rh8 26. h6 Ra7 27. f7 Ra8 28. c7# (Apronus link here)

I still think the pawn move constraint needs to be clarified, since we need all 28 moves to reach the pawn setup required. The knight move constraint confused me as well: do you mean we can only move the black knights twice total or twice per piece? And is there a similar constraint on the other pieces as well?

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ @RewanDemontay Honestly, the pawn move rule just makes absolutely no sense. Are the moves you are talking about captures? En passants? Like I said, we need all 28 moves to get the pawns in the positions needed, and there can’t be any delays. As for the first puzzle, I have a feeling that it’s going to be too broad without additional restrictions, but I’ll try again $\endgroup$
    – HTM
    Commented Apr 5, 2019 at 5:46
  • 1
    $\begingroup$ Ok, let’s see if that changes things... $\endgroup$
    – HTM
    Commented Apr 5, 2019 at 18:25
0
$\begingroup$

Just for fun, castling queenside for checkmate was the original idea here. But there are heaps of duals in such an open position, so it’s not really that strong of a puzzle. I also managed to get that idea out in two less moves then stipulated.

The Intended Solution:

! ! 1. Ke4 Bc4 2. bxc4 g5 3. Kd4 g4 4. Ke4 h5 5. Kd4 h4 6. Ke4 Rh5 7. Kd4 Re5 8. fxe5 Qa5 9. Ke4 Qc5 10. bxc5 a5 11. Kd4 a4 12. Ke3 a3 13. Kd4 a2 14. Ke3 a1=R 15. Kd4 R1a3 16. Ke3 Rc3 17. bxc3 g3 18. Kd4 h3 19. Ke3 g2 20. Ke2 g1=R 21. Ke3 Re1+ 22. Kd4 Re3 23. fxe3 h2 24. Ke4 h1=Q 25. Kd5 Qh7 26. Kd4 Qe4+ 27. fxe4 O-O-O#

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.