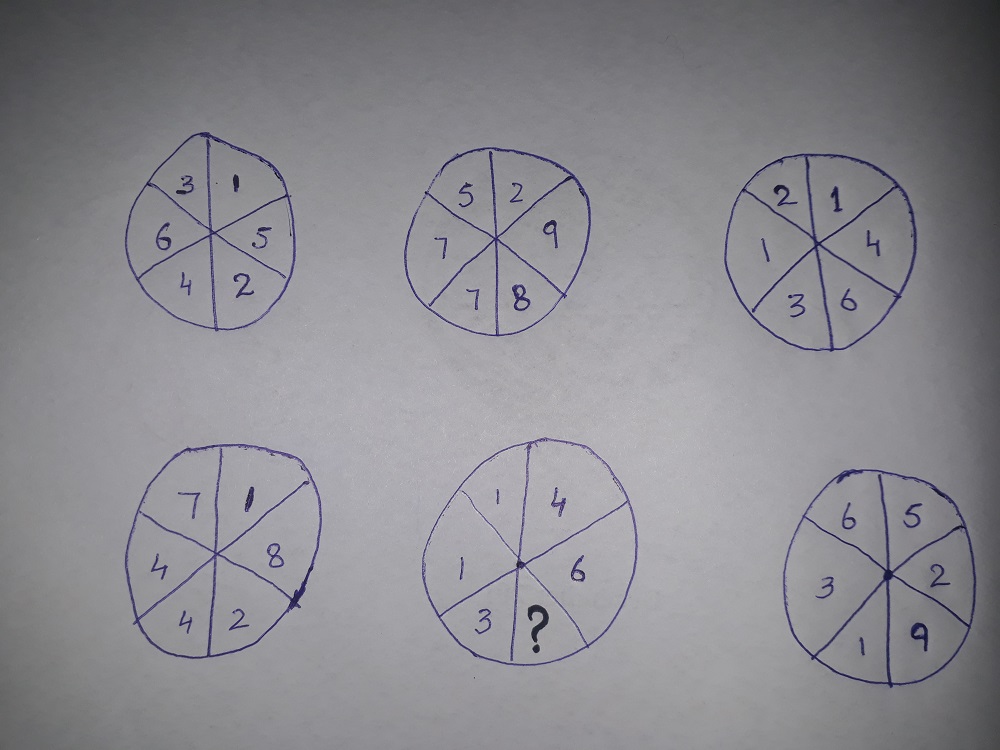
While solving the number puzzle, I couldn't find any solution for this problem. Please help me solve it and briefly explain your answer. Which number will replace the question mark in the circle below?
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
3
7
In the first row,
The number in the middle circle is the sum of the numbers in the left and right circles in the same positions.
In the second row,
The number in the middle circle is the difference between the numbers in the left and right circles in the same positions. It does not matter which is subtracting from which, it is the absolute difference.
-
$\begingroup$ Interestingly, if the 5 in the bottom right circle is changed to a 3 then the pattern which has only subtly changed would be more easily stated, because the upper and lower rows would have the same pattern. Of course, this would change the value of the "?" $\endgroup$– OctopusCommented Dec 11, 2018 at 23:06
-
$\begingroup$ @Octopus I'm afraid I don't understand. Even if that were changed, the top row's pattern wouldn't function in the bottom row. Care to expand? $\endgroup$– kanooCommented Dec 12, 2018 at 14:42
-
$\begingroup$ Diff between circle A and circle B is in circle C ... on both rows, but only if the 5 is changed to a 3. $\endgroup$– OctopusCommented Dec 12, 2018 at 17:45
$\begingroup$
$\endgroup$
3
If we number the circles as follow:
First row A B C
Second row D E F
On the first row we get for each corresponding sector B(n) = A(n) + C(n)
namely: 5 = 2 + 3, 7 = 6 + 1, etc.
On the second row the rule seems to change to alternating D(n) - E(n) = F(n)
and in the next segment D(n+1) + E(n+1) = F(n+1)
Starting from top left we get 7 - 1 = 6, 1 + 4 = 5, 8 - 6 = 2, 2 + ? = 9
Which leads us to ? = 7
answered Dec 11, 2018 at 15:14
-
$\begingroup$ Your answer was invisible when I answered. I'd like to point out, however, that the answer that I have provided is simpler and the rule for the second row more closely matches the rule for the first row. +1 anyway $\endgroup$– kanooCommented Dec 11, 2018 at 15:26
-
$\begingroup$ @kanoo Fair enough, I'm not arguing about being first. $\endgroup$ Commented Dec 11, 2018 at 15:28
-
1$\begingroup$ Nono, I know you're first, the timestamps prove that lol and I don't spend enough time on this site to care about the points. No ill intent my friend. $\endgroup$– kanooCommented Dec 11, 2018 at 15:30