Brain Testing Logical Reasoning Puzzle

Question

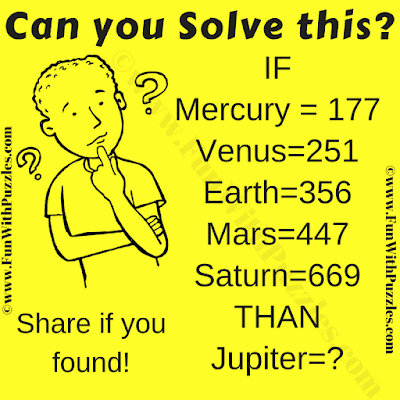
Can you answer this logical puzzle? Puzzle Source: https://www.funwithpuzzles.com/2018/12/brain-testing-logical-reasoning-puzzle.html

Answer 1

Answer:

Jupiter = 578

Explanation:

The first digit is the position from the Sun (Mercury = 1, Venus = 2, etc.) so Jupiter = 5.
The second digit is the length of the planet's name, so Jupiter = 7.
To get the third digit, multiply the first and the second modulo 9; e.g. Venus $2 \times 5 = 10 \equiv 1 \pmod 9$. Therefore, Jupiter is $5 \times 7 = 35 \equiv 8 \pmod 9$.

Also,

THAN is misspelled, it should be THEN.

Answer 2

Mercury=177

First number from left is planets position from the Sun so mercury is the first planet from the sun, hence number 1.

Second number that is number 7 is total number of alphabets present in the word Mercury.

The third number is the multiplication of first and the second i.e 1x7 if the resultent number is of two digits then we have to add those two digits with each other but if the result is a single digit then that single digit itself is the third number.

Similarly for Venus=251
i.e Venus second planet -->2
No of letters in Venus -->5
Multiplication of 2x5=10
Adding the two digits of 10 --> 1+0=1
Hence 251.. ✓✓✓✓😀

So for Jupiter
Jupiter is 5th planet from the Sun✓✓✓--> 5
Jupiter word has 7 letters so ✓✓------------> 7
Third digit from left5x7= 35 --> 3+5=8✓✓
So answer is "Jupiter= 578"✓✓✓✓✓✓

Answer 3

The first digit gives the position of the planet from the Sun say it as A The second digit is the number of letters on the left side of the equation say it as B To get the third digit, multiply the first and second digit and add the digits of this result obtained. e.g 5x7 = 35 => 3+5 = 8