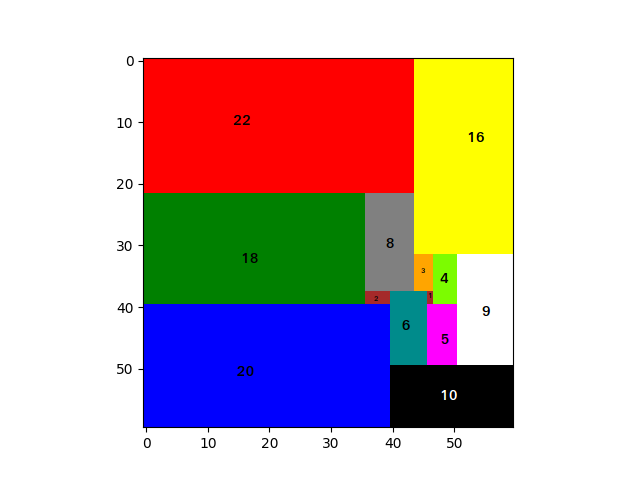
Pretty sure an expert hand tiler would be able to find this. Please post a spoiler only if you hand tile it...
Tile a $60\times60$ square with $1:2$ rectangles of sizes 1,2,3,4,5,6,8,9,10,16,18,20,22
No gaps/overlaps of course. Solution is unique, ie there is only one way to tile a $60\times60$ with all-different integer-sided $1:2$ rectangles, and only one way to fit them together, rotations & reflections aside. Sizes 2,3,4 means $2\times4$, $3\times6$, $4\times8$ etc.
One possible solution is the following
General Method
There are clearly some properties of the tiles which I have taken advantage of here.
The largest three tiles fit neatly together with their short sides along one side of the square and the fourth largest tile fits vertically in the gap beside the largest tile. I tried a couple of different orderings of the largest tiles along the left side but, with this one in particular, the size $8$ tile fits neatly in the upper gap with the size $2$ tile underneath.
From there it didn't take too long to fit the rest together in a neat way.
