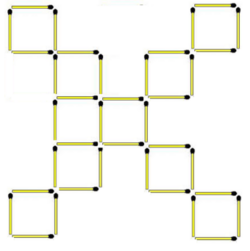
There are 10 squares in the figure. Move 3 matchsticks to create 17 squares. Squares don't have to be equal in size?
$\begingroup$
$\endgroup$
5
-
$\begingroup$ $Move$ 3 matchsticks or $Remove$? $\endgroup$– SeyedCommented Dec 28, 2017 at 17:06
-
$\begingroup$ Mostly likely move... Remove would be impossible? @Seyed $\endgroup$– QuintecCommented Dec 28, 2017 at 17:07
-
2$\begingroup$ The obvious solution has 17 squares and one extra match sticking out like a sore.. stick. This is, in general, an uncool thing: all the matches should be meaningful parts of the solution. I hope there is a less obvious solution that makes use of all the matches. $\endgroup$– BassCommented Dec 28, 2017 at 17:12
-
$\begingroup$ It also means there is no unique solution. The sore-stick solution can be formed in 16 different ways. $\endgroup$– ekhumoroCommented Dec 28, 2017 at 22:02
-
2$\begingroup$ Assuming there really is no clever "other solution", I'd like to suggest a slight modification: remove the middle stick in the vertical line of 5 sticks, and transform the problem into this famous one. Notice the lack of sore sticks, how the starting position is 4-way symmetric and the answer is not, and how all the squares in the starting position are non-adjacent, and the answer has squares crammed in as tight as possible. $\endgroup$– BassCommented Dec 28, 2017 at 23:44
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
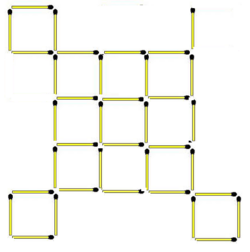
The answer is:
How:
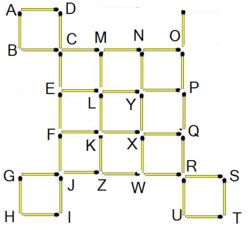
Let's name the sticks.
Here 17 squares are- (1) ABCD, (2) GHIJ, (3) STUR, (4) CELM, (5) EFKL, (6) FKZJ, (7) KZWX, (8) LKXY, (9) MNYL, (10) NOPY, (11) PQXY, (12) QRWX, (13) CNXF, (14) MOQK, (15) EYWJ, (16) LPRZ, (17) CORJ
-
11$\begingroup$ And it even has the antenna at O, with which it can communicate to the alternate universe where it’s ok for a matches puzzle to have matches left over after completing the objective. Not the answerer’s fault, of course, so here’s my upvote. $\endgroup$– BassCommented Dec 28, 2017 at 19:13
-
$\begingroup$ @Bass I don’t know... maybe you’re from an alternate universe where all puzzles have to be perfect? (Not trying to be an ass... but you seem to be hating a lot over a small thing.) $\endgroup$– QuintecCommented Dec 28, 2017 at 21:49
-
3$\begingroup$ @thecoder16, A good puzzle is always explicit about the desired objectives and the allowed means. Therefore, it’s very important to follow the generally accepted conventions, or at least add a note when deviating. In matchstick rearranging, some of the conventions are - no stacking - no breaking the matches - no overlapping - and, very importantly: no surplus. Otherwise the solution to this puzzle could be ”well, it clearly has two squares already. Just ignore the other squares.” $\endgroup$– BassCommented Dec 28, 2017 at 22:52
-
1$\begingroup$ @thecoder16 Also, please accept my apologies for using such unprofessional language in my criticism. I was a bit riled up since this wasn’t the first matches problem with the exact same issue on PSE today. I got a bit carried away. Sorry. $\endgroup$– BassCommented Dec 28, 2017 at 22:59