Arrow Number Boxes

Question

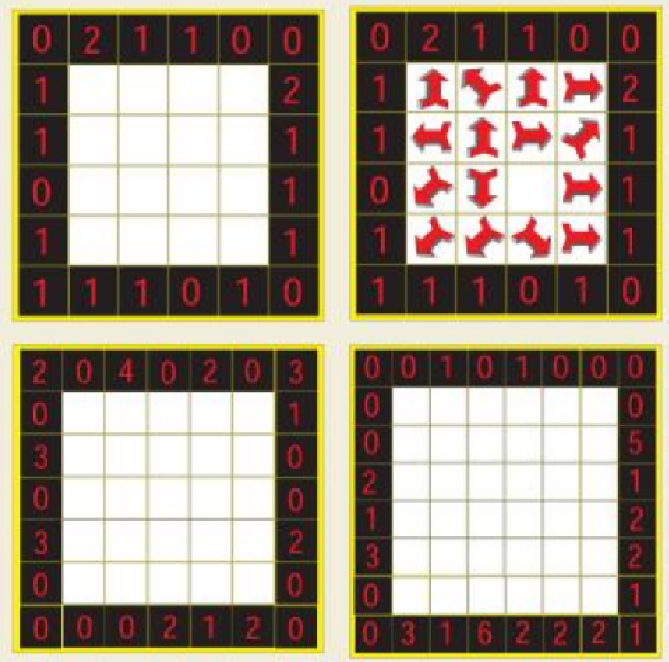
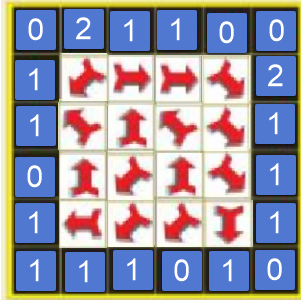
I came across this riddle in The Big Book of Brain Games by Ivan Moscovich (Riddle #12)
the book does provide the solution, but I've got no clue how to go about solving it.
If you do own this book, I suggest you open it right now and try to solve the riddle yourself (but don't look at the answers!); if not, here's the riddle:

The object of this sort of riddle is to place arrows in the boxes according to the following rules:
The arrows must point in one of the eight main compass directions (north, south, east, west, northeast, southeast, northwest, and southwest); the number of arrows pointing to each number in the outer boxes must equal the value of that number; and each box must have an arrow in it. The sample shown (upper right) is a flawed attempt at a solution, since since no arrow can be placed on the blank square within the rules of the game, and one of the outer squares has no arrow pointing at it.
can you find complete solutions for the for the arrow number boxes of order 4 (upper left), order 5 (lower left), and order 6 (lower right)?

So, to sum it up, you need to:
1. give the solution for the original riddle
2. explain the method for solving the riddle
3. (not necessary but much appreciated) provide the background for this riddle; i.e: who created it, what mathematical concept it is based on, etc.

Answer 1

Here's a solution to the first one (I think. I tried to double check, but I may have missed something)

My methodology is probably not optimal, but it went roughly

Start with a "corner direction." Fit it into as many spots as you can to take care of edge pieces.

I then

Looked at which edge had the least open spaces remaining on it. In this scenario, I did all the down right arrows, then did the right facing arrows.

This solved

The whole right hand side, more or less. I added the down arrow to take care of the bottom of the 4th column, as well.

I then added

all the down left arrows. After that, there weren't very many spots left, and I put pieces where they fit.

I'll see if I can apply the same/similar logic to the 5x5 (and eventually the 6x6 maybe) to see if I'm able to better nail down my thought process.

EDIT:

5x5 --

I have come to the conclusion that solving these is VERY non-single-answer-y. The methodology that I used to solve this one was

Draw the possible arrows on each square. After that, find the squares with the fewest possible arrows, and then pick the one that points to a higher number, and draw it in.

After that I

Eliminated any arrows for squares that were completely filled in, and started the process again for the squares with remaining arrows

While prioritizing

Filling out arrows in squares with only one option remaining immediately.

I found multiple possible answers towards the end.