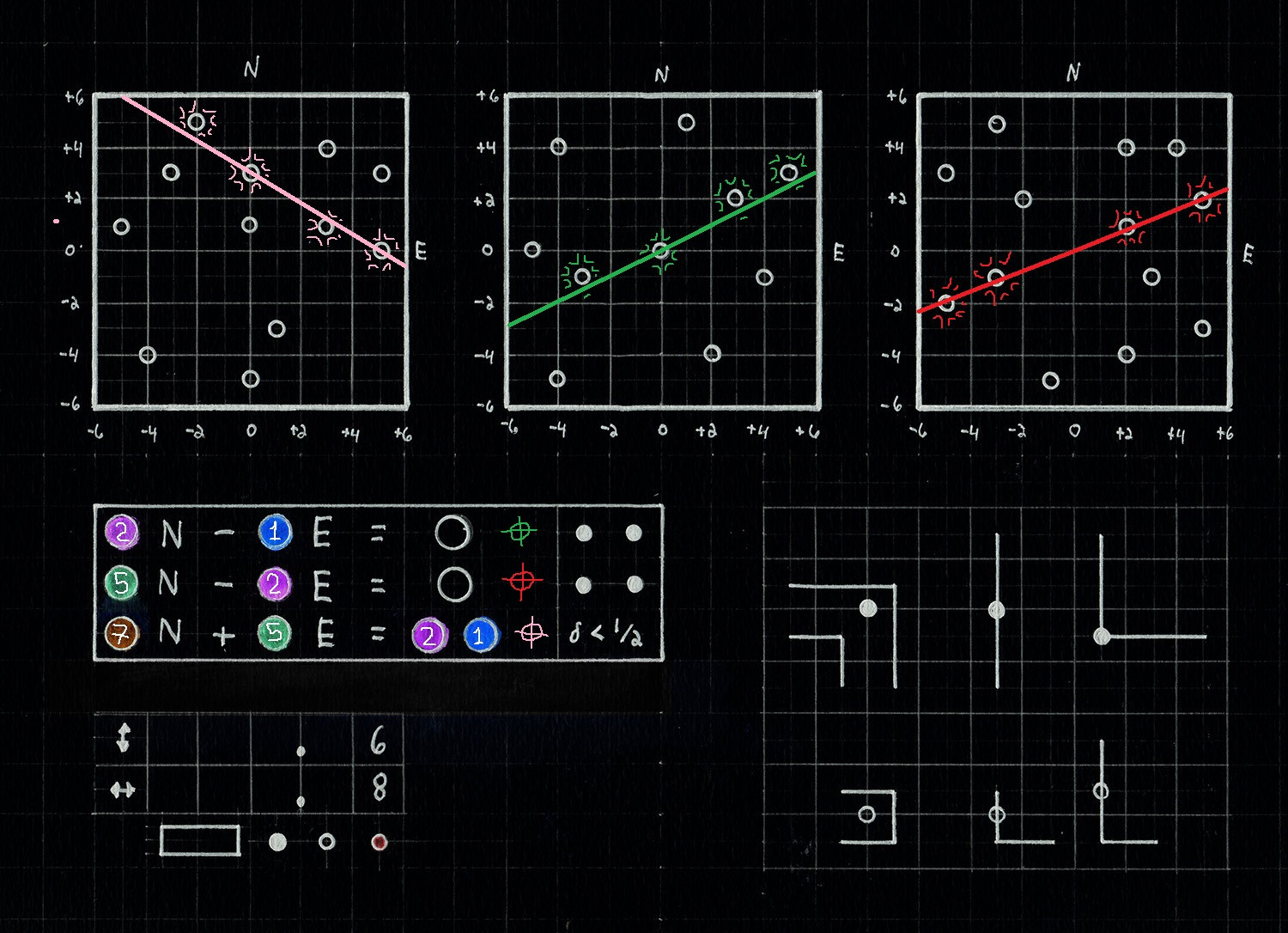
Armed with Thomas Blue's star-finding work, we may solve the 4 logic grids as follows:
The rules of the puzzle
Designate some dots as centers of spiral galaxies. Spiral galaxies must be rotationally symmetric around a this point and contain no other points, and must not overlap. Basically Spiral Galaxies rules, with the rule that every square must be used relaxed.
.
Next, traverse the remaining squares in a single loop, obeying all circle rules as indicated in the picture. Namely - circles centered on a cell obey as Masyu rules (filled circles - turn at cell, go straight both ends; unfilled circles - go straight at cell, turn at at least one end), circles on an edge must be passed through, and also obey: (filled circles - go straight both sides, unfilled circles - turn at least one end), and circles on a vertex point obey: (filled circles - enter/exit 2x2 box twice, on two adjacent sides; unfilled circles - turn at least twice in the 2x2 box)
.
Initially we are not given which circles are filled, but the initial step of calibrating the starfinder gives us which circles are filled.
Step by step solution:
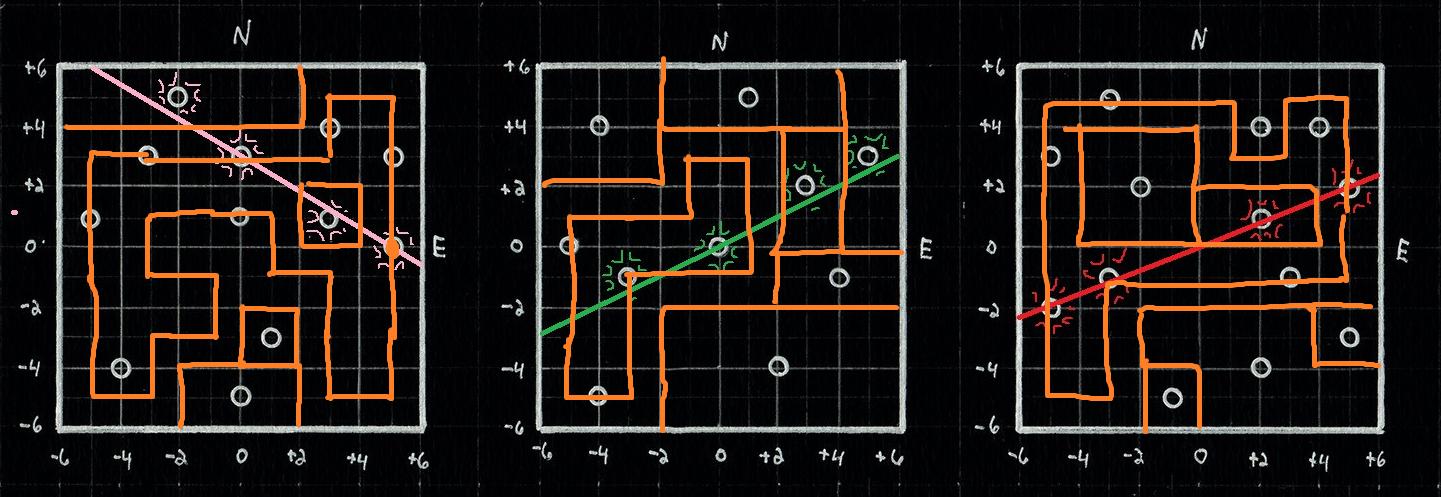
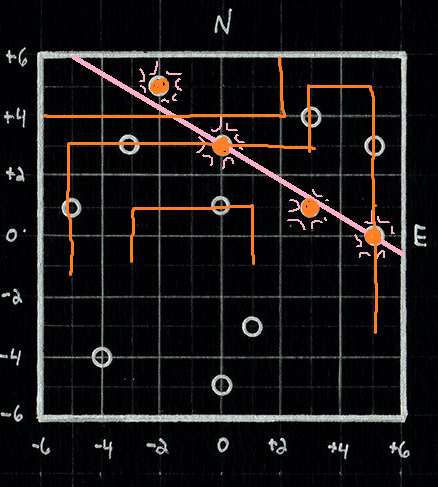
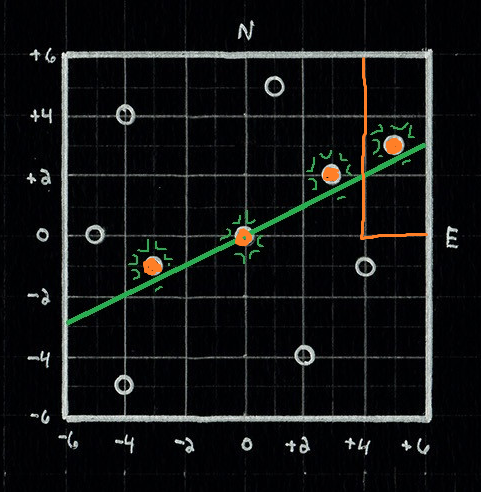
Grid 1
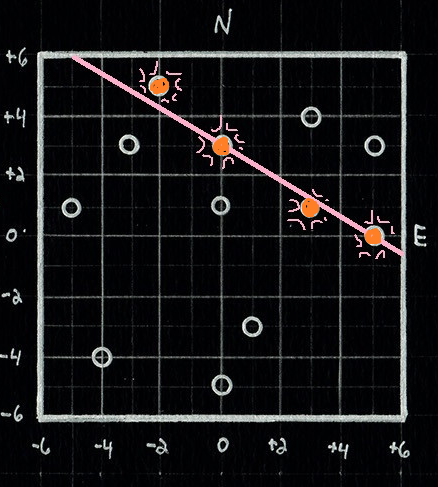
No galaxy can enter N5E5, so it must be part of the loop. This makes the loop intersect two circles (N4E3, N3E5), so we invoke loop rules. This causes the loop to further intersect the circle at (N0E5) so invoke loop rules again.
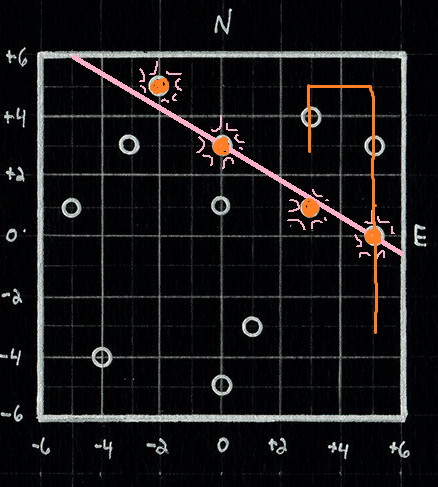
Suppose N5E1 was part of a loop. It would reach the lit up circle at N3E0 at angle, which is bad. So it must be part of a galaxy, and the only center that can take that square is N5W2.
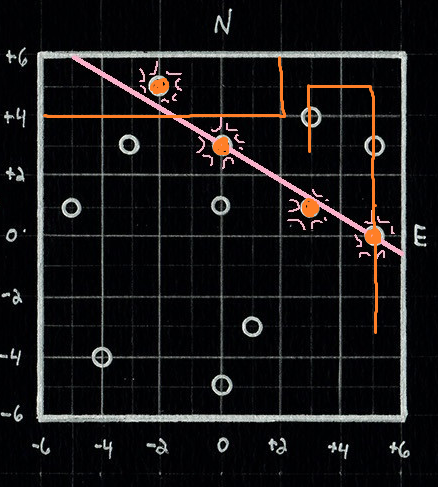
Suppose the loop end at N3E3 went south. Then it must go west to satisfy loop rule, which is forced to turn at the other end at N1W1, south because it can't turn to a lit circle. This would result in N3E0 being a galaxy, making N3W3 a galaxy (since it can't be part of the loop), and then N1W5 a galaxy, and we are stuck because N1W3 can't be galaxied.
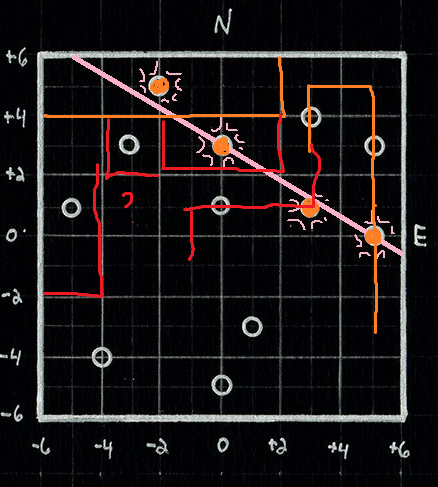
So the loop turns west. We can complete a lot of the loop. Furthermore, N1W3 still can't be galaxied so we get some more of the loop:
N1E3 must be a galaxy. S1E3 can't be part of a galaxy.
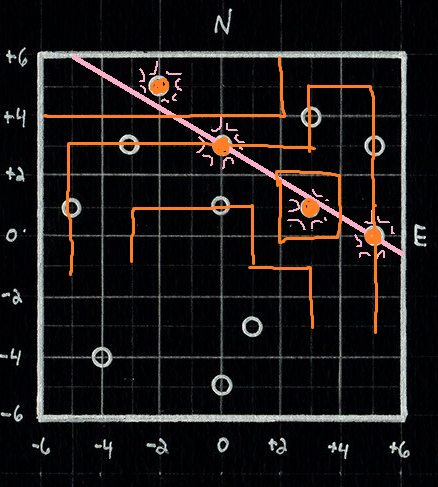
S5E5 isn't part of a galaxy, and neither is S1W1.
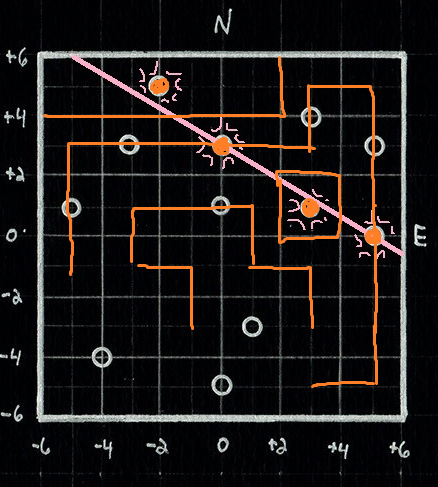
S3E1 isn't part of the loop (it would make a plural loop, and would also prevent the other loop from being completed) and thus we can quickly finish:
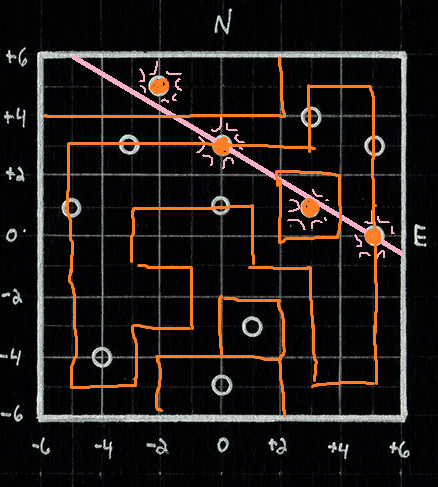
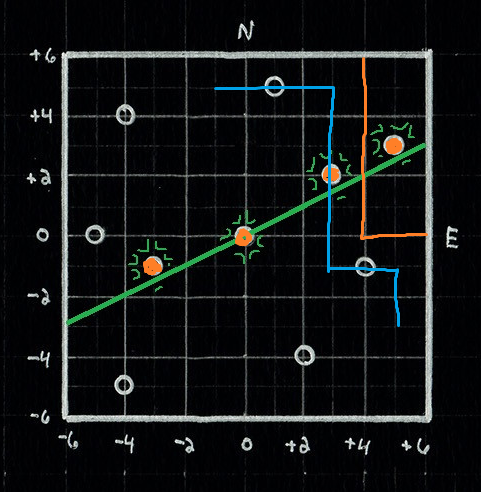
Grid 2

N3E5 can't be a loop clue, or it would clash to the left. So it must be a galaxy clue, and in particular it must extend one up because no other galaxy can reach there.
We do some casework here. First, if N2E3 is a loop clue, we get a bunch of loop clues in:
This forces S4E2 to be a loop clue, which also means S5E5 is part of the loop:
If the dot at the the middle is a galaxy, it must extend to N3E1 or else it blocks things. That means that N3W1 is part of the loop, leading to contradiction:

So what if the origin is a loop clue? Then we get the following:
Also a contradiction. So N2E3 is a galaxy, forcing N5E1 to be a galaxy as well:
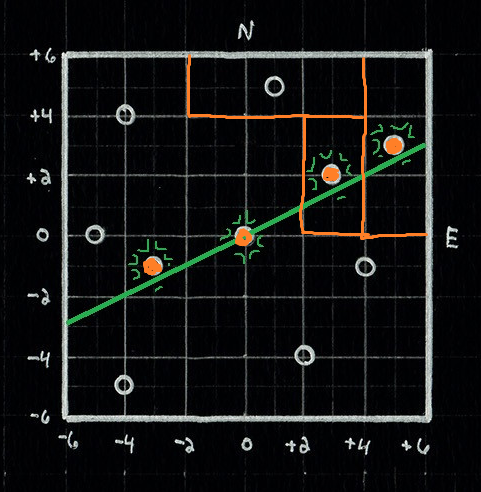
Now the center dot can't be a galaxy, since if it were, then N3W1/N3E1 would be inaccessible for the loop. So it is a loop clue. One of the two directions in the 2x2 box is west, since east is blocked:
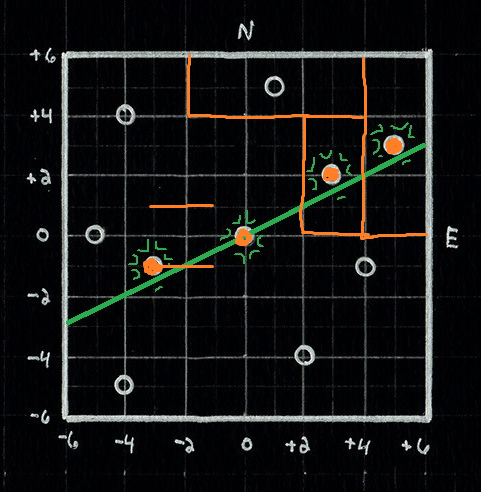
We may now resolve the loop clue at S1W3.
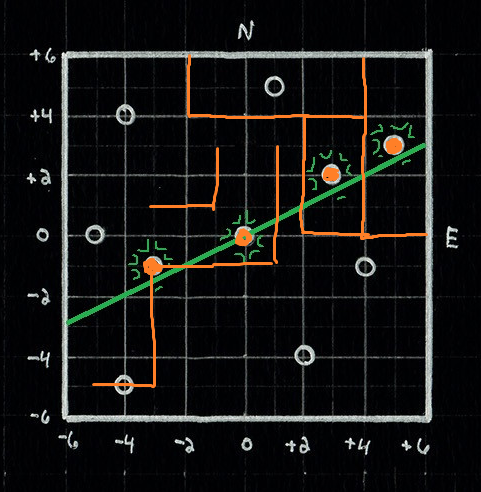
The remainder of the loop is straightforward:

Finally, we are glad that the rest of the clues can be resolved as galaxies.
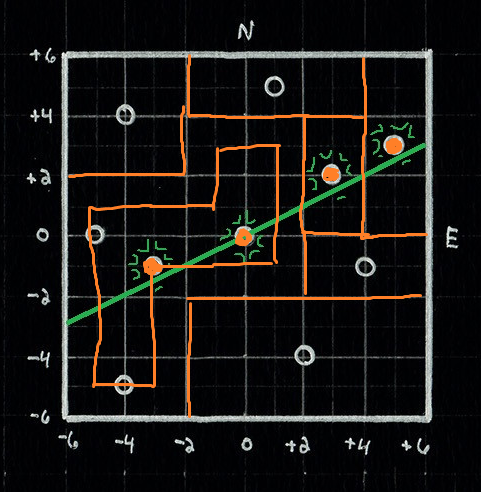
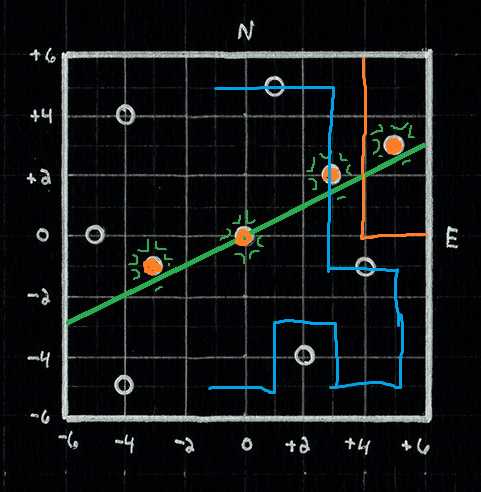
Grid 3
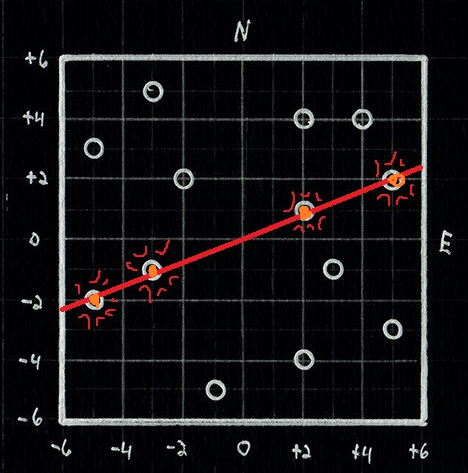
Fortunately, this one is a bit easier. (Also, the solution is indeed rotated from the first image.) It also introduces us to overlapping dots, which absolutely cannot be galaxy clues, which gets us a foothold already:
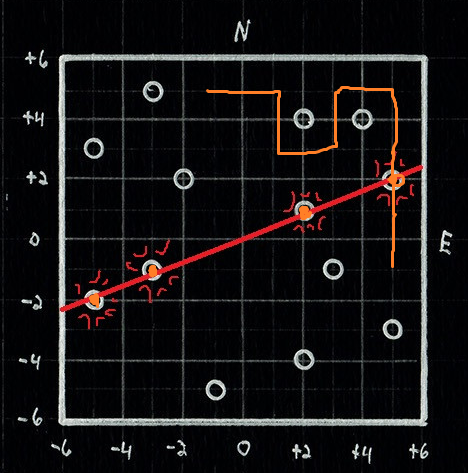
N1E2 can't be a loop clue, as it would crash into the loop later. It's a galaxy clue:
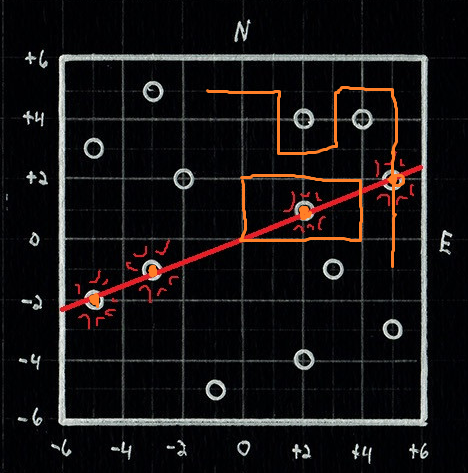
Suppose S5E5 was part of the loop. Both possibilities lead to contradiction.
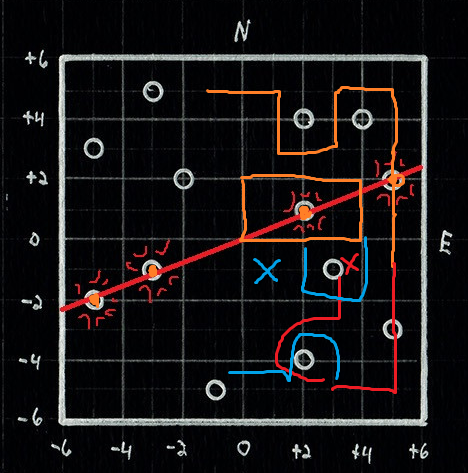
That gets us to here, after getting a quick chain of galaxy deductions:
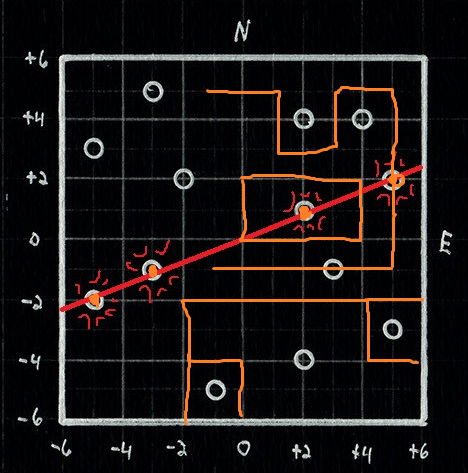
No galaxy can reach S5W3.
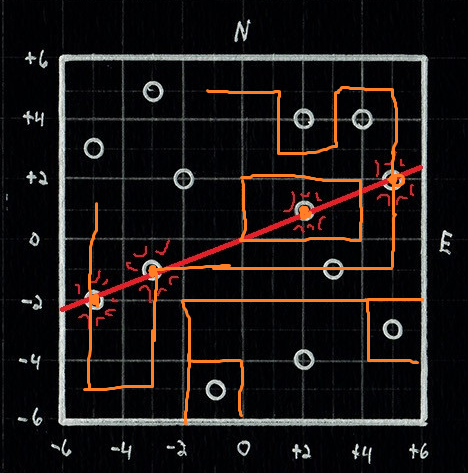
No galaxy can reach N5W5. Finally, the remaining clue can be a galaxy.
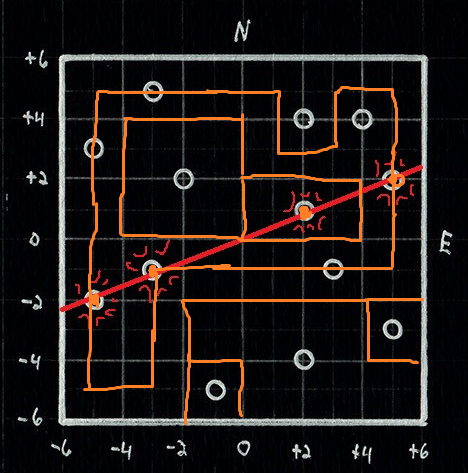
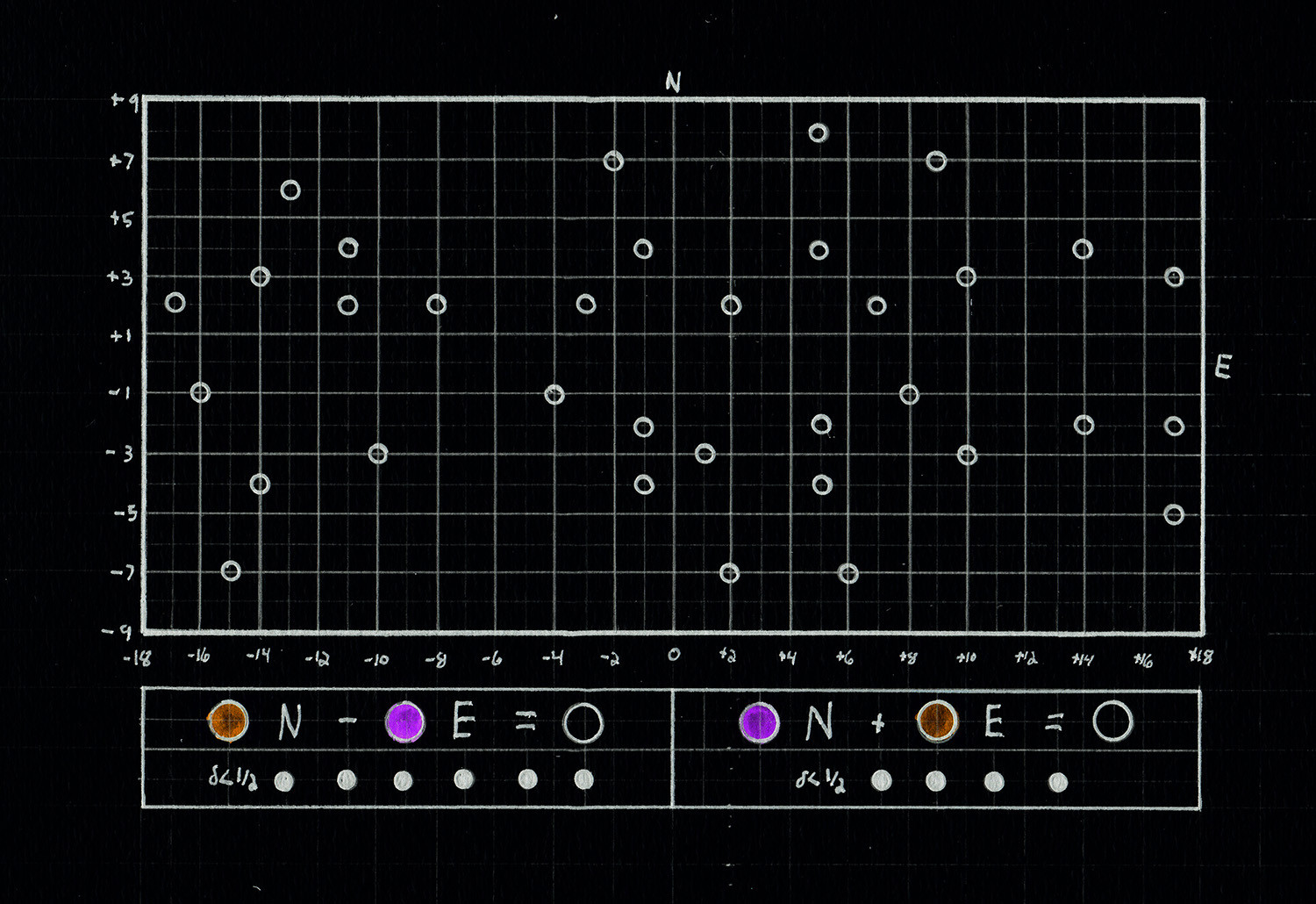
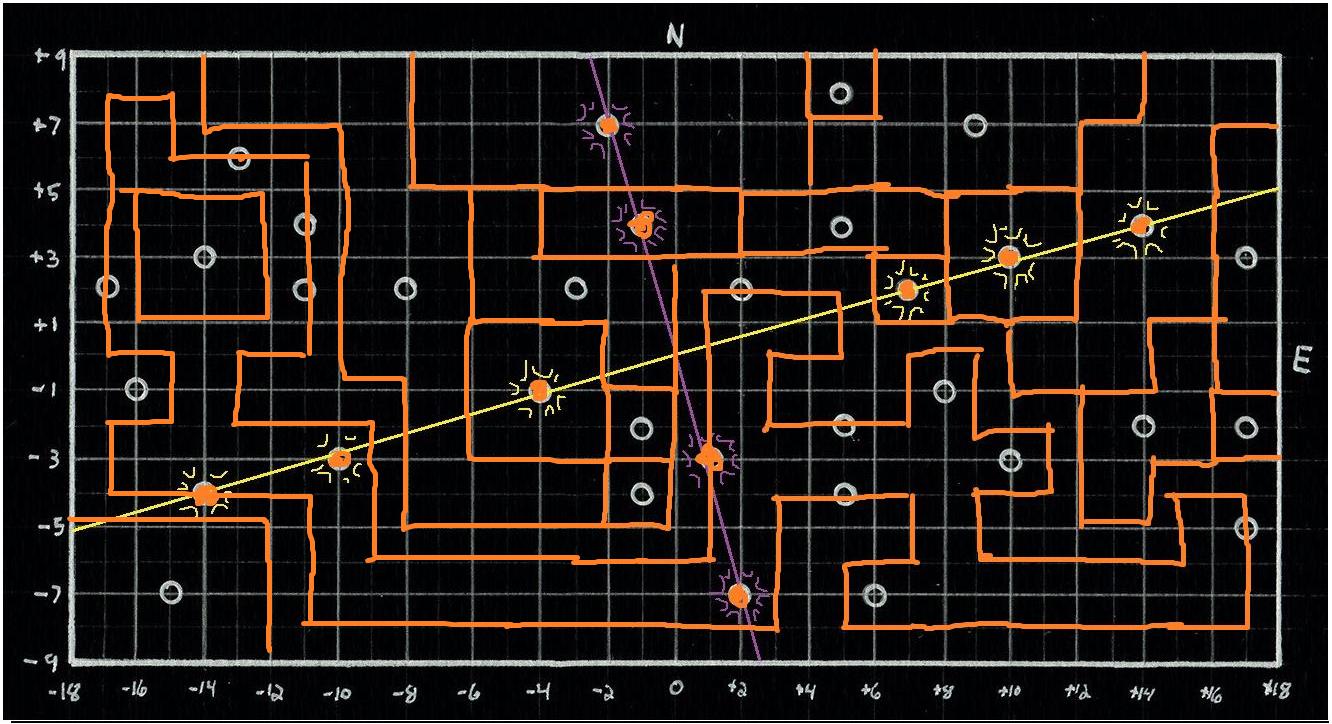
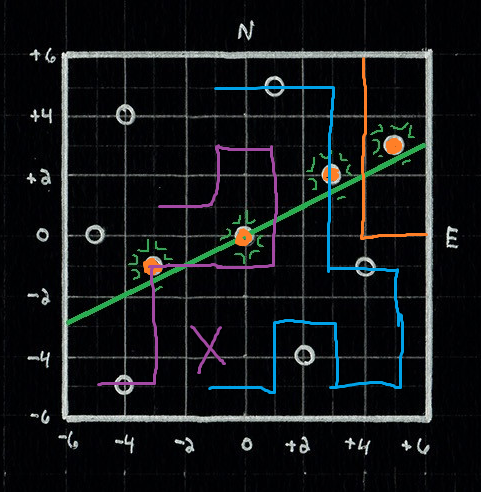
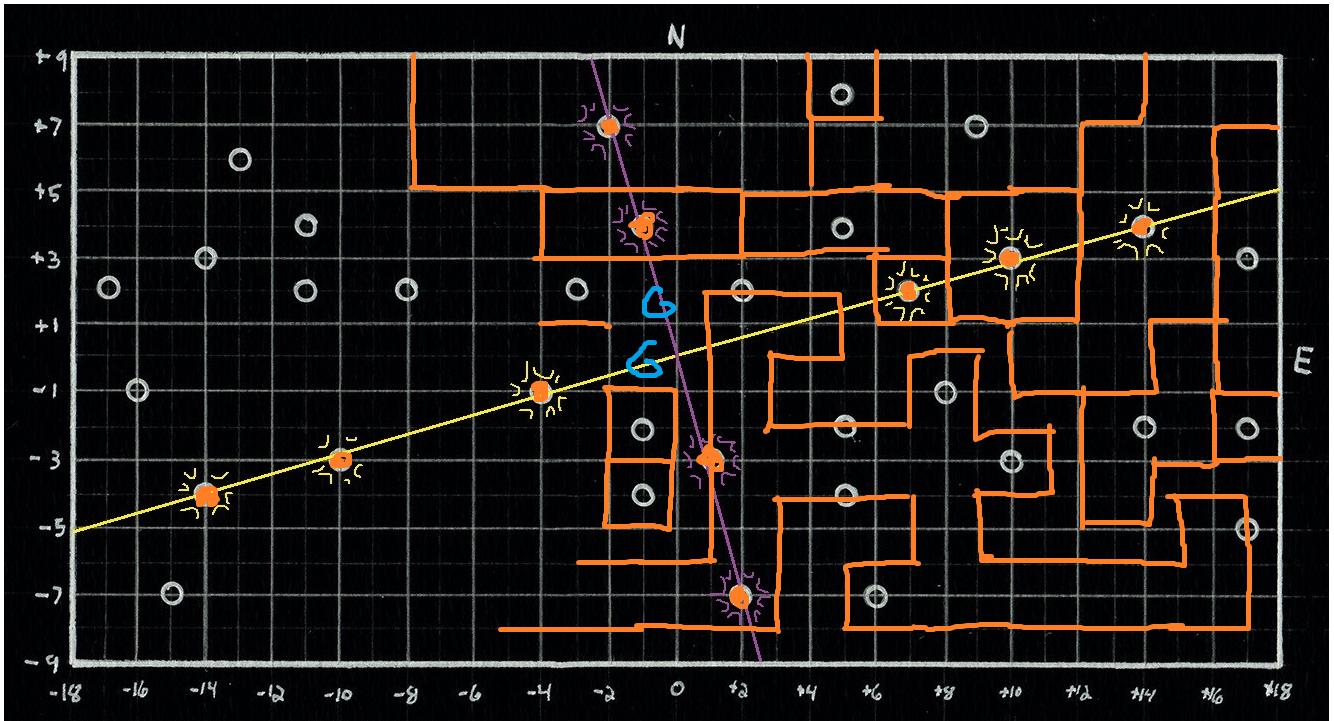
Big grid
Initial deductions. S8E17 has no accessible galaxies, thus it crosses into S5E17, and turns left. That makes S2E17 a galaxy. Also, S1E8 and S3E10 overlap, so they are both loop clues.
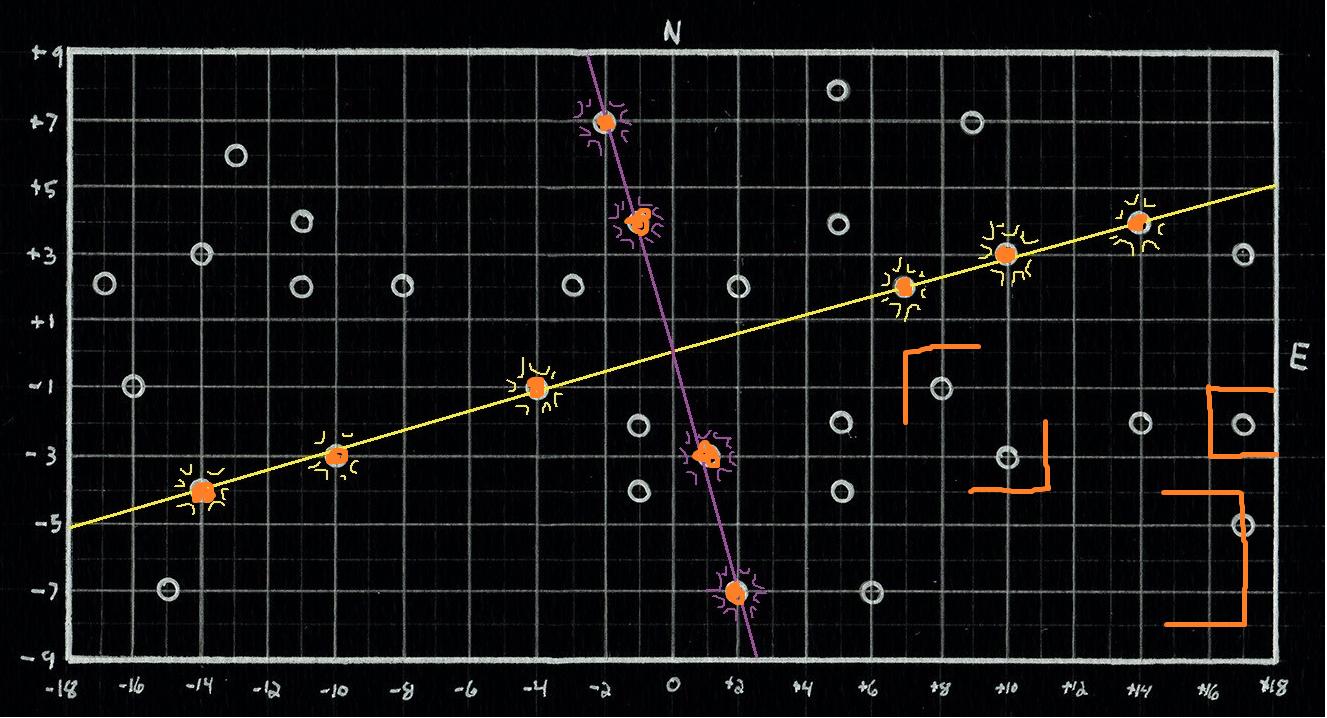
What if N4E14 was a loop clue? It would force N3E10 and N3E17 to be loop clues as well, resolving as follows:
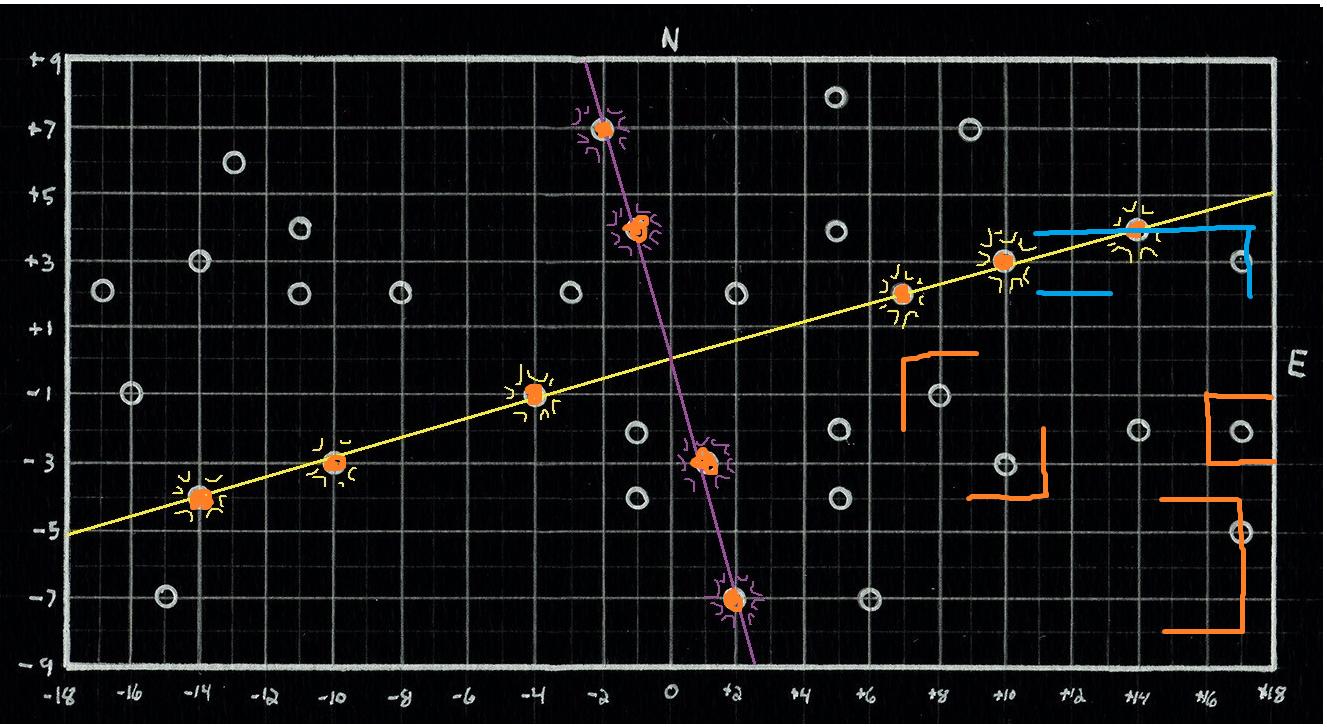
If N3E10 points north, it results in the following plural loop.
If N3E10 points south, it results in the NE corner requiring a plural loop (it can't be covered by a galaxy due to N6E13)
Thus, N4E14 must be a galaxy. Also, N3E10 is also a galaxy, as if it were a loop clue, it would cause N2E7 to be a loop clue, but as we can see it can't complete a full turn.
If N0E17 is part of the loop, then that makes N3E17 a loop clue, which is bad, as N3E17 does not have enough space to turn.
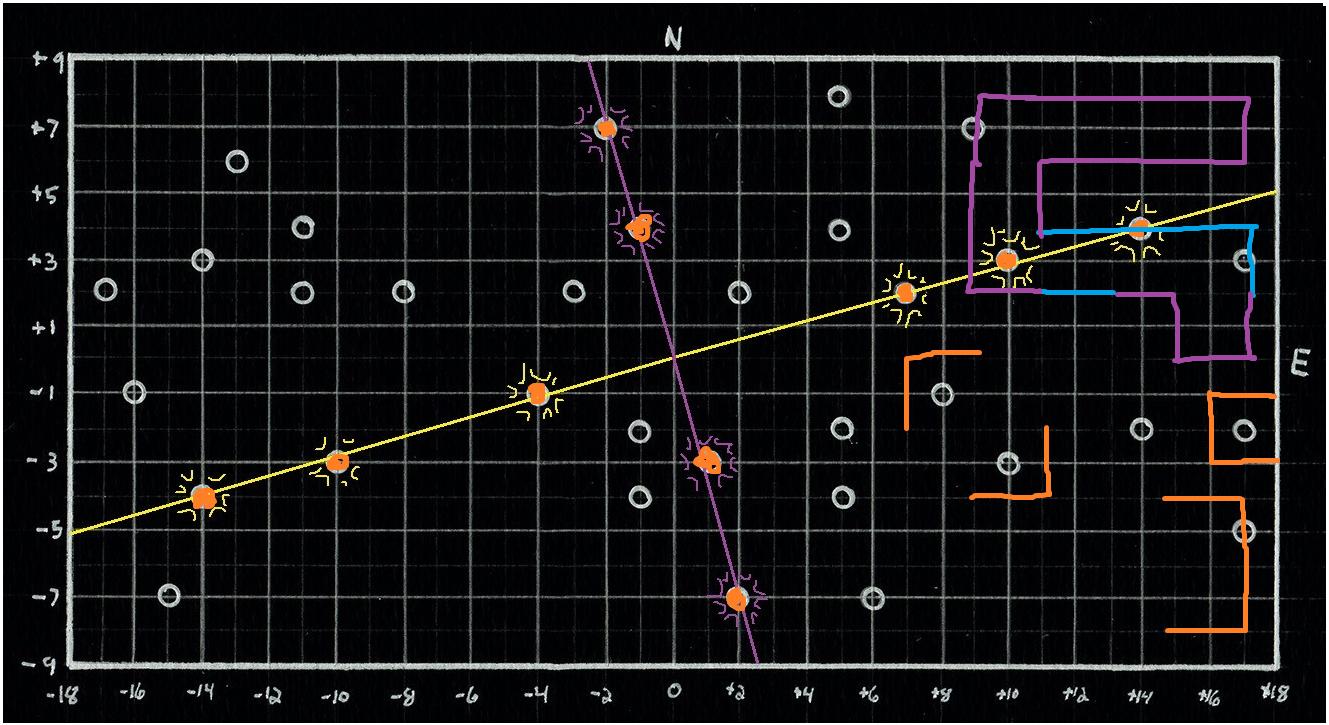
Two galaxies could claim ownership of N0E17. N4E14 leads to a square being inaccessible.
This means that N8E17 is a galaxy square. There are two galaxies that could own it - N7E9 or N4E14. What if N7E9 were that galaxy?
Ok, we've just deferred our notch issue some squares westward. This burden goes to N7W2.
And again...
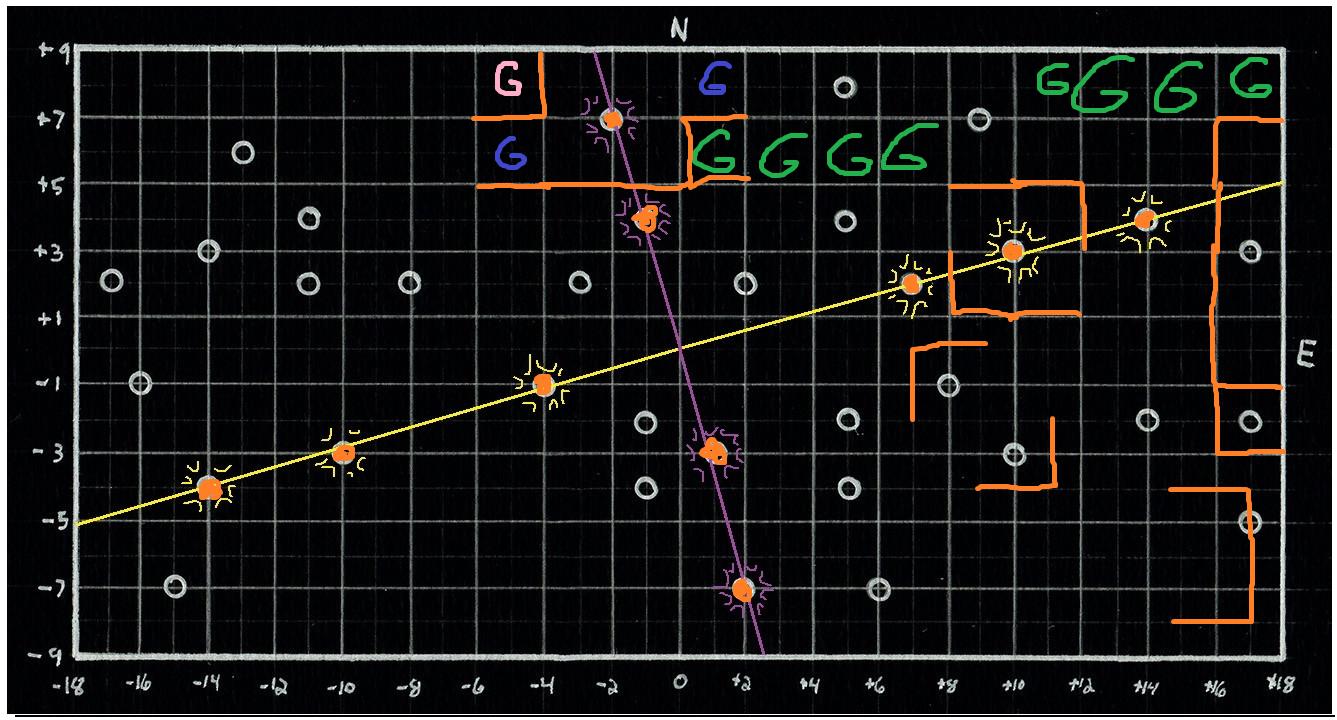
The buck stops here. This leads to a contradiction.
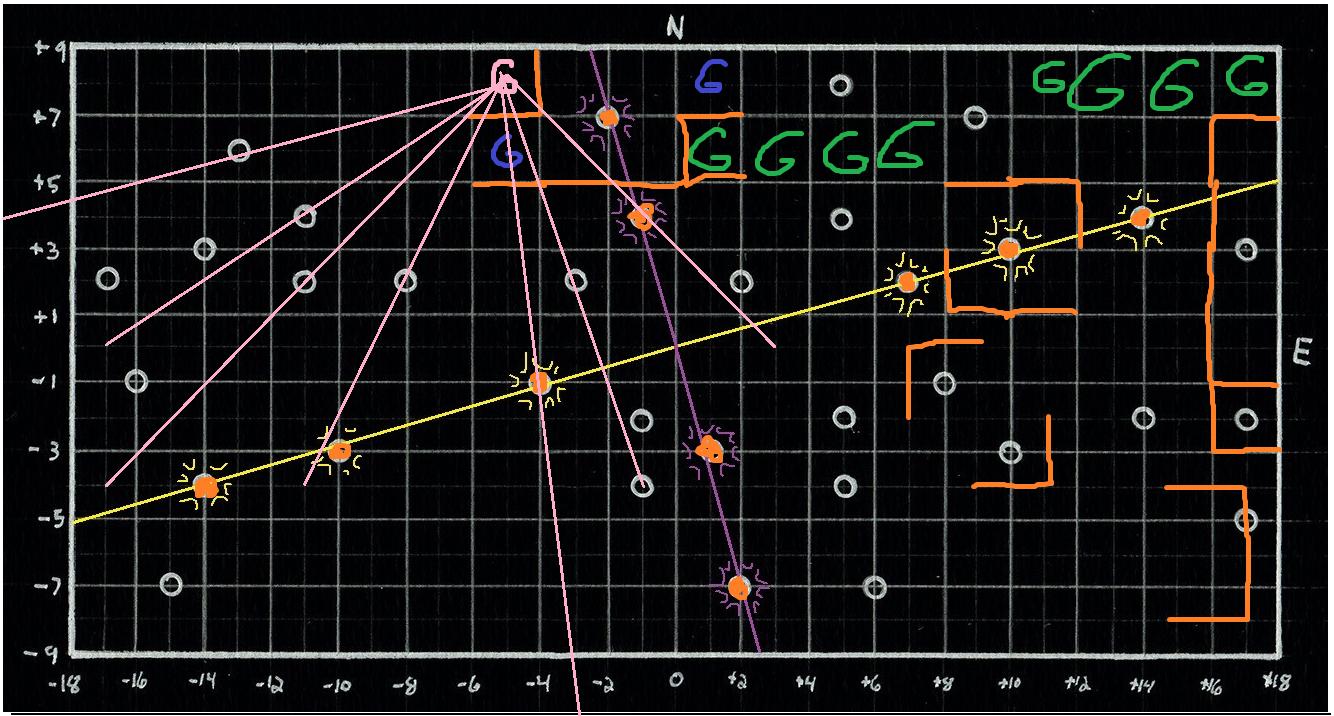
Instead, N4E14 taking N8E17 is eminently more reasonable. Note that N4E14 must take N2E13 since it must now take N6E15.
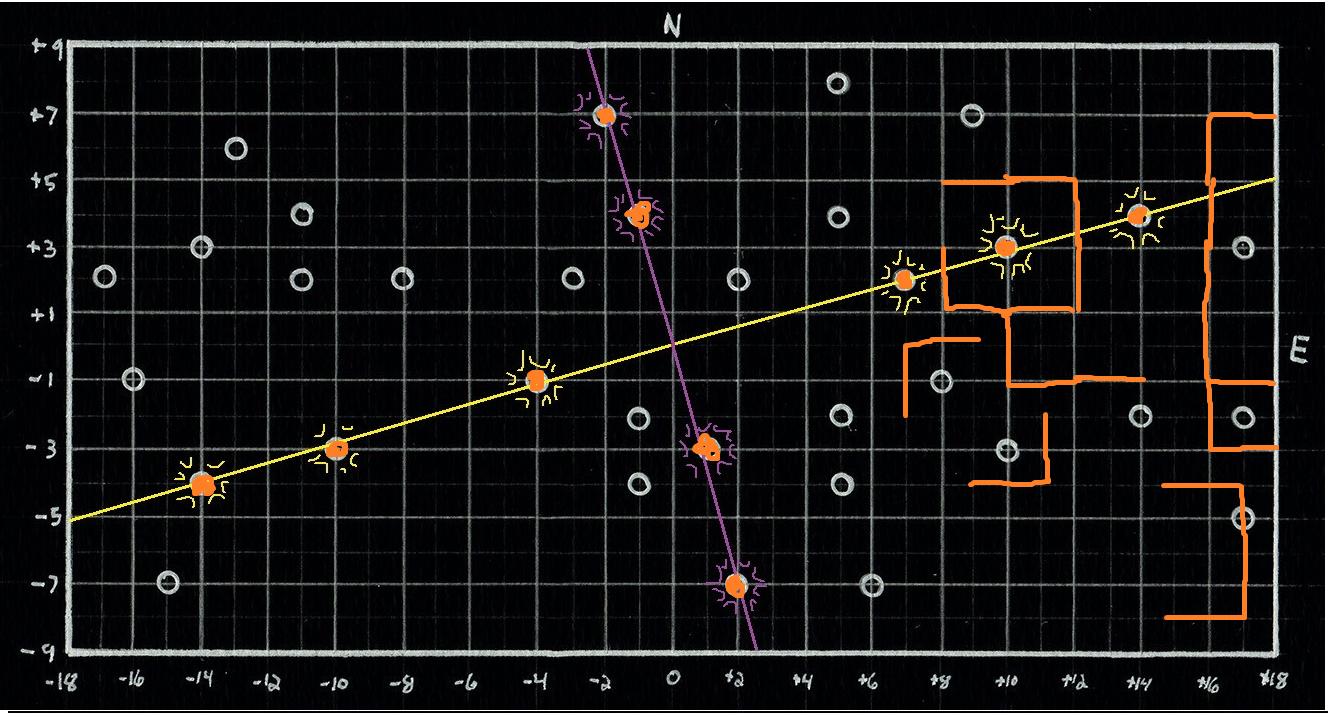
Detour from galaxying - what if S2E14 were a loop? We end up with a plural loop. S4E13 and S6E15 are too far away from any potential galaxy center and end up with two neighbors. So S2E14's a galaxy. Back to galaxying.
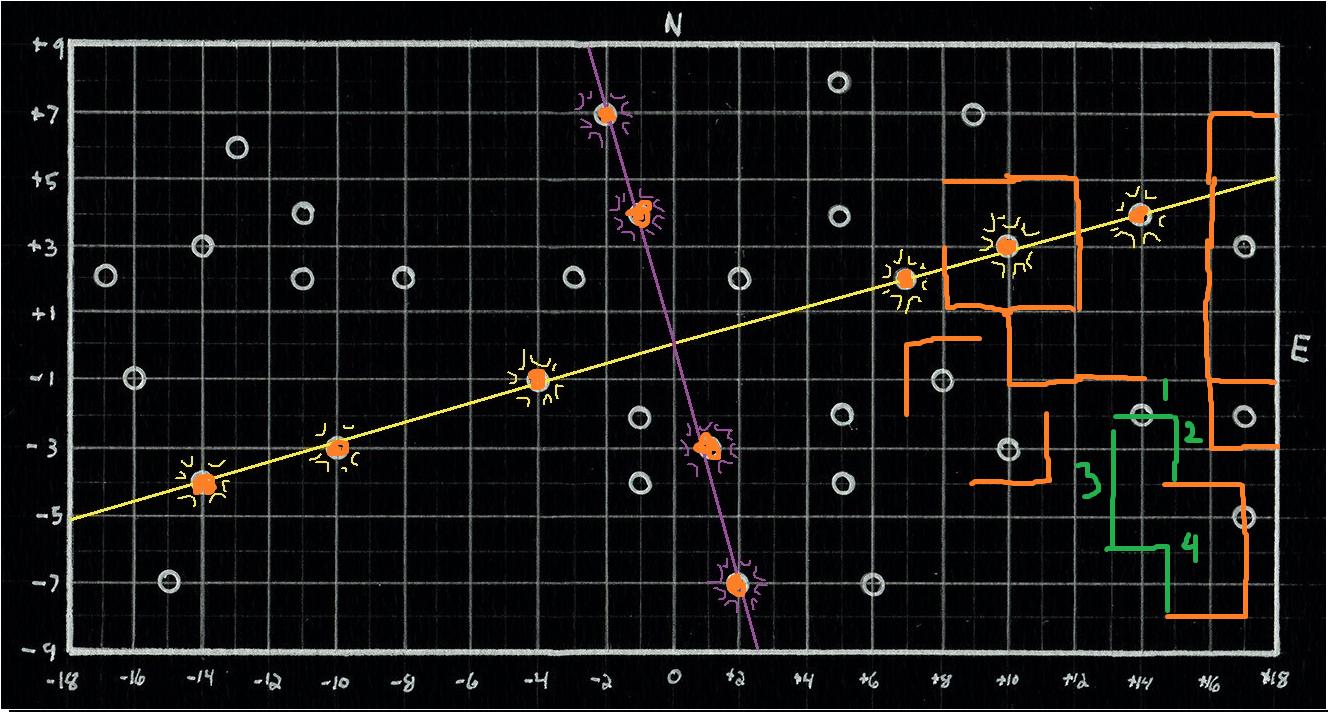
It is not too hard to see that galaxy S2E14 must extend down exactly one block to S4E13. If it doesn't, the loop will end up missing an excess square, and if extends two blocks, there is no way to extend both ends of the loop out of that corner.
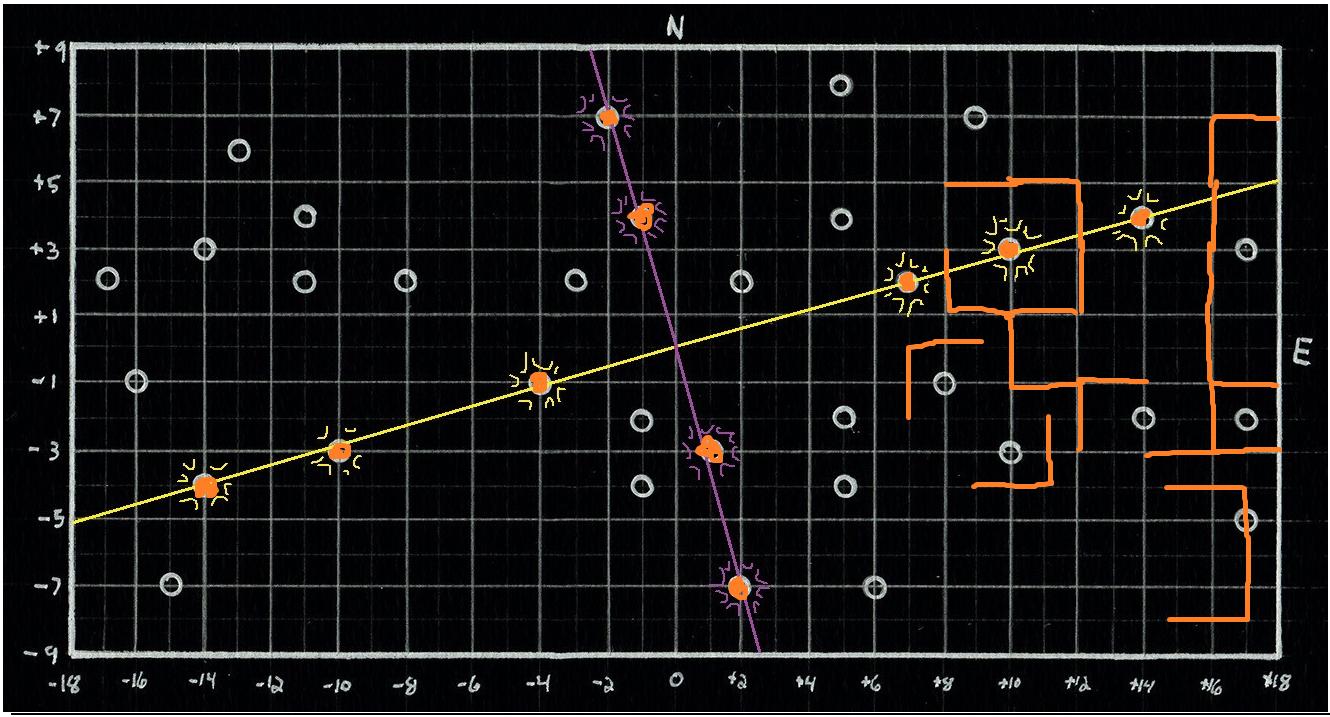
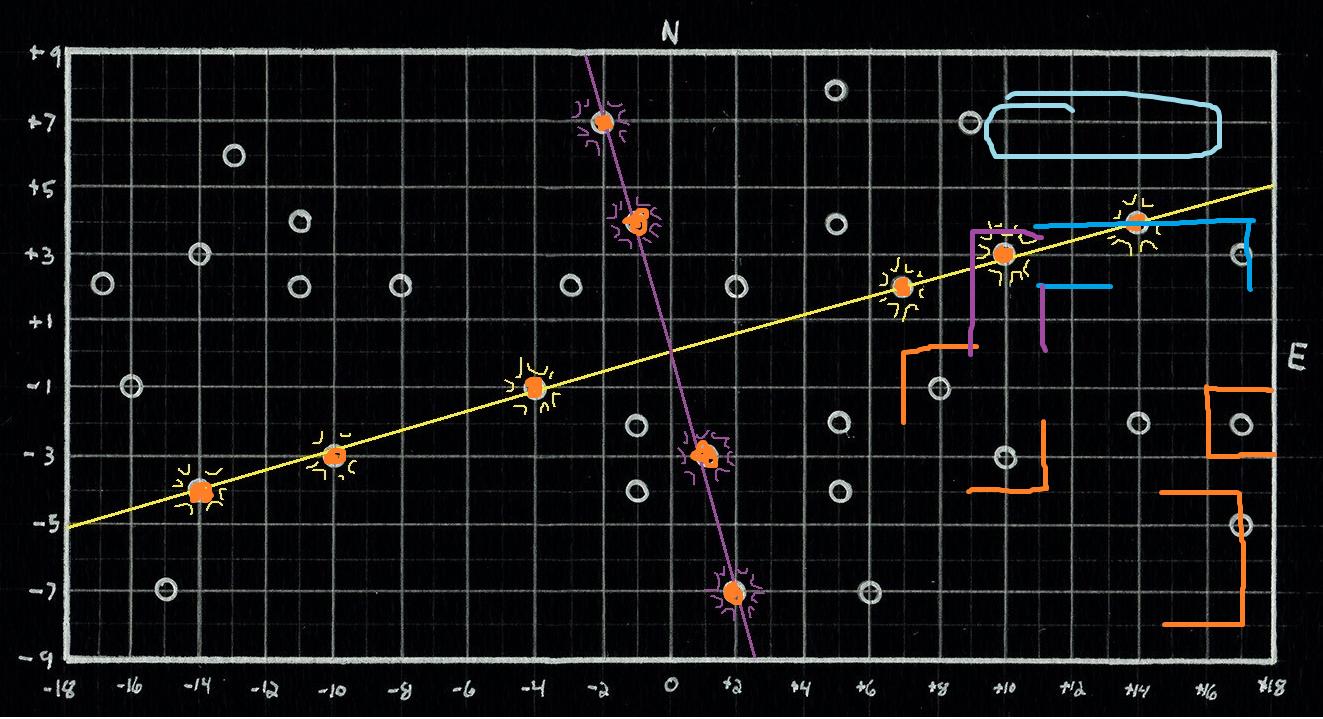
That lets us finish a lot of galaxies. We also now get that N2E7 is a one-square galaxy, which in turn means that N4E5 is a three-square galaxy.
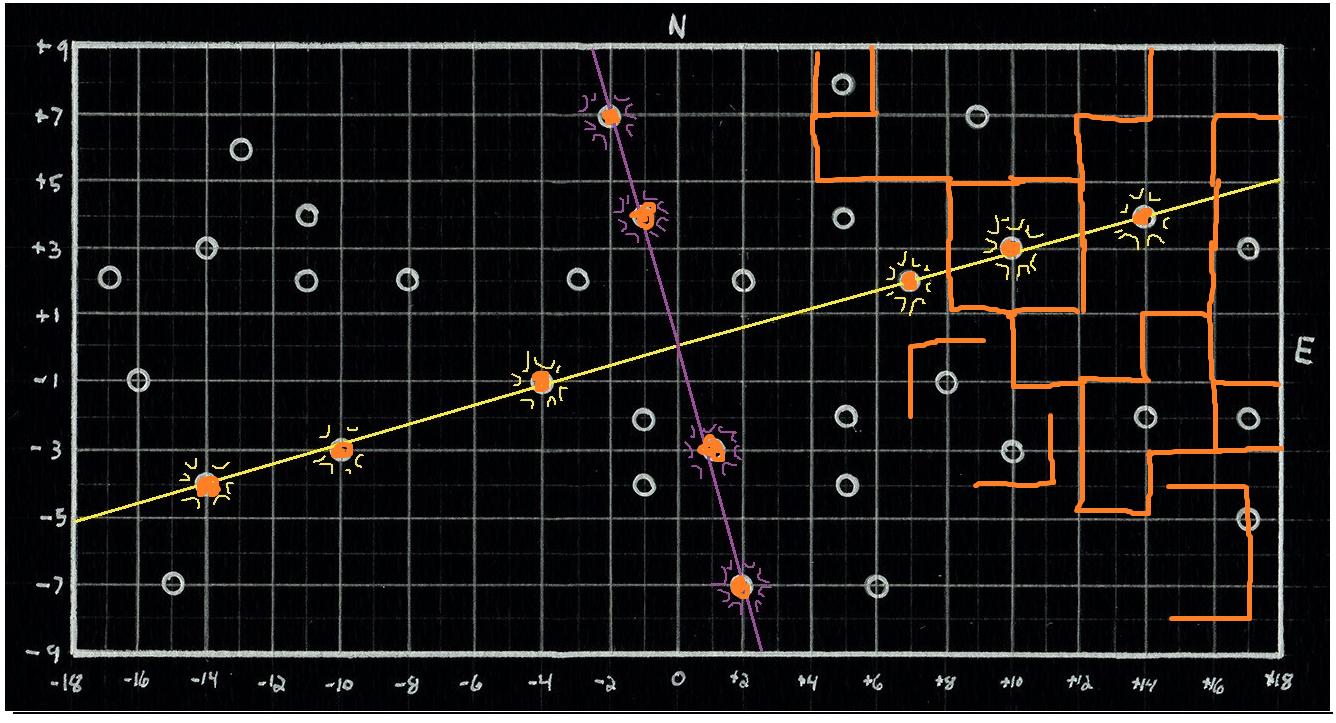
Let's also do the loops up to this point.
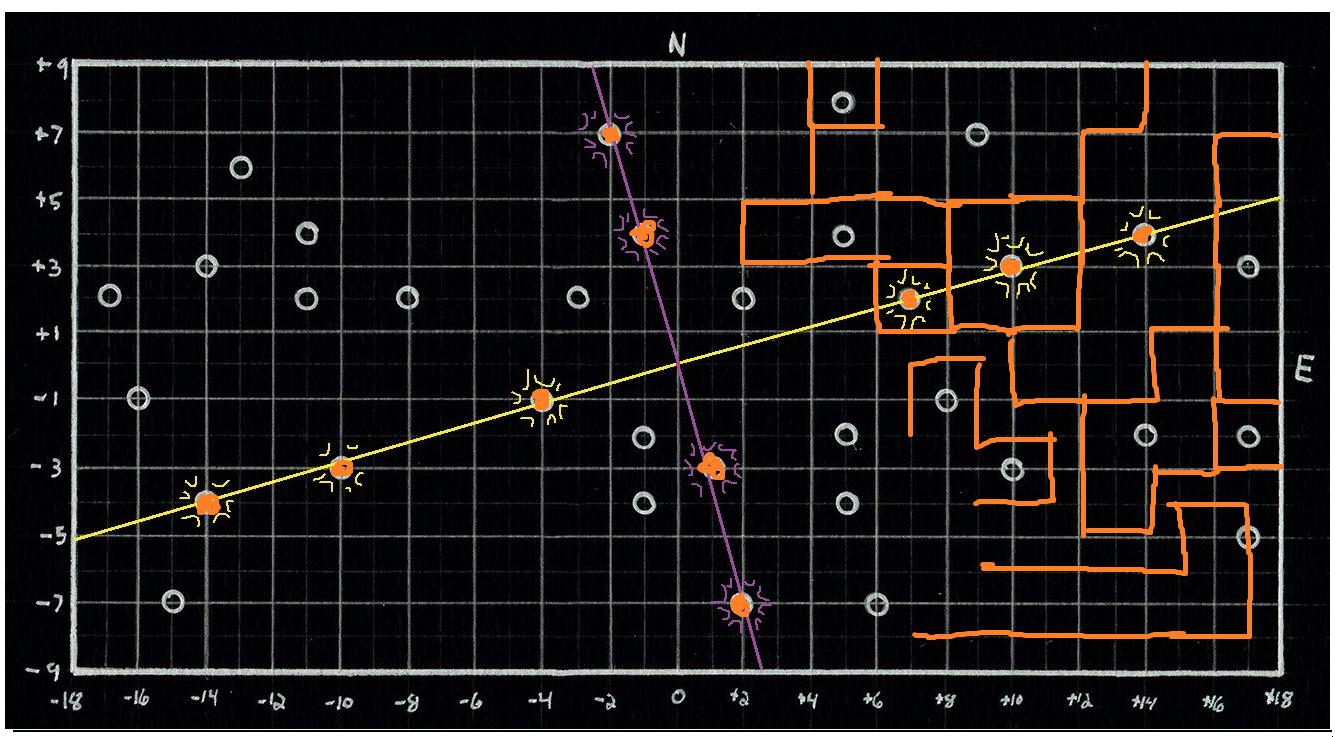
Ah, now our loop enters S7E6. There are two possibilities to resolve this. One of them leads it to S7E2 where it faces swift contradiction.
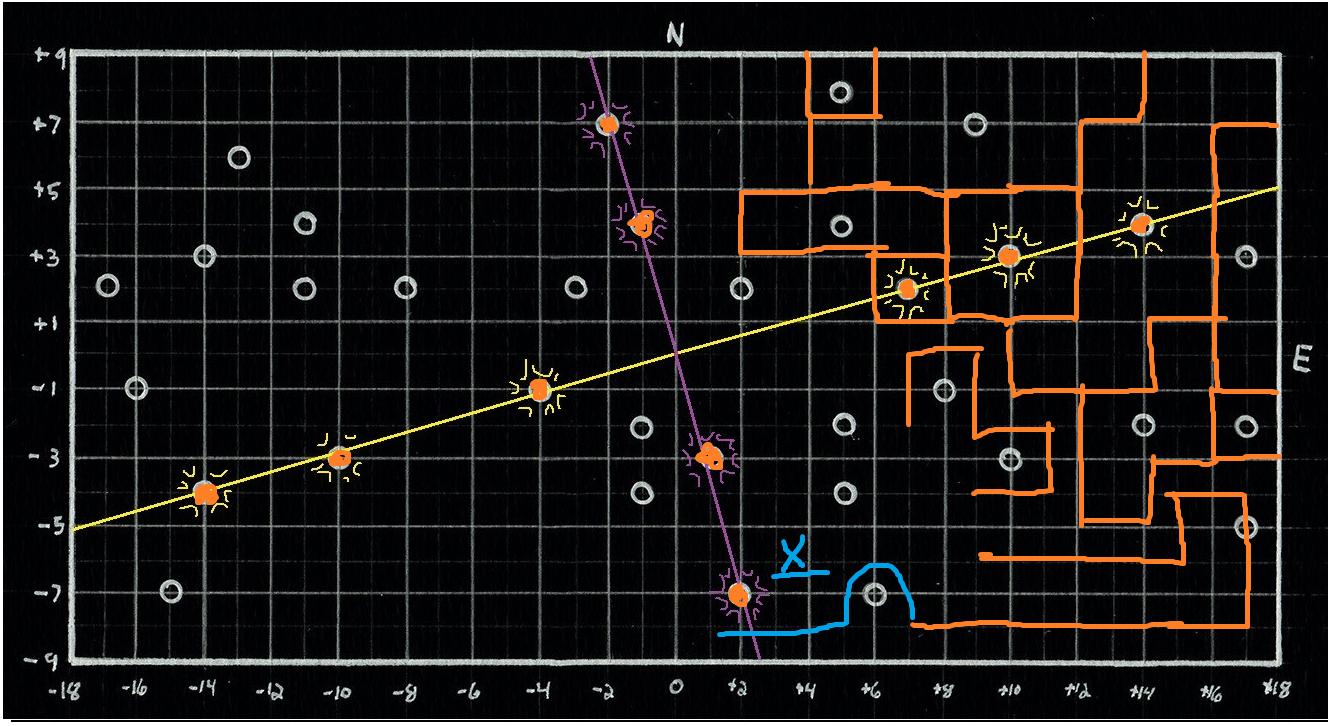
The other leads us to here.

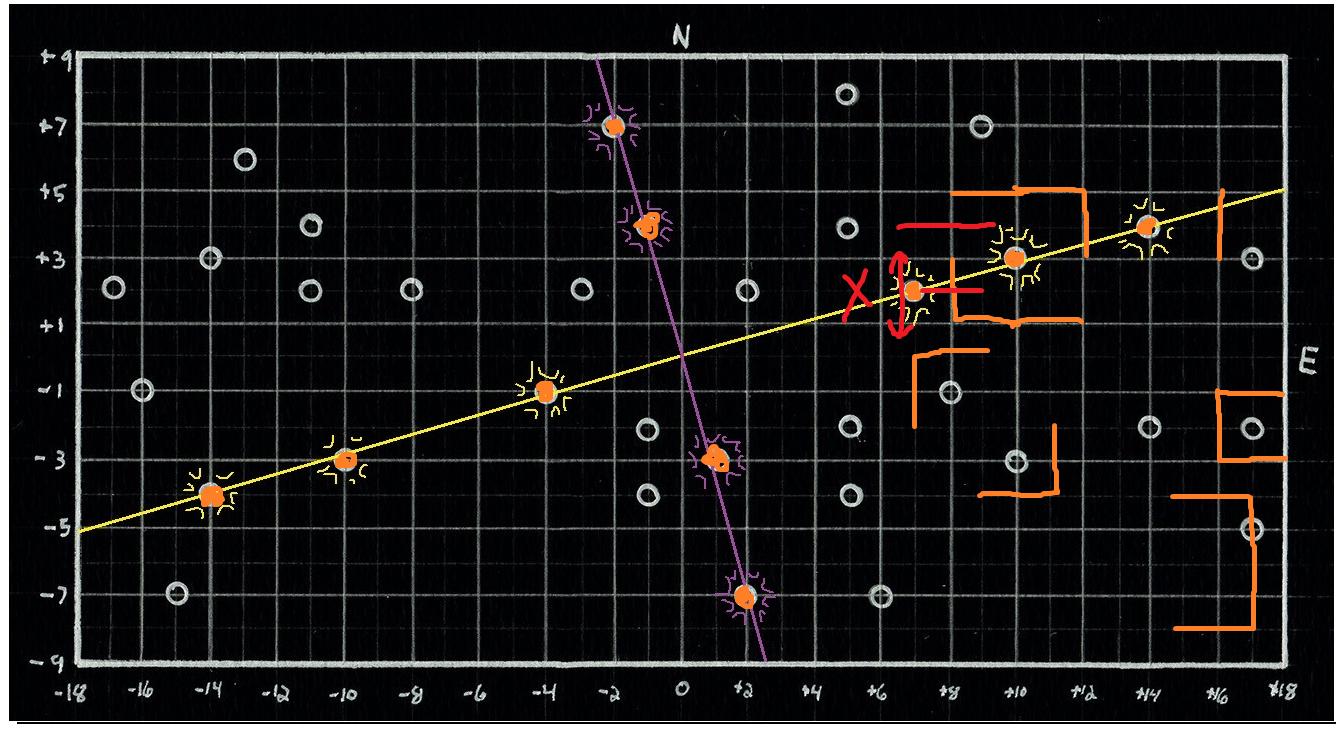
Neither end can go west from here, or else it enters S3E1 at an angle, which is bad. So they both turn ways. We enter S7E2 anyway at a better angle.

No galaxy can enter N0E5. So we're here now.

Two independent contradictions: 1) N7W2 is not a loop clue, or else N4W1 is a loop clue, and that would not work. 2) S2W1/S4W1 are not loop clues, or else S1E4 is a loop clue, and that would not work.
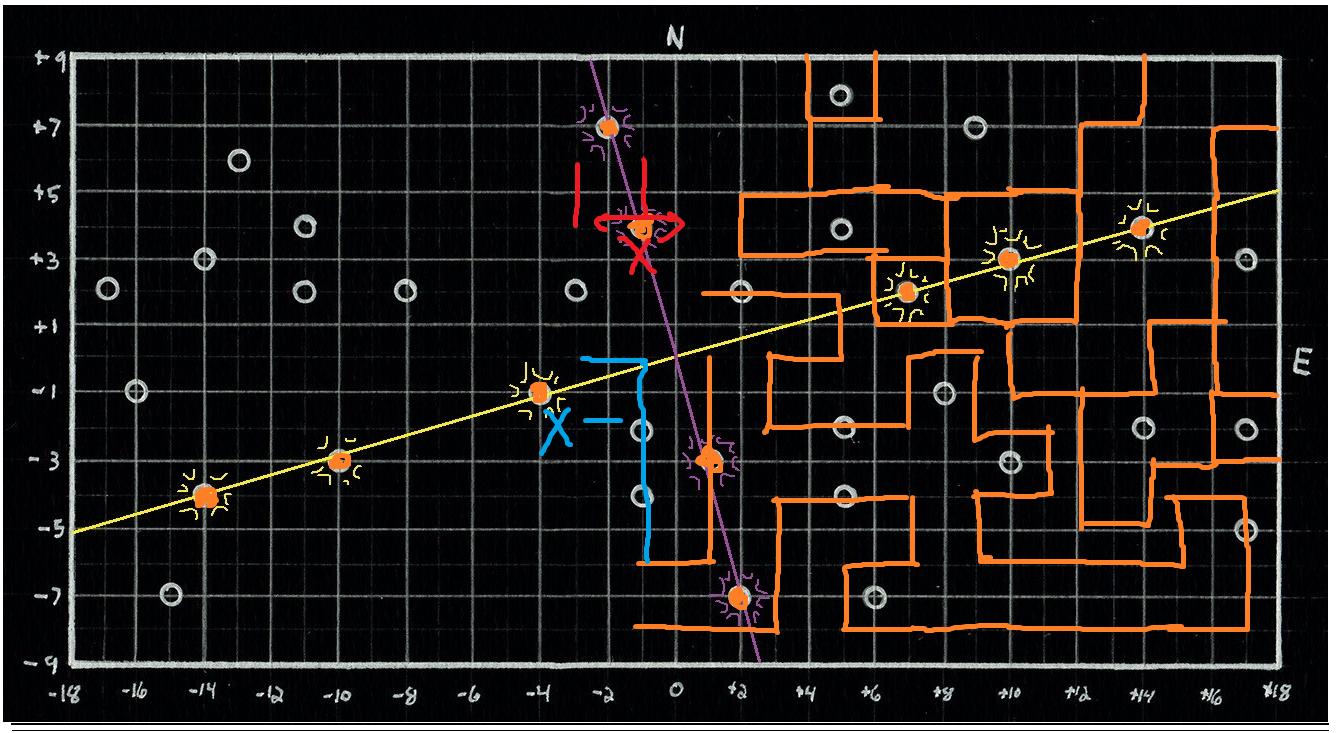 So both S2W1/S4W1 are one-cell galaxies.
So both S2W1/S4W1 are one-cell galaxies.
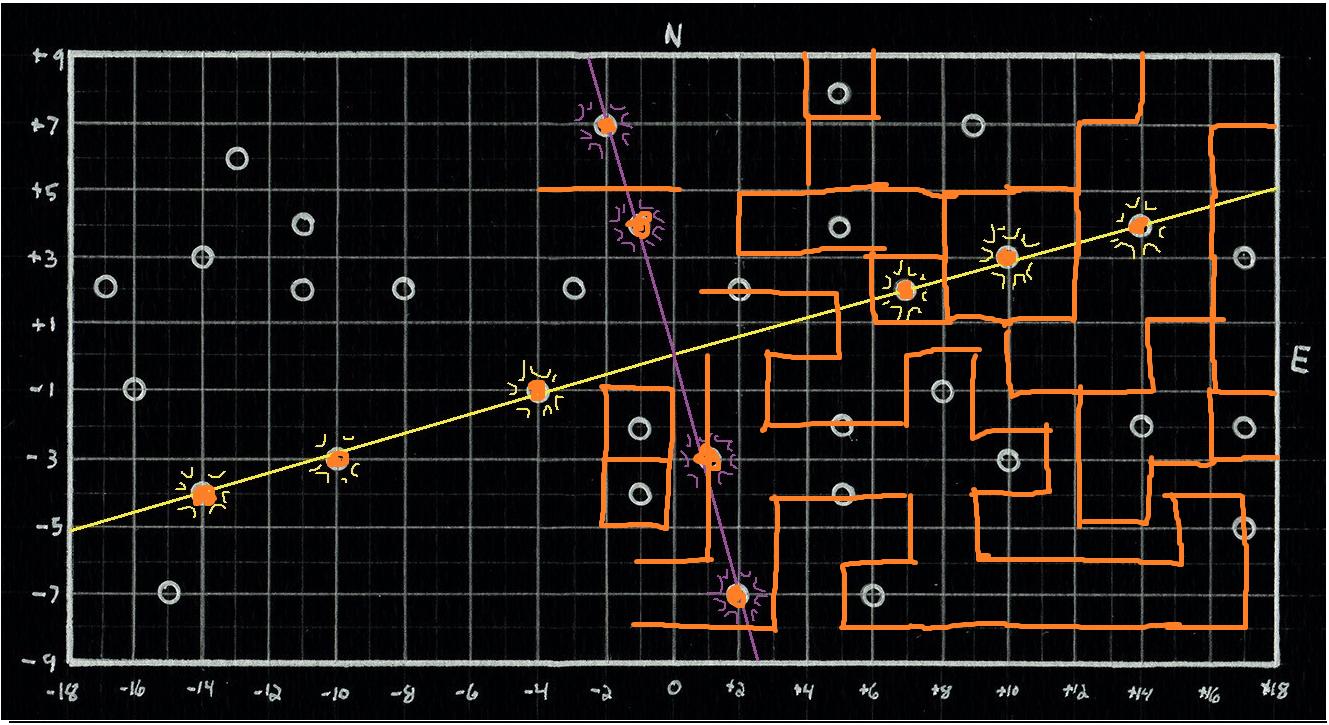 Likewise, N4W1 being a loop clue would not work as it would make S1E4 a loop clue in the wrong way.
Likewise, N4W1 being a loop clue would not work as it would make S1E4 a loop clue in the wrong way.
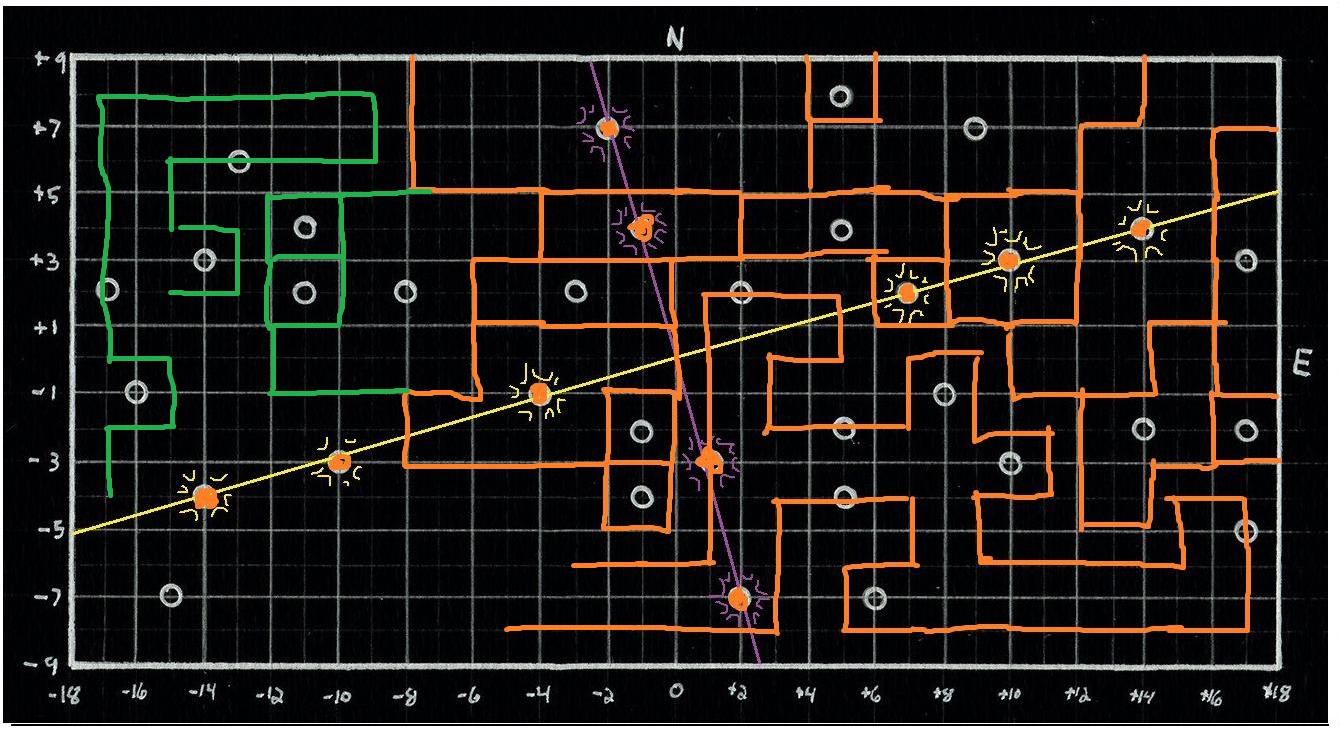
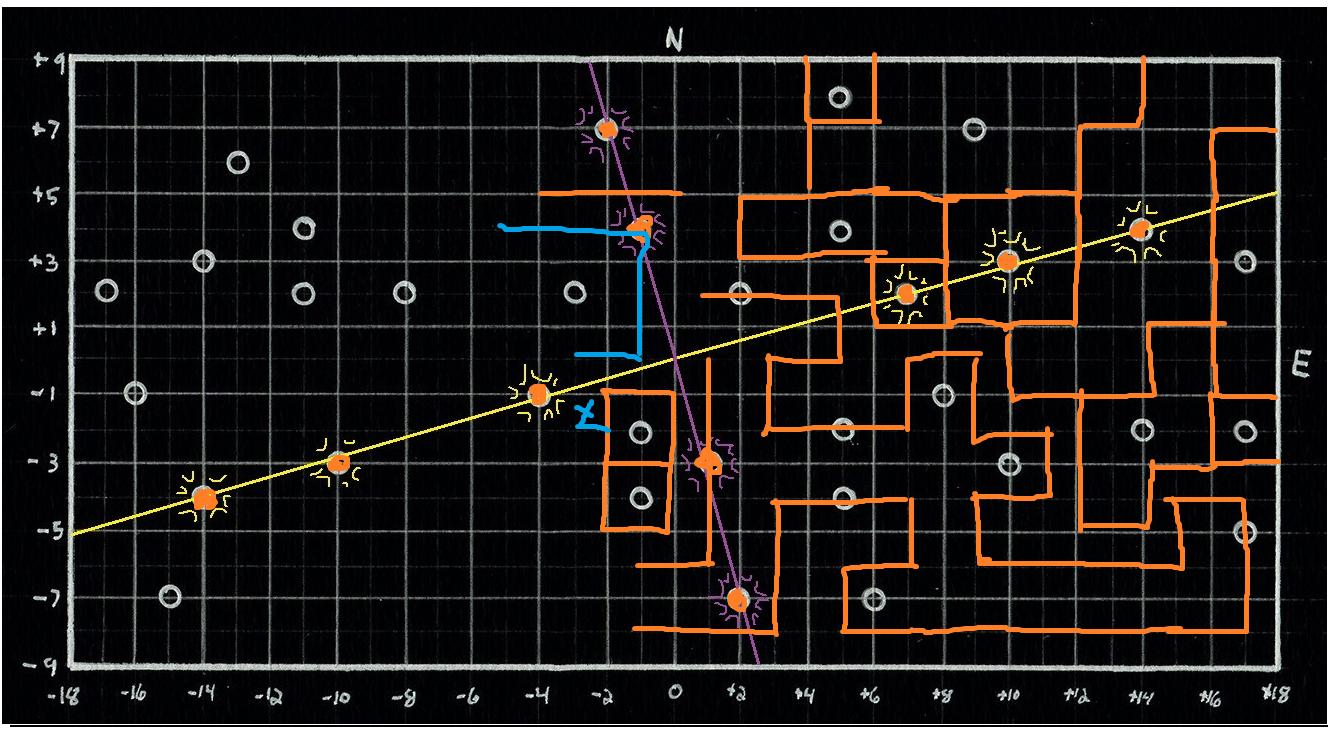 Since N4W1 is a galaxy, N4E1 is rendered unusable by a loop, so N4W1 annexes it. This also gets us the exact borders of galaxy N7W2, and resolves loop clue N2E3.
Since N4W1 is a galaxy, N4E1 is rendered unusable by a loop, so N4W1 annexes it. This also gets us the exact borders of galaxy N7W2, and resolves loop clue N2E3.
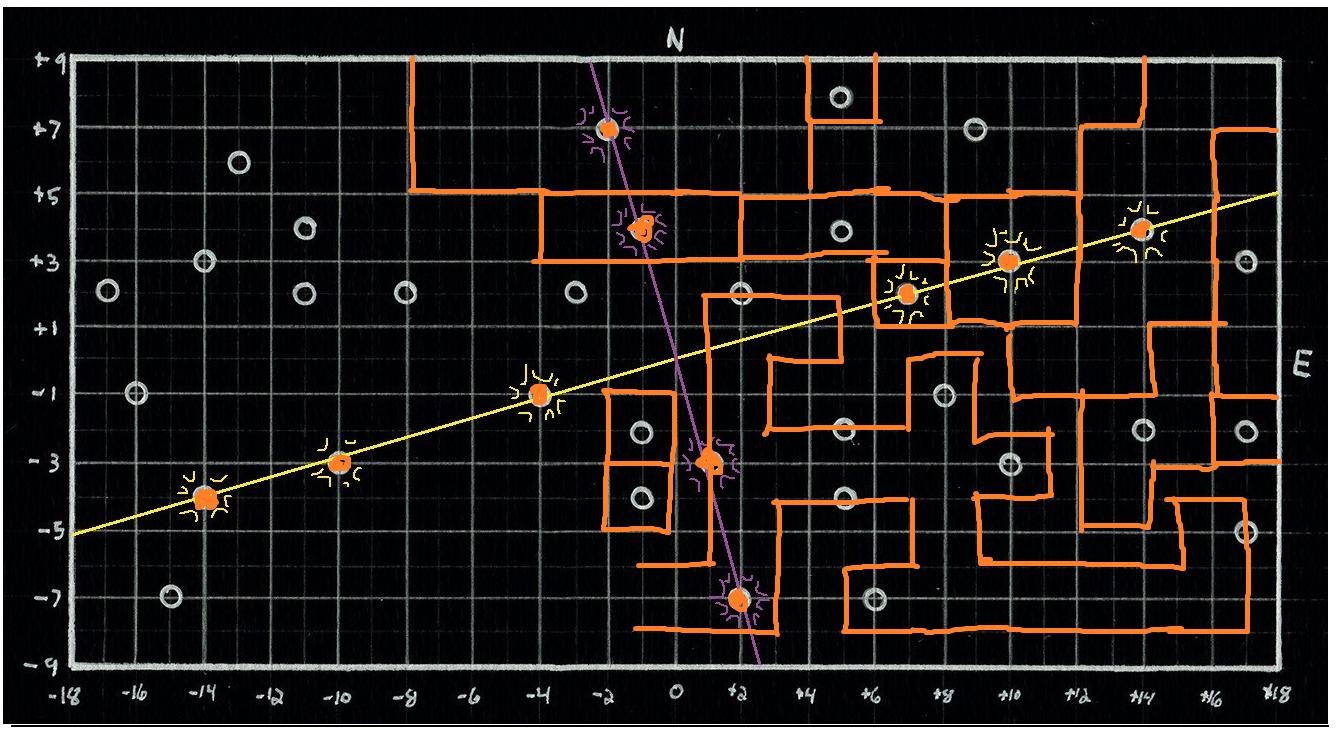
N0W1 and N2W1 are galaxy squares because - you guessed it! - S1W4 making a loop clue in the wrong way. This also means N2W3 is a galaxy.
I love bifurcating! Let's casework on the three cases these two galactic squares resolve.
Nope, they don't split 1 for 1.
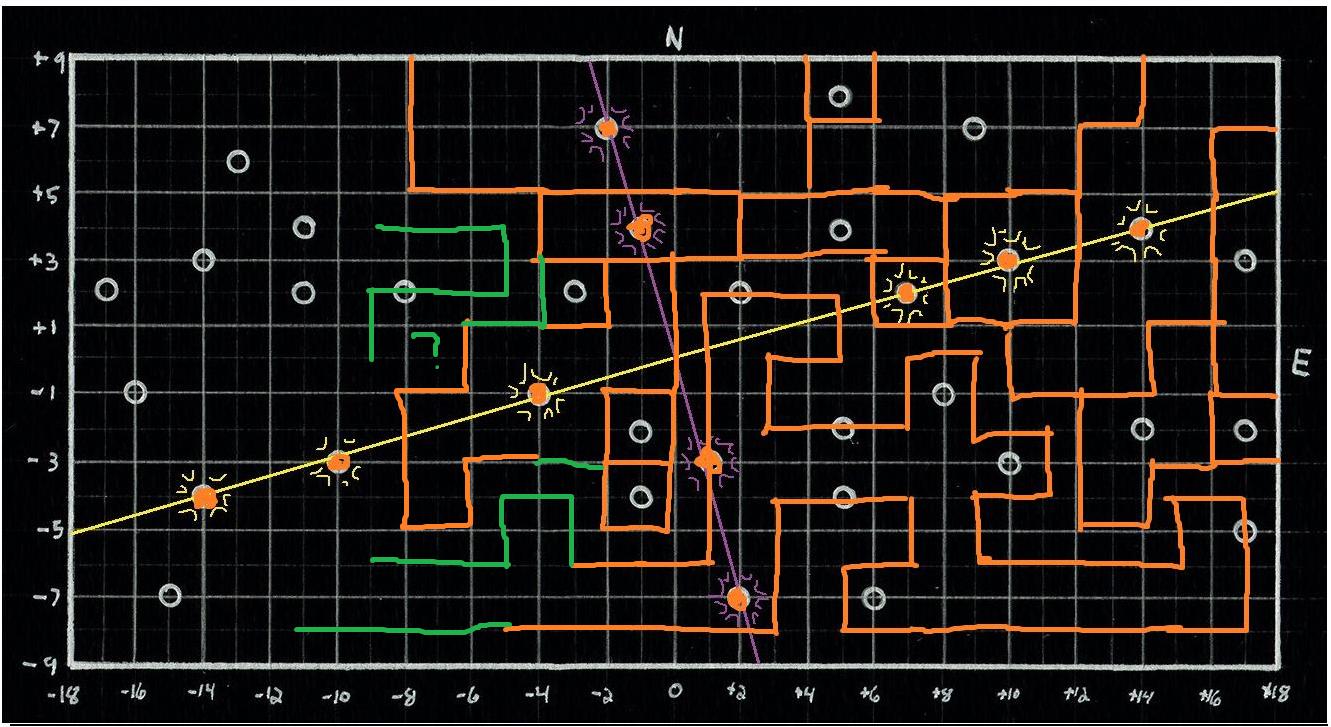
I guess not here either.
OK, now the most ridiculous deduction yet. As in, I did not actually do this when solving. I am just invoking this deduction because I don't want to screenshot so many things. (Fun fact: there are 56 pictures right now and counting)
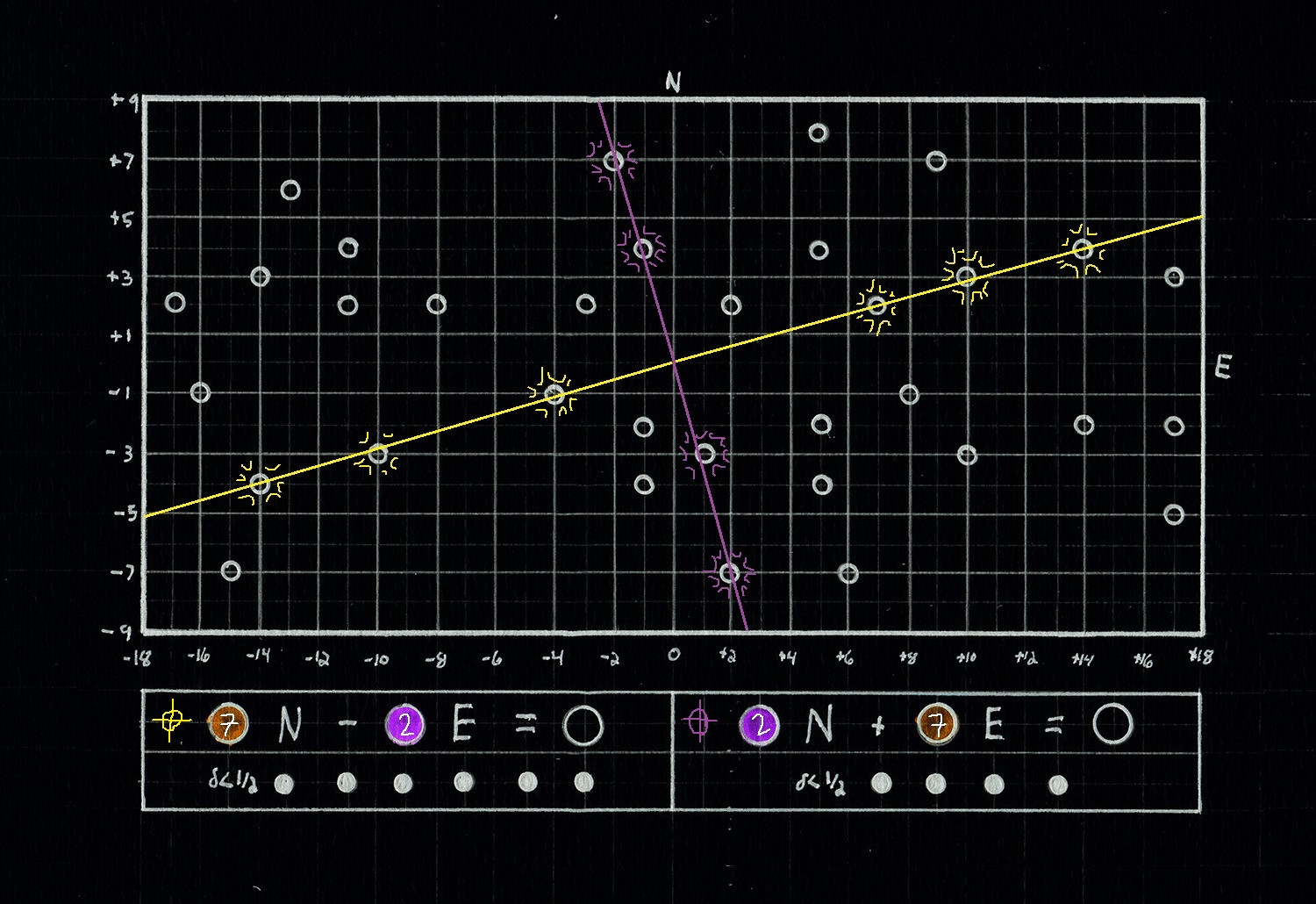
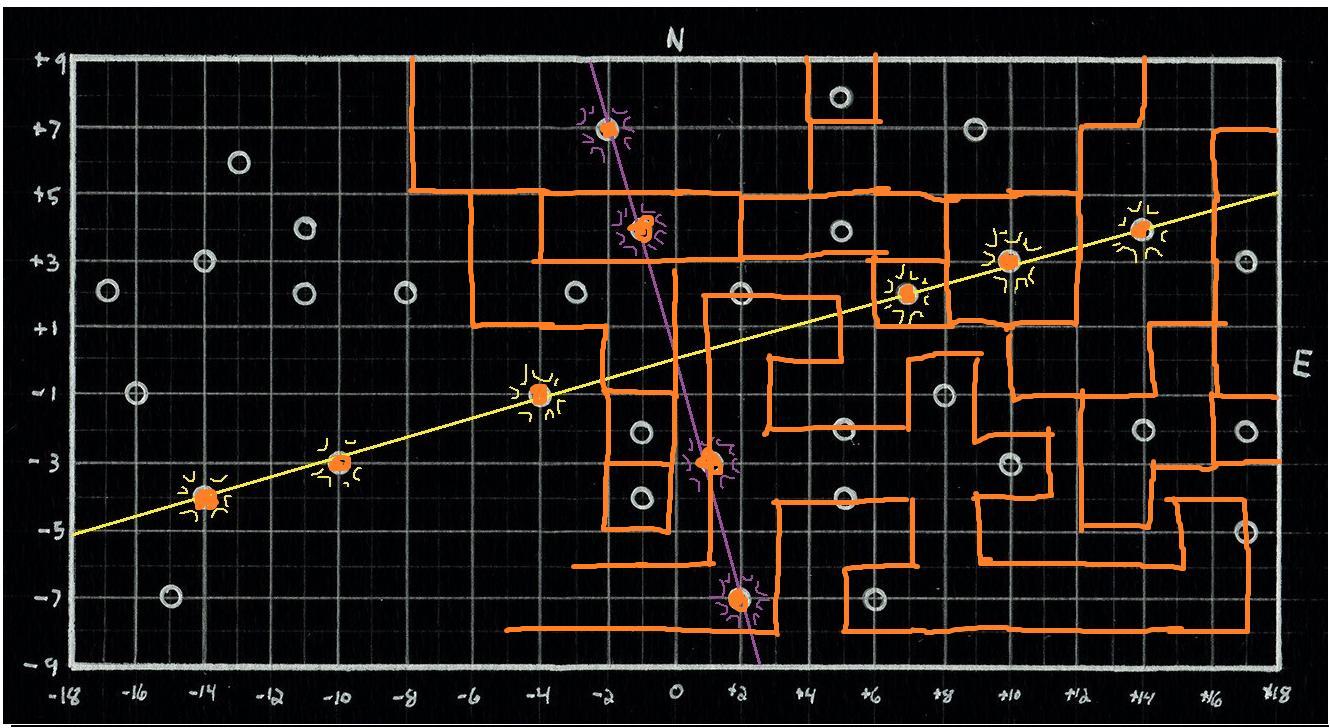
So, as a result of the loop condition, if we color the squares in a checkerboard fashion, the loop will traverse an equal number of white and black squares. The only thing that can affect the parity are galaxies centered on a square. As of so far, there have been 8 galaxies, 4 of each color. There are 4 remaining circles on a square. if either N2W17/N6W13/N4W11 is a galaxy, then N2W11 is a galaxy as well (and the other two points mentioned are not galaxies).
So, suppose N2W17 is a galaxy. Then we have:
 A plural loop! Thus, N2W17 isn't a galaxy. Since that worked so well, let's assume N6W13 is a galaxy.
A plural loop! Thus, N2W17 isn't a galaxy. Since that worked so well, let's assume N6W13 is a galaxy.
Can't turn up.
Turning down instead is a bit more successful, but now we have a problem with the end at N0W13 - it can't turn onto S4W14, but if it turns onto S3W10, it must turn in from the west, but that ultimately leaves N0W11 unvisited.
 Suppose N6W13 was vertical. There are two ways to resolve N3W14. This one is the easier one to refute.
Suppose N6W13 was vertical. There are two ways to resolve N3W14. This one is the easier one to refute.
The harder one. Suppose N2W8 galaxies N8W9. Then we get a contradiction.
Otherwise we loop towards there, and must loop back to take N6W11.
And this contradicts, sadly. Fortunately we feel like we're pretty close now.

Surely N8W13 must be loopy?
If N6W15 goes west:
Plural loop! So N6W15 goes south and we get this. But we see that we galaxy one of the cell circles, and hence get a parity problem.
 What?? N8W13 isn't loopy??
What?? N8W13 isn't loopy??
Indeed. Turns out that there exists a galaxy that can accommodate N8W13. It's hard to find, but here it is:

There we go. The rest should be pretty straightforward. First do all of the loop deductions:
S2W13 must go right, the last of the deductions, and we can complete the loop thusly:
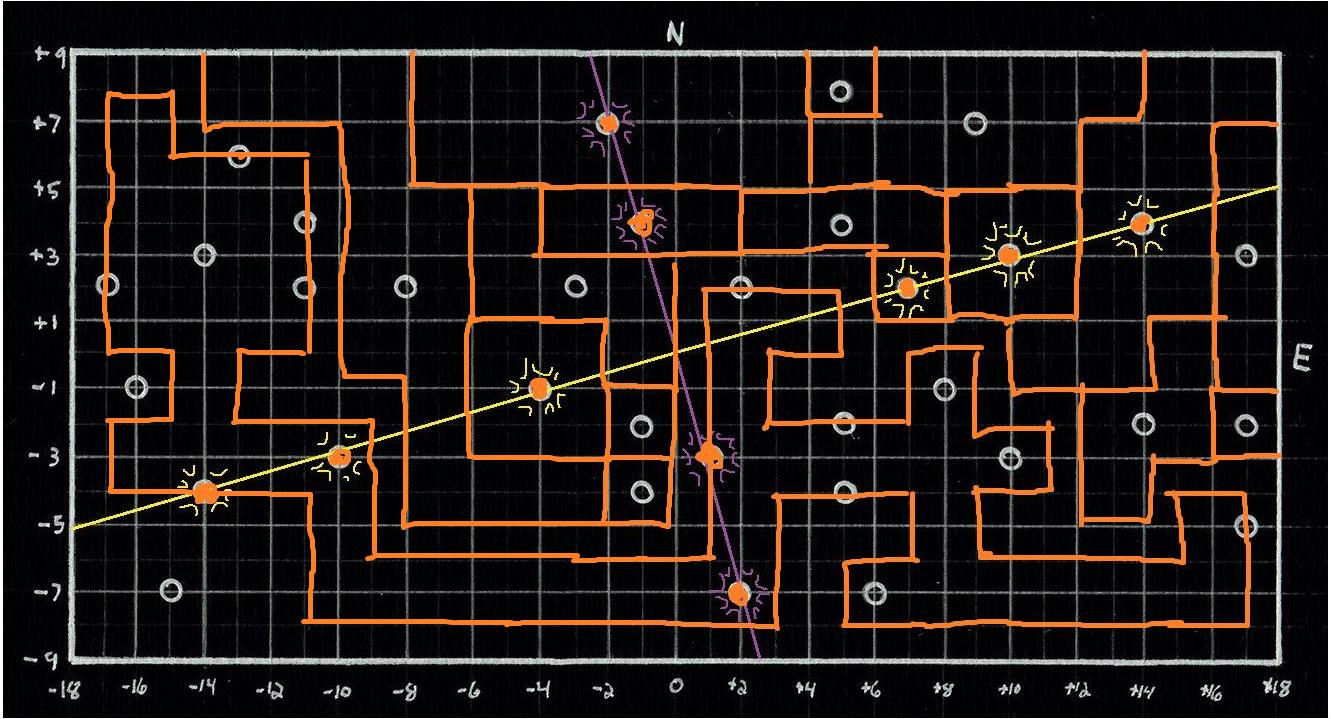 And we can finish up the galaxies as well, resulting in the final answer:
And we can finish up the galaxies as well, resulting in the final answer:

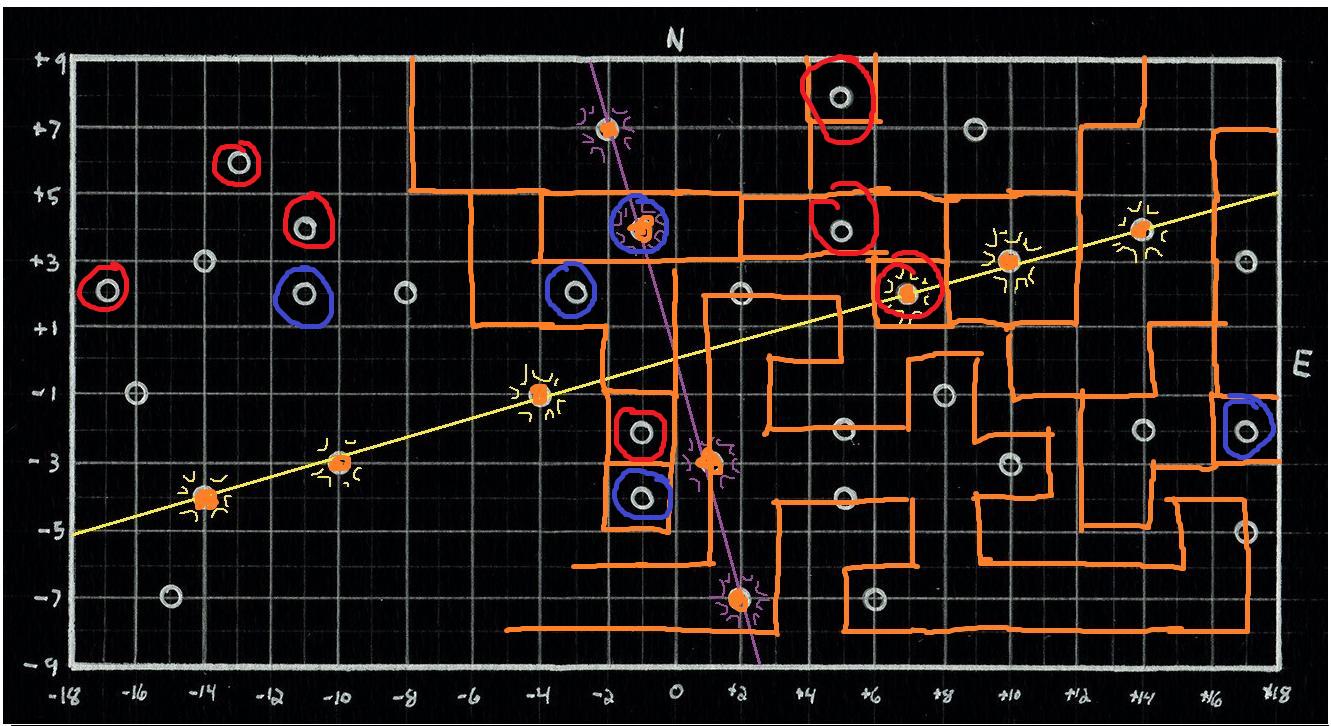
Here begins speculation
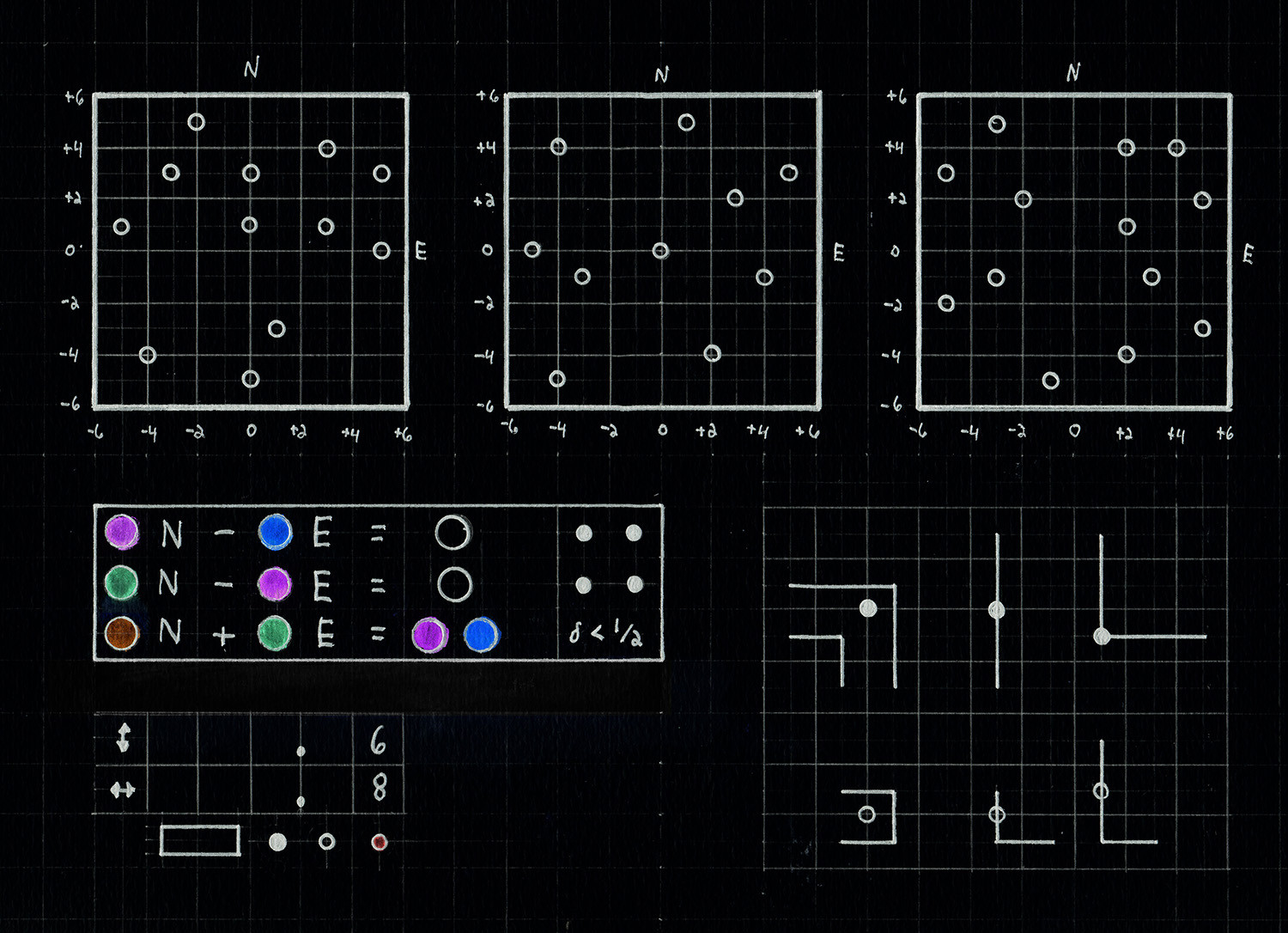
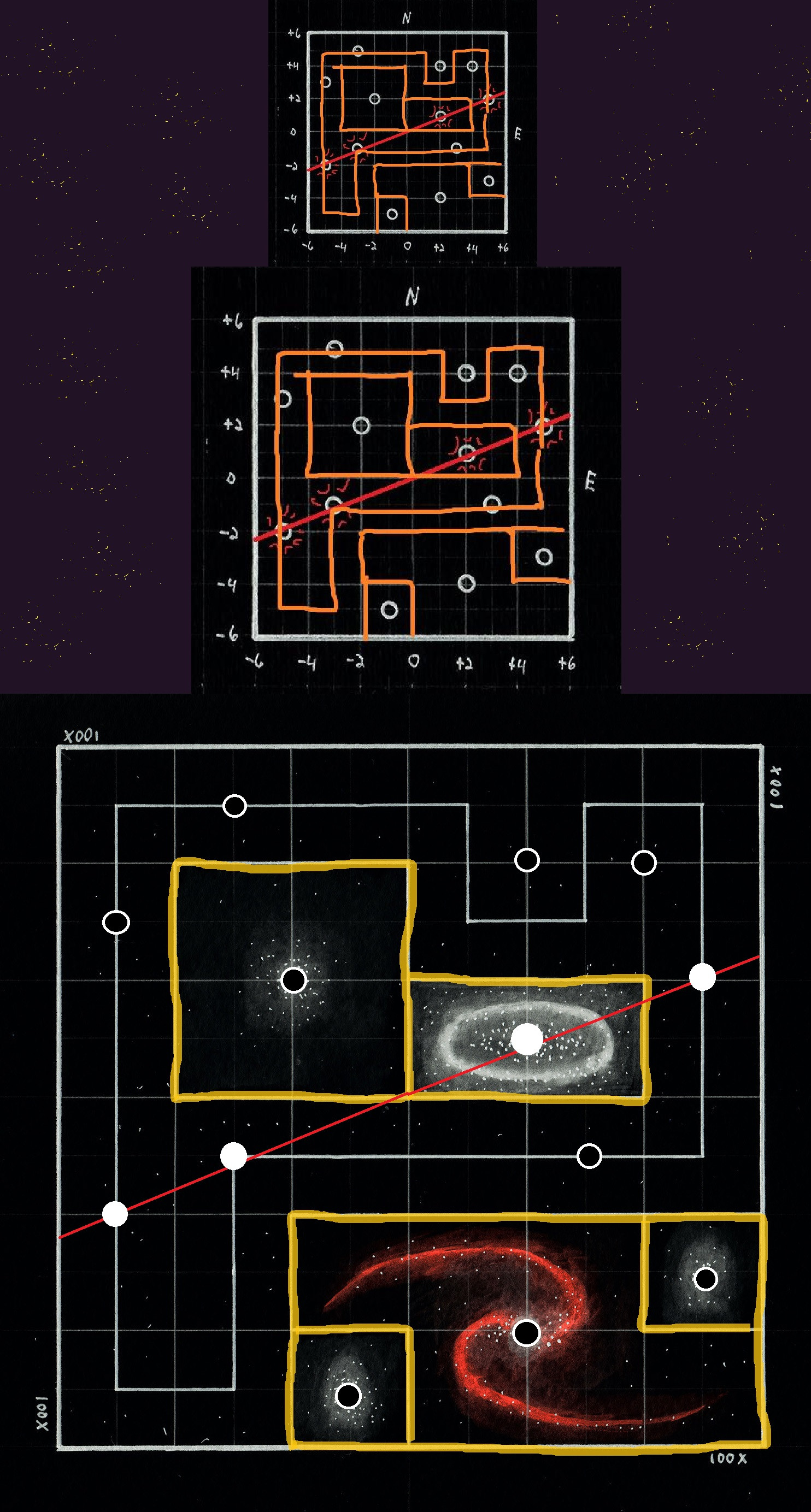
We still don't know where the guy was, but we still have some pieces remaining. An inventory:
Obviously we haven't used the solutions to the logic grids. In particular, the lighting up factor of the galaxies, so far, has been irrelevant, so that might play a role here.
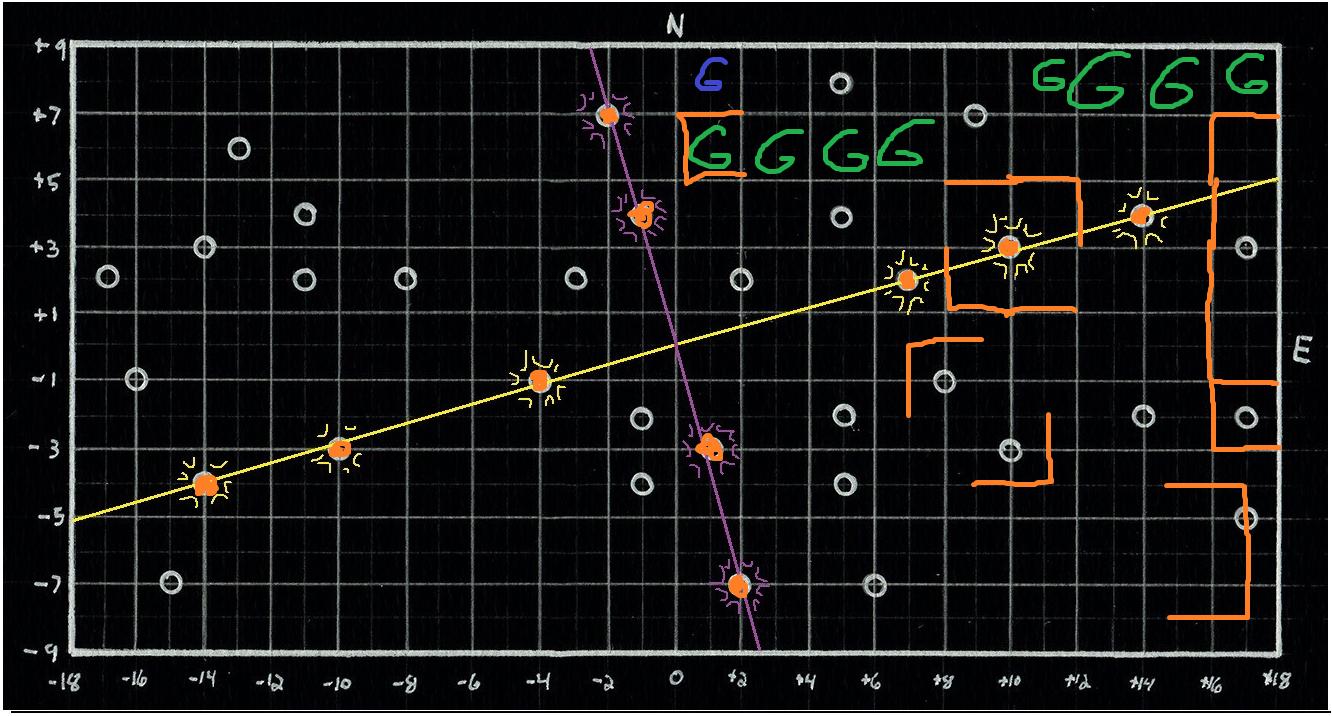
We have  this little dude. Probably get some lat/longs and get a location. The symbols underneath are indicative... of something. Maybe the rectangle indicates area of loop?
this little dude. Probably get some lat/longs and get a location. The symbols underneath are indicative... of something. Maybe the rectangle indicates area of loop?
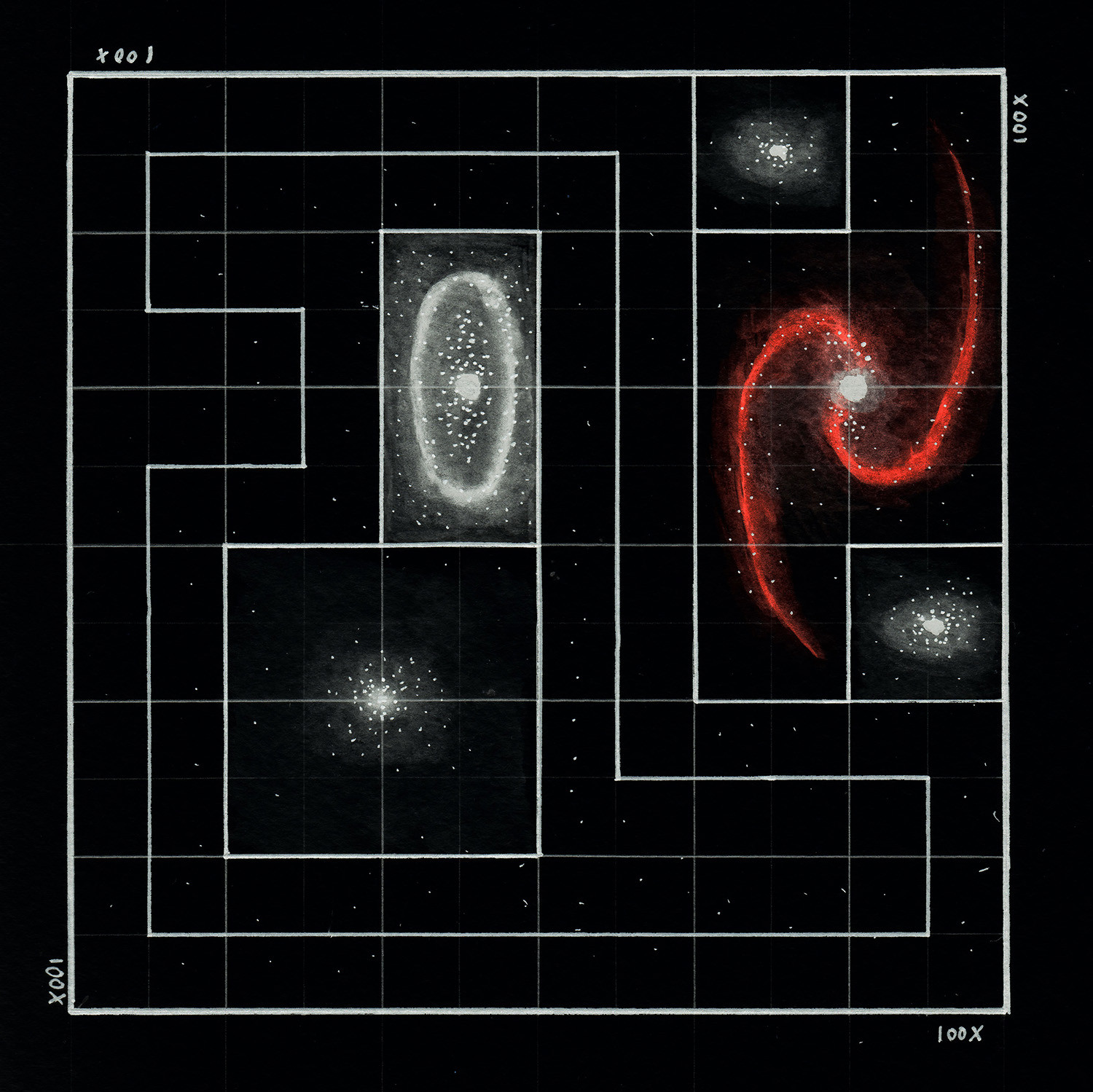
In particular one of these dots is red. That's weird. But the digits above are already filled, so maybe we don't have to worry about this.
But do you know what else is red? The galaxy in the example picture. Hmmm... What makes it special? Well, it is only 2-way rotationally symmetric, and perhaps it's the largest of its grid. Incidentally, the only thing special about the lit up galaxy in that grid is that it has a halo around it. But it's not sure where decoration ends and puzzle element begins.