A Tic-Tac-Toe variant with three marks - winning strategy and chances

Question

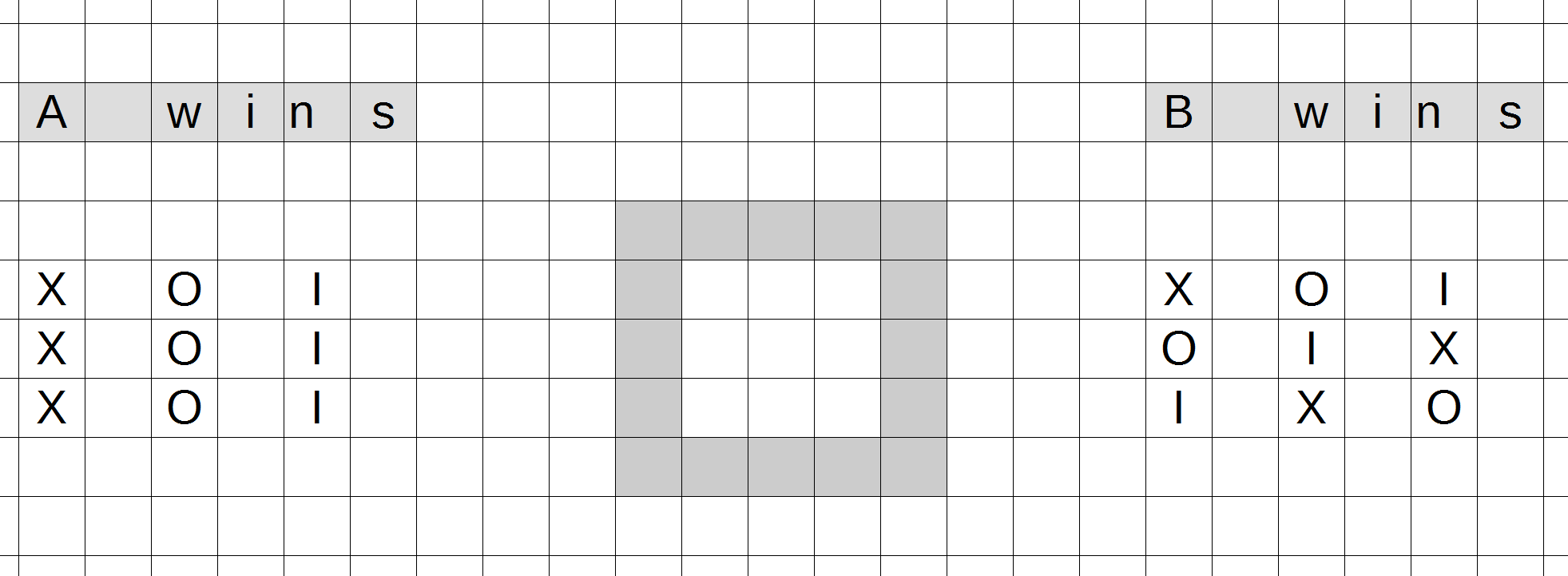
We start with a usual $3 \times 3$ grid, $3$ different marks (say, $X, O, I$) and $2$ players.

• Player $A$ has to make a vertical, horizontal or diagonal line made of $3$ identical marks (XXX, OOO or III).

• Player $B$ has to make a vertical, horizontal or diagonal line made of $3$ different marks (XOI, OXI, XIO, IOX, IXO or OIX).

If both players have a 'winning' position on the last step, the game is a draw.

Below is an illustration (only some separate examples of winning lines are shown for $A$ and $B$, it's not a full list neither it's an example of a final board).

Which player has better chances at winning, if they go first? If the other goes first?

Which player has an easier time forcing a draw in each case?

Are the winning/draw strategies for Players $A$ and $B$ fundamentally different?

Answer 1

Solution

The second player always wins.

---

Step-by-step deduction

If A plays first, then

B wins.

Proof: the crucial realisation is that B can always force A to play in a specific square. WLOG, say A's first move is an $X$.

• If A's first move is in a corner (WLOG, an $X$), B plays as follows:

  X   O   ?
  
  O   .   .
  
  ?   .   .

  where the ? marks denote the positions of A's subsequent forced moves (each of which can be either an $X$ or an $O$). Finally, B plays

  an $I$ in the centre to guarantee victory.

• If A's first move is in the middle of an edge (WLOG, an $X$), B plays as follows (first $O$, then $I$):

  I   .   .
  
  X   O   ?
  
  .   .   .

  where the ? mark denotes the position of A's forced move (either an $X$ or an $O$). At this point, A cannot prevent B both from completing the first column and from completing the leading diagonal.

• If A's first move is in the centre (WLOG, an $X$), B plays as follows (first $O$, then $I$):

  I   .   .
  
  O   X   ?
  
  .   .   .

  where the ? mark denotes the position of A's forced move (either an $X$ or an $O$). At this point, A cannot prevent B both from completing the first column and from completing the leading diagonal.

If B plays first, then

A wins (thanks @IvoBeckers for help with this part of the proof).

Proof: we can more or less adapt the same proof as used in the first half of the solution.

• If B's first move is in the centre or in the middle of an edge, then A can employ a strategy identical to B's strategy described above, except playing $X$ at every turn instead of $O$ or $I$, thus forcing B's hand in the same way as B forced A's when A went first.

• If B's first move is in a corner (WLOG, an $X$), A plays more $X$'s, first in the centre and then adjacent to the filled corner:

  X   X   .
  
  .   X   .
  
  .   .   ?

  where the ? mark denotes the position of B's forced move (either an $O$ or an $I$). At this point, B cannot prevent A both from completing the first row and from completing the second column.