14
$\begingroup$

The King has tired of watching the Queen swim in the Pool of Pawns. Help him return to his palace on h8 using as few moves as possible.

Only legal chess moves are allowed. Pawns are not promoted on the final rank, nor are they removed.

You can post your solutions in quasi-algebraic notation. For example, moving the queen from e6 to e7 can be notated as Qe6e7. Or you can post them as animations.

enter image description here

(Click on the image for a virtual board.)

Improve this question
$\endgroup$
2
  • $\begingroup$ Are pawns removed from the board when they reach the eighth row? $\endgroup$
    – GoodDeeds
    Commented Nov 29, 2016 at 5:18
  • $\begingroup$ Nope, they just sit there. $\endgroup$
    – DyingIsFun
    Commented Nov 29, 2016 at 5:18

3 Answers 3

Reset to default
8
$\begingroup$

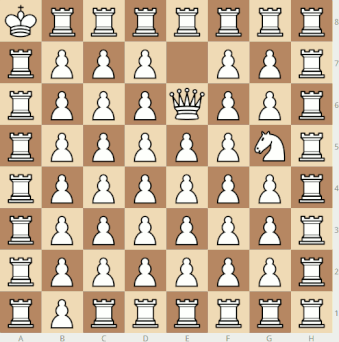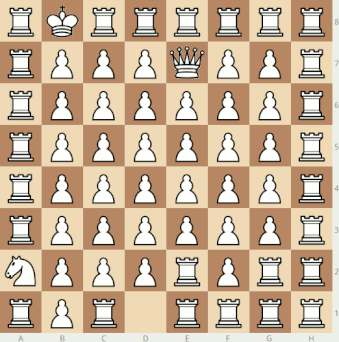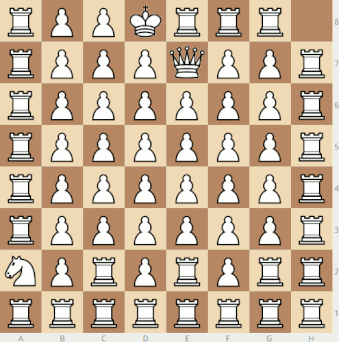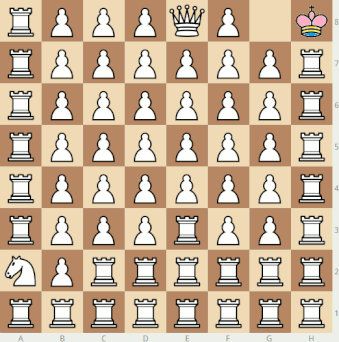
My solution has:

181 moves - Note that it is the same as Johnathan Allan's solution (see his for a gif of the solution), I made an arithmetic error doing it by hand and not checking with a computer (Don't believe me? Check the revision history!).

I will use P to denote pawn for nice formatting (sorry chess fans) and instead of writing multi-move chains of sliding the same type of piece in a line I will just put the start of the chain, the end of the chain and the number of moves between in brackets.

The key part is the start:

Rh3-e7 (9 moves) For example, this denotes Re8-e7, Rf8-e8, Rg8-f8, etc. round to Rh3-h4.
Ng5-e3 (1 move)
Pg2-g5 (3 moves)
Rg1-g2 (1 move)
Nh3-g1 (1 move)
Re7-h3 (9 moves)
Qe6-e7 (1 move)
Pe2-e6 (4 moves)
Ng1-e2 (1 move)
Rc1-g1 (4 moves)
Ne2-c1 (1 move)
Rb8-e2 (17 moves)

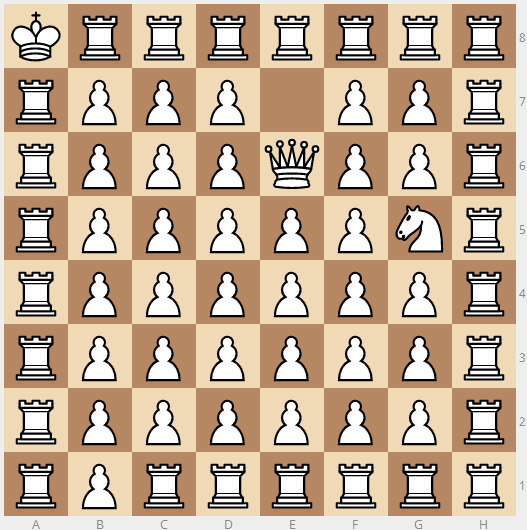
This gives:

The position shown here after 52 moves.
Midway...

This is the brute force part:

Ka8-b8 (1 move)
Ra2-a8 (6 moves)
Nc1-a2 (1 move)
Rc8-c1 (17 moves)
Kb8-c8 (1 move)
Pb1-b8 (7 moves)
Rd8-b1 (17 moves)
Kc8-d8 (1 move)
Pc2-c8 (6 moves)
Re8-c2 (16 moves)
Kd8-e8 (1 move)
Pd2-d8 (6 moves)
Rf8-d2 (14 moves)
Ke8-f8 (1 move)
Qe7-e8 (1 move)
Pe3-e7 (4 moves)
Rg8-e3 (13 moves)
Kf8-g8 (1 move)
Pf2-f8 (6 moves)
Rh8-f2 (8 moves - note the shortcut via g2)
Kg8-h8 (1 move)

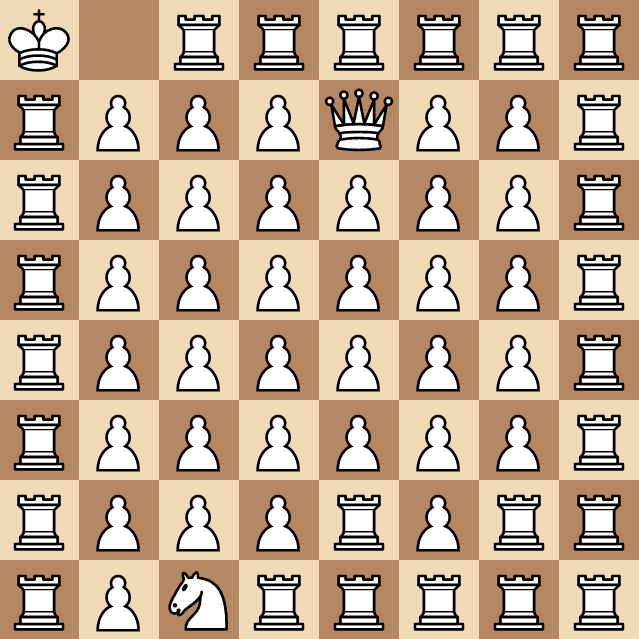
This gives the final position of:

This! (after another 129 moves)
End!

Animated solution credits to Johnathan Allan:

Animated solution

Improve this answer
$\endgroup$
1
  • $\begingroup$ It seems like you deserve the green check, since you posted first! When I checked your solution by hand, I didn't notice it was the same as @JonathanAllan's, and your (incorrect) count of 208 moves further prevented me from noticing... It was good that you spotted this. $\endgroup$
    – DyingIsFun
    Commented Nov 29, 2016 at 20:52
6
$\begingroup$

I have a solution that takes

$181$ moves

Animated solution

Improve this answer
$\endgroup$
6
  • $\begingroup$ how do you prepare this kind of gif files? :D $\endgroup$
    – Oray
    Commented Nov 29, 2016 at 18:34
  • $\begingroup$ Thanks @GentlePurpleRain - I couldn't seem to compress it enough without making it tiny. $\endgroup$
    – Jonathan Allan
    Commented Nov 29, 2016 at 19:03
  • 2
    $\begingroup$ @Oray - I used Gimp - but my skills are not that great. $\endgroup$
    – Jonathan Allan
    Commented Nov 29, 2016 at 19:04
  • $\begingroup$ @Oray I used this and this. I think they're related sites (I got to one by following a link from the other). $\endgroup$
    – GentlePurpleRain
    Commented Nov 29, 2016 at 19:22
  • $\begingroup$ @boboquack I had not looked at yours, but I see yours now has the same count. Good work! $\endgroup$
    – Jonathan Allan
    Commented Nov 30, 2016 at 7:26
-2
$\begingroup$

19 moves:

Re7  Rfe8  f8    Qf7   Ne6 
Rg5  R6h5  R7h6  R8h7  Rgh8
Qg8  f7    f6    Rgf5  Rhg5
R6h5 R7h6  R8h7  Qh8
Improve this answer
$\endgroup$
3
  • 3
    $\begingroup$ You want to move the King (♔) to h8, not the Queen (♕). Maybe I should add a line in the instructions about which pieces are which. $\endgroup$
    – DyingIsFun
    Commented Nov 29, 2016 at 7:14
  • 1
    $\begingroup$ @BeastlyGerbil It is a wrong answer but it is an answer $\endgroup$
    – paparazzo
    Commented Nov 29, 2016 at 8:13
  • 1
    $\begingroup$ I thought the queen was the 'him', sorry $\endgroup$
    – CyberianRat
    Commented Nov 29, 2016 at 21:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.