7
$\begingroup$

Is it possible to completely fill the infinite tetris field $\mathbb Z^2$ with tetrominoes such that no tetromino borders another one of the same type?

Assume that two tetromiones border each other if their blocks (squares) share at least one edge, not just one vertex.

Improve this question
$\endgroup$
5
  • $\begingroup$ For me the remark about the rotation is a little bit vague. Do you mean that two tetrominoes in the tessellation, which are rotations of each other, are not allowed to touch? $\endgroup$
    – elias
    Commented Aug 17, 2016 at 6:27
  • $\begingroup$ @elias Yes. I removed that note now. I guess it should be implicit to any tetris player. $\endgroup$
    – GOTO 0
    Commented Aug 17, 2016 at 6:41
  • $\begingroup$ @GOTO0, if you are still around you should probably accept one or other of the answers here unless there's something nonobviously wrong with them. $\endgroup$
    – Gareth McCaughan ♦
    Commented Dec 1, 2016 at 20:00
  • $\begingroup$ @GarethMcCaughan Sorry, I haven't been around here for a while. $\endgroup$
    – GOTO 0
    Commented Dec 1, 2016 at 20:55
  • $\begingroup$ Perfectly understandable. I see you've done it now -- thanks! $\endgroup$
    – Gareth McCaughan ♦
    Commented Dec 1, 2016 at 21:29

2 Answers 2

Reset to default
9
$\begingroup$

Yes it is, here is a block that can be repeated vertically and horizontally

Shown here

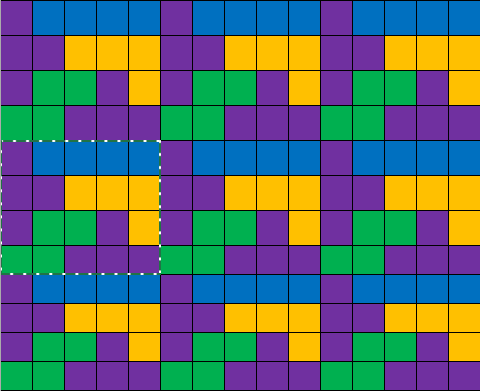
enter image description here

and in a tile formation

enter image description here

Interpreted the even when rotated part of the question as just stating that a tetromino cannot touch the same colour tetromino regardless of orientation.

Improve this answer
$\endgroup$
6
$\begingroup$

A possible solution can be reached by building

two different 2-row pattern, which don't have a common tetromino, and inside themselves do not have touching tetrominoes of the same type. A pair of possible patterns of this kind is:

LLLJJJ
LIIIIJ
and

 SSTZZTTT
SSTTTZZT
which both can be repeated infinite times to 'fill' a $2\times \mathbb Z$ part of the plane. Placing them below each other in an interchanging order you get a tessellation of the plane. Any of the 2-row elements can be translated horizontally, so this already gives infinite solutions.

For clarification, here is a picture.

enter image description here
In this one, I enhanced the first 2-row pattern with the square tetromino, so now each type is used in the tessellation. Also this version has a $4\times8$ tiles repetition. By putting this kind of blocks next to each other (both horizontally and vertically), you get a tiling which suits the criteria.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Sorry if my solution was not clear. I suggested building one 2-row-pattern of the first kind, then below it the other one. I will include a picture for better understanding. As the two patterns don't share a common tetromino, you can put these different $2\times\mathbb Z$ patterns below each other with arbitrary horizontal translation without same types of tetrominoes touching. $\endgroup$
    – elias
    Commented Aug 17, 2016 at 7:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.