9
$\begingroup$

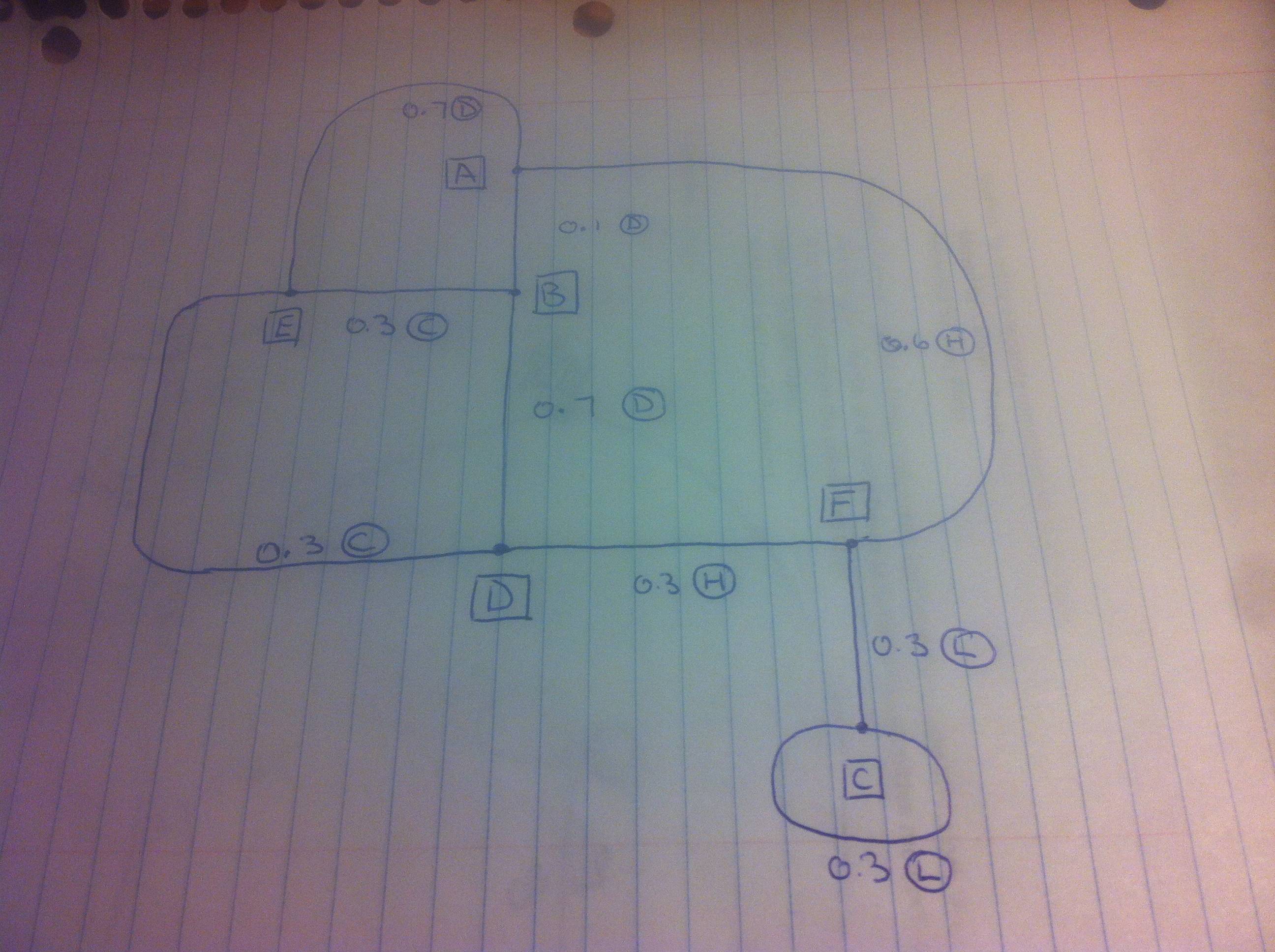
On a recent amble, I happened upon a sign $\small \raise2mu ( {\normalsize\sf\color{#d90} A} \raise2mu )$ that seemed to indicate a mistake — or— a mystery.

signs A,B,C,D,E,F | click for a slightly obscured picture of the actual sign A

Really, sign $\normalsize\sf\color{#d90} A$, really?   Dutch Flat Trail is in both directions and on the way to itself?   This turned out to signify both, a mistake and a mystery!

The mistake was not in the information but in the design of its portrayal. The numbers shown are true travel distances from these signs, although separated unhelpfully from additional information not shown. (Distances were originally in miles, incidentally, but this version is not locali${\kern2mu \sf\scriptsize \rlap{ \raise8.5mu s } \raise-2mu z \kern2.5mu}$ed.)

The mystery is: What information is missing?

Spawned puzzle: What sequence of signs describes the longest non-overlapping trail route?

Start at the park entrance.
• The sign there differs from the others in a reasonable way.
• All signs are at three-path ⊥-shaped junctions, as shown by arrows, of two or three trails.
• No sign may be visited more than once.

Every junction is represented by its sign above, so you won't need to enjoy exploring the park in order to figure out the layout of its trails or the meanings of these distances. Trails meander and do not obey geodesic constraints such as triangle inequality.

This requires no electronic technology to solve and is just a puzzlingly presented example of an actual public-park sign-design puzzle solution gone awry the wild. Every park's layout is already like a puzzle whose clues are trail signs, which many visitors find puzzling enough even when they are sensibly designed. I really did have to figure out what these meant-to-be-helpful signs really meant.

Improve this question
$\endgroup$
8
  • 2
    $\begingroup$ My first thought was that the numbers are mile markers instead of distances (i.e. points of interest labeled by their distance from the beginning of the trail, not from the sign's location); but that still doesn't explain how the same point of interest could be in two opposite directions from the same point! $\endgroup$
    – 2012rcampion
    Commented Jul 2, 2016 at 2:46
  • 1
    $\begingroup$ that's entirely possible if there are two paths you can go by. $\endgroup$
    – Jasen
    Commented Jul 2, 2016 at 11:02
  • 1
    $\begingroup$ +1 for being based on something real and for reminding me of hiking trails. $\endgroup$
    – MackTuesday
    Commented Jul 2, 2016 at 20:00
  • $\begingroup$ Your hunch about mileposts contains a subtly crucial insight, @2012rcampion, but the puzzle statement now clarifies that these are indeed ambling distances from the signs. $\endgroup$
    – humn
    Commented Jul 2, 2016 at 22:38
  • 1
    $\begingroup$ This was a fabulous puzzle. $\endgroup$
    – LeppyR64
    Commented Jan 25, 2017 at 14:57

4 Answers 4

Reset to default
7
+50
$\begingroup$

How to Read the Signs

Each sign represents an intersection with three directions.

Dutch Flat Trail .7 ^
Canada Trail .3 <-
Heron Trail .3 ->

This means that

if one walks to the right they will be walking on Heron Trail. In 0.3 units, another intersection will be reached. Walk to the left will be on Canada trail and in 0.3 units an intersection will be reached. Walk straight and in 0.7 units an intersection will be reached.

The Confusion

One sign (A) has the following instructions:

Dutch Flat Trail .1 ->
Dutch Flat Trail .7 ->

Walking right, in 0.1 units an intersection will be reached. The second sign in the same direction indicates that the shortest path to the entrance is 0.7 units.

The missing piece of information is whether the sign points to the entrance or an intersection.

This extra information is applied to:

Signs A, B, and C.
The reason that extra distances it isn't listed for E and F is that their shortest paths are direct edges to the entrance and therefore an extra sign is not needed.

Sum it Up

The entrance is

D because it is the only one at the head of three paths.
Map
The letters in the squares are the sign posts. The letters in the circles are the trail names.

The longest non-overlapping path from the entrance is:

D -> B (0.7)
B -> E (0.3)
E -> A (0.7)
A -> F (0.6)
F -> C (0.3)
Total = 2.6 units
Which confirms Gareth McCaughan's Answer

Bonus

Thanks to @2012rcampion, I found your sign.
https://goo.gl/maps/AEEYZT9dDx12

Improve this answer
$\endgroup$
2
  • $\begingroup$ Excellent! Your interpretation of the secondary numbers makes more sense than either of the ones I came up with. Your map happens to be identical to mine (aside from details of layout) but unlike me you actually figured out why it's right :-). $\endgroup$
    – Gareth McCaughan ♦
    Commented Jul 6, 2016 at 19:07
  • $\begingroup$ And your longest path has been validated, @GarethMcCaughan. I think there are 3 effectively-identical possible layouts in all, if you picture the whole thing on a sphere that could be punctured within one of 3 different loops that include the entrance. (2 more layouts, one with a fun inside-out interpretation for "lollipop," isolate the entrance from the outside world.) $\endgroup$
    – humn
    Commented Jul 6, 2016 at 21:55
7
$\begingroup$

I'm not sure exactly what the mystery really is here, because these signs don't seem particularly confusing to me. (Which doesn't at all mean that I've interpreted them correctly; but my problem is "too many interpretations" rather than "no interpretations".)

As I understand it, the challenge is to explain how it can make sense for one sign to point to the same thing in two opposite directions, or at two distances in the same direction. Here are two (to me) plausible ways it can happen. First:

Something like "Dutch Flat Trail .7" means "if you go this way, the next new thing you will reach is the Dutch Flat Trail in 0.7 miles". The DFT is not a single point, it is a meandering path, so there is no contradiction in being able to get to different bits of it by going in different directions. When there are two of these pointing in the same direction, as e.g. at the bottom of sign A, it means "If you go this way, the first new thing you will reach is (a junction with) the DFT in 0.1 miles; the next is (a junction with) the DFT in 0.7 miles". Again, no contradiction, it just means that the DFT crosses the path more than once.

Second:

Something like "Dutch Flat Trail .7" means "if you go this way, you will be on the Dutch Flat Trail, and the next point of interest is 0.7 miles along it". So, again, no contradiction in having two of these in opposite directions; indeed, if going to the right means you're on the DFT then you'd expect that going to the left also does. When there are two pointing in the same direction, as e.g. at the bottom of sign A, it means there are two points of interest nearby in that direction (perhaps they list all up to 1 mile).

We could distinguish between these

if there were a sign with two different trails named in the same direction; that would be consistent with the first possibility but not with the second.

We don't have that particular clue. My feeling is that the second option would be more plausible if it weren't for the cases where the same trail is named twice in the same direction, but that given that the first is a bit more likely.

I can't find many pictures of posts from the actual site online, but the ones I have found seem to favour the second explanation.

There's a further subdivision of options: in either of the two cases above, having two distances a,b listed in the same direction could mean that the next-but-one point is at distance b or at distance a+b.

As for the puzzle here, with the constraint that the junctions shown here are all the junctions in the park (as indicated by humn's comment below), it seems to require the second variant of the second option above. I'm having trouble fitting everything together correctly and need to get some sleep, but for now I offer the following tentative answer which might be right even if some bits of my scribbled map are wrong:

I think D (with different trail names in two opposite directions) is probably the entrance. Then perhaps one can go D [0.7 DFT] B [0.3 CT] E [0.7 DFT] A [0.6 HT] F [0.3 LL] C for a total distance of 0.7+0.3+0.7+0.6+0.3 = 2.6 miles.

Improve this answer
$\endgroup$
8
  • $\begingroup$ I've only found one interpretation and map where these signs represent the entire layout. The interpretation from the real park is genuinely represented here but all signs are fabricated except for the part of sign A that is shown in the picture here. $\endgroup$
    – humn
    Commented Jul 3, 2016 at 2:31
  • $\begingroup$ Yes, I think you're right if the signs are meant to be the entire layout. Working on that. I notice that my second option actually has two different interpretations, one of which appears to be the right one for your map. $\endgroup$
    – Gareth McCaughan ♦
    Commented Jul 3, 2016 at 2:33
  • $\begingroup$ ... hmm, actually I can't make either interpretation quite work. Maybe I should go to bed instead since it's 3.30am local time... $\endgroup$
    – Gareth McCaughan ♦
    Commented Jul 3, 2016 at 2:35
  • $\begingroup$ I thought I'd offered 2x2=4 explanations for the extra distances, the one of which I tentatively favour despite not having got the details to work out yet being number (2,2). So e.g. if my scribbly map is right, which it probably isn't, you could go DBAE, all along the DFT, and the two distances shown rightward from A are A->B and B->D respectively. One thing that's lacking in all my explanations, though, is a principled explanation of how they decided when to list two distances -- it doesn't appear to be "whenever possible". (But maybe with the right map it is.) $\endgroup$
    – Gareth McCaughan ♦
    Commented Jul 3, 2016 at 2:58
  • $\begingroup$ On sign A, .7 is a distance from A $\endgroup$
    – humn
    Commented Jul 3, 2016 at 2:59
3
$\begingroup$

Perhaps:

The terms 'Dutch flat trail', etc. do not describe destinations, but the type of trail, i.e. 'Dutch flat' might be flat, 'Cañada' might involve scrambling, 'Lollipop' be suitable for kids (or similar). So the signs are simply saying what type of trail goes off in each direction. IE the names are adjectival.

and

The reason for 2 x 'Dutch flat trail' in the same direction is because there you can travel 0.1 mile that way, and either turn off onto a different type of trail, or do a further 0.6 miles on the same type of trail

Improve this answer
$\endgroup$
3
  • $\begingroup$ Great ideas for a different puzzle, although one of the terms you mention does, in a way, describe a part of the underlying map $\endgroup$
    – humn
    Commented Jul 4, 2016 at 22:07
  • $\begingroup$ @humn is it simply that the dutch flat trail is quite flat (suitable say for wheel chairs, but after 0.1 miles goes under a bridge, and 0.6 miles later goes over the same bridge, with there being some staircase or similar up the side of the bridge - i.e. a loop that can be cut off? $\endgroup$
    – abligh
    Commented Jul 4, 2016 at 22:13
  • $\begingroup$ To have different routes for different abilities would be quite enlightened. Alternate routes do play a role in this puzzle but not exactly along the lines you describe. $\endgroup$
    – humn
    Commented Jul 4, 2016 at 22:18
2
$\begingroup$

Incomplete guess:

I'm working off of Gareth's 2nd option (trail refers to the trail you are walking along, distance is distance to next point of interest), which I also think is the correct interpretation.

For signs A, B and C, which indicate two trails in the same direction but for different distances, the shorter length might be literally a point of interest (something like a landmark or interesting sightseeing spot). However, they are not junctions. The longer distance indicated is the distance to the next junction along the stated trail.

So the missing information is:

The reason why x units from the sign is marked out (is it a landmark, another junction, or interesting in some other way?)

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.