135
$\begingroup$

The Sheikh dies, leaving behind three sons, 17 camels and the following order:

  • His oldest son shall inherit one in two camels.
  • His middle son shall inherit one in three camels.
  • His youngest son shall inherit one in nine camels.

Now the three don't know what to do. So they ask an old friend of the family, which knows a solution such that everyone is happy.

What did he propose?

Improve this question
$\endgroup$
8
  • 30
    $\begingroup$ This was the example with which we were taught about catalyst (in Chemistry) in school :) $\endgroup$
    – PermanentGuest
    Commented Oct 8, 2014 at 16:12
  • 2
    $\begingroup$ why sheikh..... $\endgroup$
    – Naeem Shaikh
    Commented Aug 28, 2017 at 14:01
  • 5
    $\begingroup$ @NaeemShaikh Who else would own so many Camels? $\endgroup$
    – Jasen
    Commented Oct 13, 2017 at 5:20
  • 7
    $\begingroup$ He offers to chop the camels in half, and then gives all the camels to the one who implores him not to kill the camels and give them all to his brothers? $\endgroup$
    – Jack M
    Commented Oct 16, 2017 at 21:48
  • 4
    $\begingroup$ @OldBunny2800 en.wikipedia.org/wiki/Judgment_of_Solomon $\endgroup$
    – Alexander
    Commented Oct 17, 2017 at 6:33

8 Answers 8

Reset to default
134
$\begingroup$

He brought an extra camel to assist in the division. Current total = $18$.
Oldest son gets = $18/2 = 9$
Middle son gets = $18/3 = 6$
Youngest son gets = $18/9 = 2$
Total camel involved = $9+6+2 = 17$.

The old friend then takes back the remaining extra camel and solves the problem.

Improve this answer
$\endgroup$
8
  • 9
    $\begingroup$ Nope, this is actually the genuine solution in all formulations of the problem. $\endgroup$
    – user88
    Commented Oct 7, 2014 at 16:17
  • 36
    $\begingroup$ @SiliconMind a sarcastic answer might be, he killed all three sons and took the camels for himself, making them all happy ("Call no man happy before his death" -- Solon of Athens). $\endgroup$
    – David Conrad
    Commented Oct 7, 2014 at 17:31
  • 3
    $\begingroup$ are you saying 17=18 ???? according to your answer, 9/17 = 1/12 . $\endgroup$
    – Alireza Fallah
    Commented Oct 11, 2014 at 10:41
  • 8
    $\begingroup$ @bgmCoder Plot twist: all the camels are actually fish. $\endgroup$
    – Rand al'Thor
    Commented Oct 2, 2016 at 1:25
  • 6
    $\begingroup$ @randal'thor haha - humpback whales $\endgroup$
    – bgmCoder
    Commented Oct 2, 2016 at 14:33
105
$\begingroup$

Note that the mathematical inconvenience is caused by the fractions in the will not summing to one:
$$\frac{1}{2} + \frac{1}{3} + \frac{1}{9} = \frac{17}{18}$$

Assuming that the father intended to divide the entire flock, we can

normalize the fractions using division by 17/18, to get the expected solution.

  • The eldest son will get:

    $$ \frac{1}{2} \div \frac{17}{18} \times 17\ \textrm{Camels} = 9\ \textrm{Camels} $$

  • The middle son will get:

    $$ \frac{1}{3} \div \frac{17}{18} \times 17\ \textrm{Camels} = 6\ \textrm{Camels} $$

  • The youngest son will get:

    $$ \frac{1}{9} \div \frac{17}{18} \times 17\ \textrm{Camels} = 2\ \textrm{Camels} $$

Improve this answer
$\endgroup$
3
  • 2
    $\begingroup$ In the traditional solution, this is done by the wise man coming along and giving them his camel. $\endgroup$
    – user88
    Commented Oct 7, 2014 at 16:18
  • 9
    $\begingroup$ @JoeZ. But this seems a lot simpler to understand. The wise man trick fails mathematically in other situations even though it may look just as logical. $\endgroup$
    – ghosts_in_the_code
    Commented Jan 2, 2016 at 11:24
  • 2
    $\begingroup$ @ghosts_in_the_code It does work mathematically in every situation, just you need to figure out how many to add. It's basically just reverse-engineering this. $\endgroup$
    – somebody
    Commented Apr 23, 2018 at 3:56
65
$\begingroup$

C = Camel.

First son gets

C C C C C C C C C C C C C C C C C = 9 camels.

Second gets

C C C C C C C C C C C C C C C C C = 6 camels.

Third gets

C C C C C C C C C C C C C C C C C = 2 camels.

Total number of camels is 17. No need for an extra camel.

Improve this answer
$\endgroup$
8
  • 2
    $\begingroup$ However, in the first case, for example, the first son is getting 9/17 of all the camels, not 1/2 of them. $\endgroup$
    – user88
    Commented Oct 7, 2014 at 16:18
  • 26
    $\begingroup$ The first gets 9/17, > 1/2, the second gets 6/17 > 1/3, and third gets 2/17 > 1/9. They all get more than their fair shares of 17. $\endgroup$
    – djv
    Commented Oct 7, 2014 at 19:19
  • 22
    $\begingroup$ I interpreted "one in two", "one in three", and "one in nine" to mean exactly this. $\endgroup$
    – Justin
    Commented Oct 8, 2014 at 0:02
  • 5
    $\begingroup$ This solution looks right, but doesn't work if you would actually do it. <br/> You can see the first C is marked for the first, second and third son. So who gets it after all?<br> If you would do this, it's more like:<br> C C C C C C C C C C C C C C C C C<br> <br> 1 1 1 1 1 1 1 1 <br> 2 2? 2 2? 2 <br> 3? <br> <br> So this is from where the confusion comes: according to the algorithm, some camels have multiple owners, while others have none. $\endgroup$
    – Flax
    Commented Oct 12, 2014 at 11:05
  • 2
    $\begingroup$ @Flax one should assume that some communication between the brothers exists that solves that particular problem. In any case, my aim was not to actually "solve" the problem, it was to show that one can solve it in another way than the usual. $\endgroup$
    – Jakob
    Commented Oct 13, 2014 at 6:33
15
$\begingroup$

This is how I see it. The father being a knowledgeable man, knows that he has three pregnant camels.

So at the time of his death he says that the oldest would receive one in two, or one camel, a pregnant camel, which implies a third camel carried by the mother camel. Thus the oldest receives three camels.

The same goes for the middle who receives one in three camels. He will get two camels who are not pregnant and one that is. Thus, with time, he will receive four.

Finally the youngest will receive eight not pregnant camels, and one that is. Thus he will receive ten camels.

3+4+10=17.

The point would be that all three sons will have at least one fertile camel (the other camels may be infertile). The old friend is brought in to distinguish each pregnant camel and to allot one to each son.

No extra camels.

Improve this answer
$\endgroup$
1
  • 4
    $\begingroup$ But this requires that initially they have only 14 (visible) camels. I don't think when a statement says "XYZ dies and leaves behind 17 camels" it actually means 14 camels with 3 of them pregnant. $\endgroup$
    – justhalf
    Commented Oct 9, 2014 at 6:38
6
$\begingroup$

The old friend knows Sheikh and their son from long time ago...

He knew that the sons were not happy to work with each other and always complain and threat that they want to be separate. This makes the Sheikh very sad !

So Sheikh decided to create an impossible puzzle, not only to convince the sons to be with each other, but also show them that there are many cases that being together is the best solution.

So the old friend knows the solution:

None of you can inherit any thing, you must be together and share the camels.

Improve this answer
$\endgroup$
2
  • 6
    $\begingroup$ the tradional solution to such a conundrum is fratricide. $\endgroup$
    – Jasen
    Commented Jun 19, 2016 at 0:07
  • $\begingroup$ @Jasen, I know that this is an extremely late response, but that would not satisfy the question's phrase "everyone is happy." $\endgroup$
    – Galactic
    Commented Nov 25 at 6:24
5
$\begingroup$

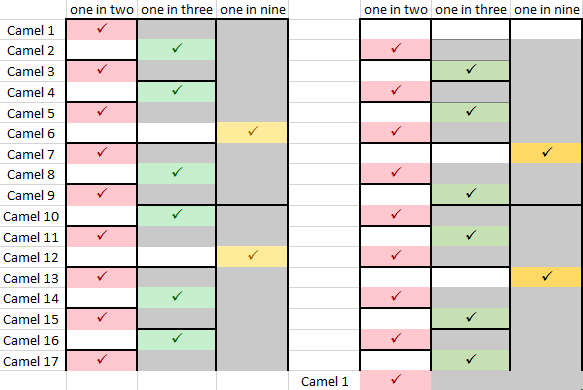
There are other correct answers up there, but this puzzle is improved if instead of $18-1=17$ camels is available, there are $2*18-1=35$ camels available.

Then when the old friend brings a camel to make the division work, eldest gets $36/2=18$, middle gets $36/3=12$, youngest gets $36/9=4$ camels, which sums to 34, leaving TWO surplus camels, so the old friend gets his camel back, and claims the other as his reward for solving the brothers' problem.

[edit] I delivered a version of the traditional puzzle to an extracurricular math club for 6th-7th graders this week, and they loved it. I staged it "One day in Arabia, a mathematician was traveling on his camel through the desert, when he came across three men and a pack of camels. They were crying, and sharpening knives..."

After the class was done, I was thinking, wouldn't it be cooler if instead of just getting his camel back, he actually got a bonus camel? And (near)doubling the herd was able to double the slop from those fractions not summing to 1. I'm going to ask them next week, "What if there were 35 camels?" and let them work it out.

Improve this answer
$\endgroup$
3
  • 4
    $\begingroup$ You can't just change the rules of the question like that. $\endgroup$
    – boboquack
    Commented Oct 11, 2017 at 2:08
  • 2
    $\begingroup$ It's not a rule change, it's an improvement. Will definitely try to remember. $\endgroup$
    – Alexander
    Commented Oct 11, 2017 at 7:38
  • 2
    $\begingroup$ Thanks. I delivered a version of the traditional puzzle to an extracurricular math club for 6th-7th graders this morning, and they loved it. I staged it "One day in arabia, a mathematician was traveling on his camel through the desert, when he came across three men and a pack of camels. They were crying, and sharpening knives..." $\endgroup$
    – RubeRad
    Commented Oct 11, 2017 at 14:31
4
$\begingroup$

I number camels like below and group them as two / three / nine to select one in each group:

       1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17
      +---+---+---+---+----+-----+-----+-----+---.
two   |   |   |   |   |    |     |     |     |
      +---+-+-+---+---+-+--+-----+-----+--+--+---.
three |     |     |     |        |        |  
      +-----+-----+-----+--------+--------+------.
nine  |                 |                 
      +-----------------+------------------------.

That last groups not completed, I can select that wanted one camel in two ways:

  1. First camel

           1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17
      +---+---+---+---+----+-----+-----+-----+---.
two   |*  |*  |*  |*  |*   | *   | *   | *   | *      => 9 Camels
      +---+-+-+---+---+-+--+-----+-----+--+--+---.
three |- * -|* -  |- * -| *  -   | -  *  -| *  -      => 6 Camels
      +-----+-----+-----+--------+--------+------.
nine  |- - - - - * - - -| -  -  *  -  -  -  -  -      => 2 Camels
      +-----------------+------------------------.

  2. Last camel

           1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 ..
      +---+---+---+---+----+-----+-----+-----+---..
two   |  *|  *|  *|  *|   *|    *|    *|    *|    *   => 8 Camels + 1  out-bounded Camel 
      +---+-+-+---+---+-+--+-----+-----+--+--+---..
three |  - *|- * -|  - *| -  *  -|    -  *| -  *  -   => 6 Camels
      +-----+-----+-----+--------+--------+------..
nine  |  - - - - - * - -| -  -  -  *  -  -  -  -  -   => 2 Camels
      +-----------------+------------------------..

    And Camel 1 is not selected so replace it with that out-bounded camel.

And at last one in two selects 9 camels, one in three selects 6 camels and one in nine selects 2 camels.

An extra image to make more sense

Improve this answer
$\endgroup$
4
$\begingroup$

The point is that each brother is "happy" because each one ends up with at least as much as he was promised in the will. We do not in fact give the eldest brother $\frac{17}2$ camels, we give him $\lceil\frac{17}2\rceil$. But the question only requires that he be "happy", not that he get exactly the right amount. It just so happens that $\lceil\frac{17}2\rceil+\lceil\frac{17}3\rceil+\lceil\frac{17}9\rceil=17$. In other words, the father left them less than 100% of his camels in the will, which is why there was wriggle room to give them all what they wanted while giving the family friend a bit more.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.