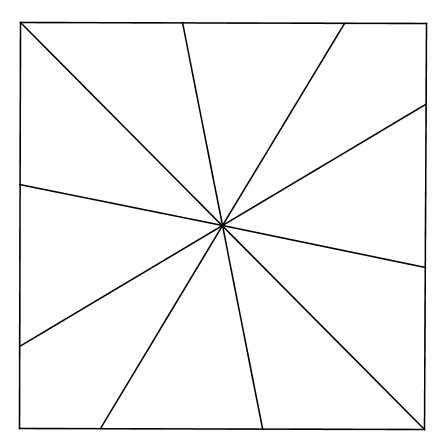
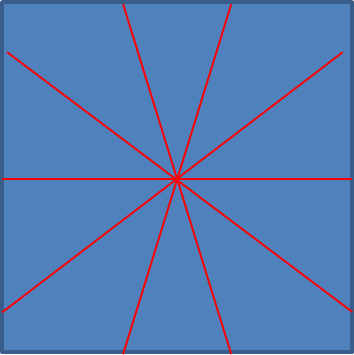
You are serving a cake to $10$ children. The the cake is shaped like a box, whose top face is square. The top and sides are covered with a thin layer of frosting.
$\qquad\qquad\qquad\qquad$
Every child demands a fair share, so you must cut the cake into $10$ pieces where every piece has the same volume of cake and surface area of frosting. How do you do this?