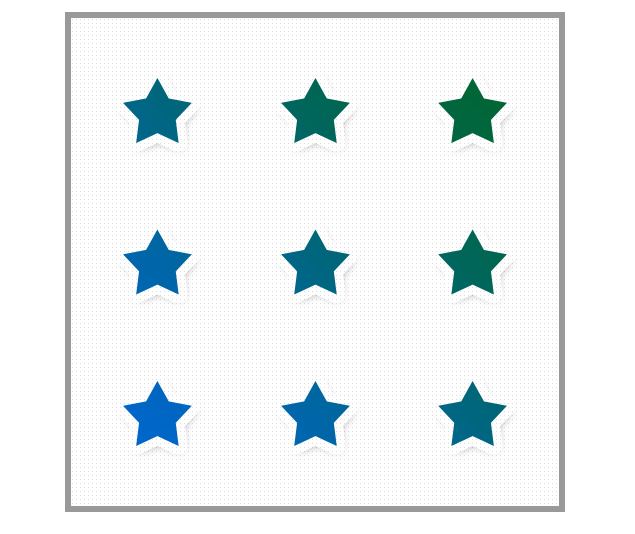
Using 4 straight lines connect these 9 stars together without lifting your pen from the surface. The end of each line must be start of the next line.

Using 4 straight lines connect these 9 stars together without lifting your pen from the surface. The end of each line must be start of the next line.
I've never seen this one before, but here's what jumps out at me:
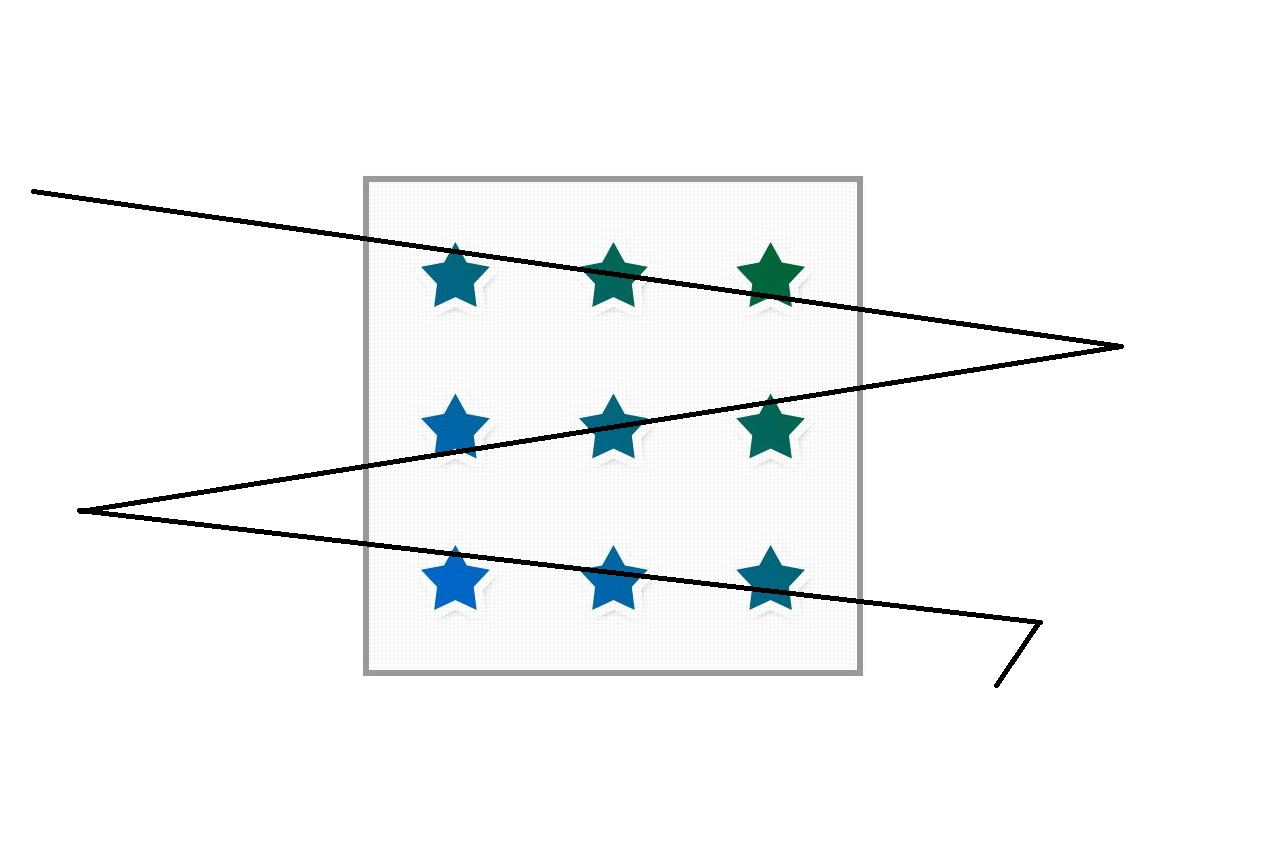
Using the information from http://en.wikipedia.org/wiki/Parallel_(geometry)#Extension_to_non-Euclidean_geometry:
- parallel, if they do not intersect in the plane, but have a common limit point at infinity, or [...]
So, if we put this in a non-Euclidean plane, it can be done with one line:
But, of course, this would take forever to draw (But it does pass through the centres of all stars)
I can also confirm it is impossible to do on a Euclidean plane without going outside the box with this Python script.
There are 84 different ways to do it with 5 lines inside the box, but that should be around 11 unique ones because most are reflected or rotated.