Can you assemble the icosahedron?

Question

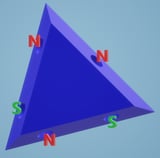
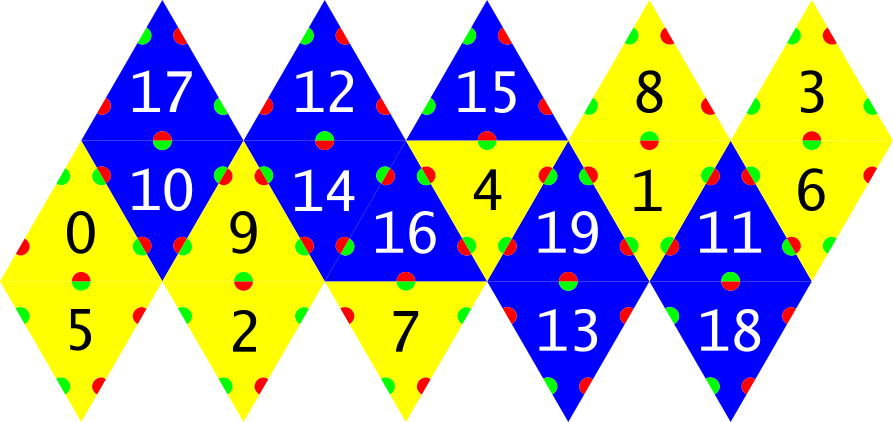
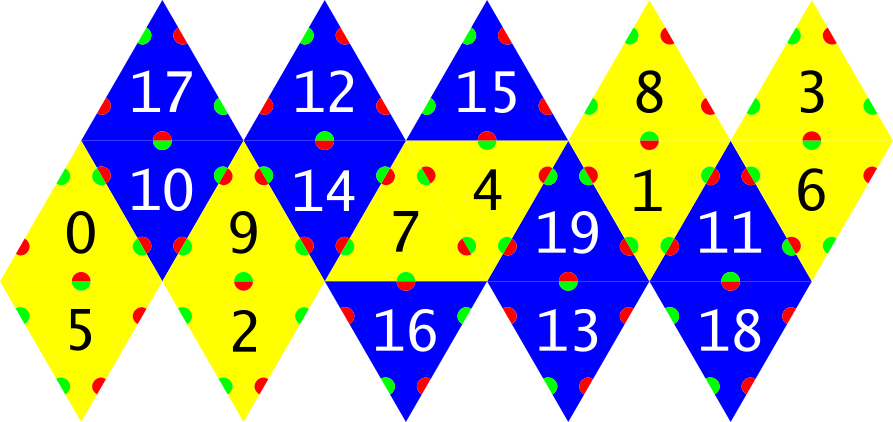
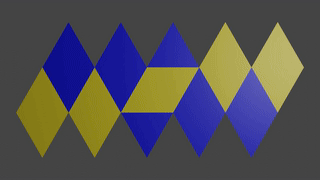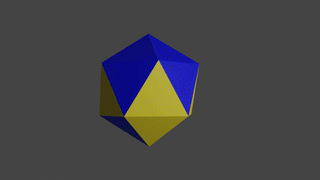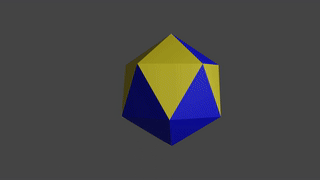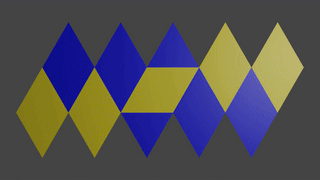
An icosahedron has 20 faces which are equilateral triangles.

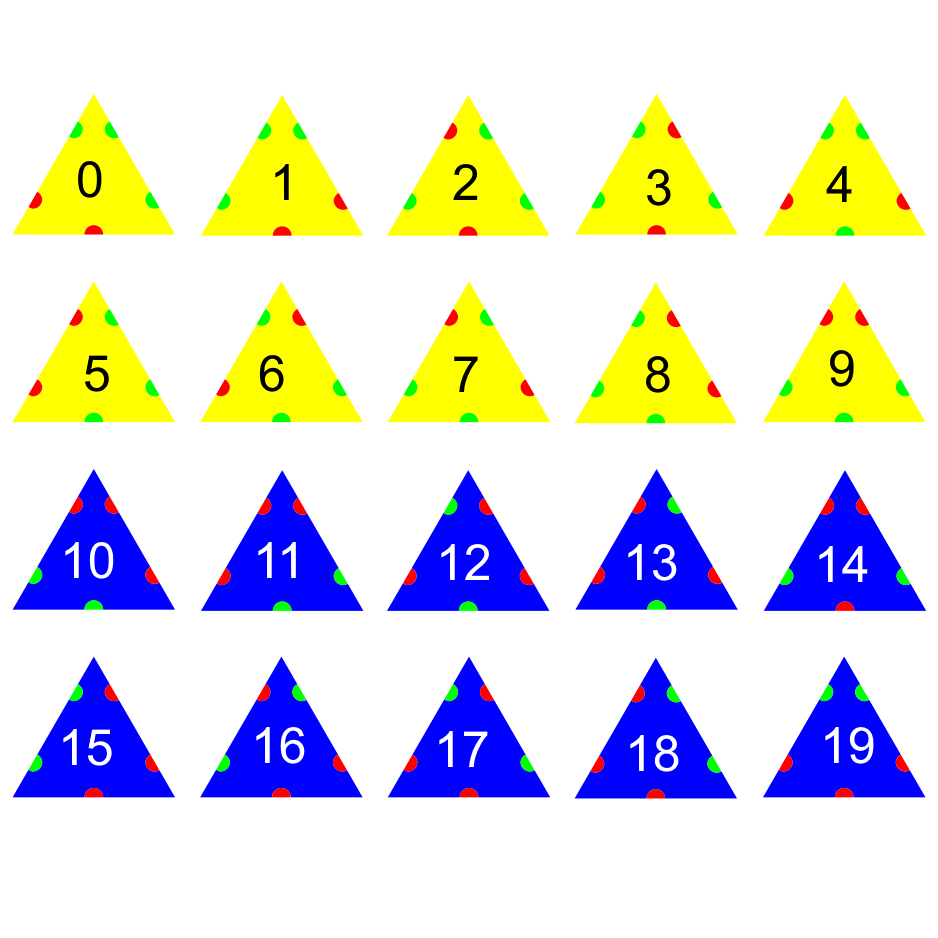
Tom wants to create a very hard mechanical puzzle. With his brand new 3D printer in mind he designs 20 puzzle pieces with identical shapes which in principle nicely fit together to build the icosahedron:

The face that is visible after assembling the icosahedron is on the back.

To hold the parts together he uses magnets which he glues into the 5 holes that he had prepared quite devilishly.

There are exactly 10 ways to orient the magnets such that two magnets are oriented with north poles facing outwards. These 10 parts he wants to print in yellow. Here is one example:

And of course there are also exactly 10 ways to orient the magnets with two south poles facing outwards. These 10 parts he wants to print in blue. Example:

Hey, we got a complete set of exactly 20 different pieces! There are $19!*3^{19}> 10^{26}$ ways to assemble the icosahedron if we ignore the magnets.

1. Is at least one way for the magnets to match everywhere (north to south)?
2. Are there especially nice looking solutions with 5-fold symmetry? Obviously, only the colors of the pieces are interesting for this part.

Answer 1

Partial Solution:
The answer to part 1 is:

Yes. I wrote a program to brute force solutions.
While it is true that there are 19! * 3^19 possible arrangements of triangles on an icosahedron, the magnets severely limit tilings. My brute force search is limited to solutions that match the orientation of magnets in the solution below, which restrains the rotation of each triangle and so has a mere 19! solutions to comb through.

Numbering the possible triangles like so:
One possible solution is:

For part 2:

I was able to find a more symmetrical solution by hand.
I started by filling in the top and bottom 5 triangles on the net. I tried to use up as many of the pieces with two of the same pole on the sides. I also made sure to use the inverse of each piece on the opposite end:

Once I had these two ends, I worked on the center. I started by connecting piece 10 to piece 0, and piece 11 to piece 1, since those were the only possible connections left for those sets of edges. This part was the most trial-and-error, getting a row that connected and had the correct pattern of poles on the top and bottom. While I wanted to keep things symmetrical here, that didn't seem possible. I eventually found this pattern that works, though the top end cap would need to be shifted to line up:

From here, I was able to get a complete net that looked like this:

Just for fun, I started shifting the net around, and it ended up in this configuration. I noticed that the diamond formed by pieces 7 and 16 could be rotated 180 degrees, and this is the final net I've settled upon:

While this construction does not have 5-fold symmetry, it does have a single axis of rotational symmetry, shown in the animation below:

Answer 2

Part 2:

There are only two ways to arrange the 10 single-magnet edges (shown with thicker lines), and only one of these arrangements leads to a solution.
Also, this is the only color pattern that works.