Given a special name

Question

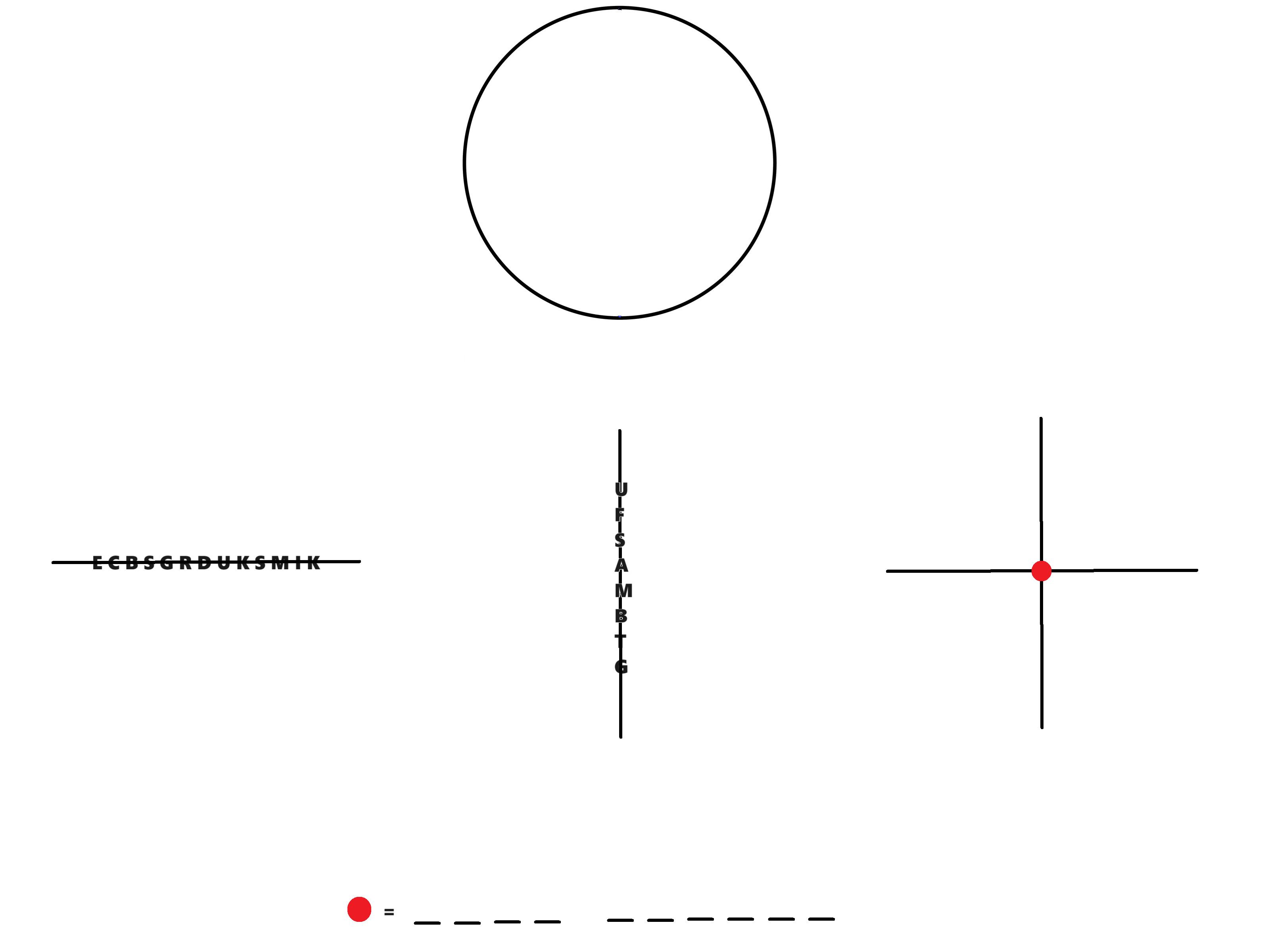
What's the 10-letter answer to this puzzle?

---

Answer 1

The 10-letter answer is:

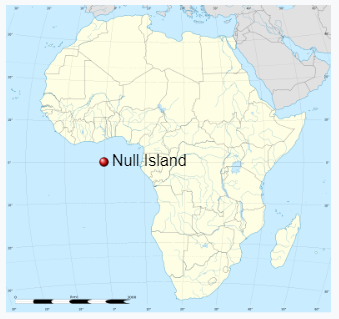
NULL ISLAND. This is the name given to the location on Earth at zero degrees latitude and zero degrees longitude, where the prime meridian intersects with the equator, in the Gulf of Guinea (there is no physical island there though!).


_{Source: Wikipedia}

To find this, interpret the images as follows:

1. The circle is a basic representation of the Earth.

2. The horizontal line passes through 13 letters: ECBSGRDUKSMIK. These correspond to the starting letters of the 13 countries through which Earth's equator passes, in order from west to east (on a typical map of the world centred on the prime meridian):

Ecuador, Colombia, Brazil, Sao Tome & Principe, Gabon, Republic of the Congo, Democratic Republic of the Congo, Uganda, Kenya, Somalia, Maldives, Indonesia and Kiribati.

3. The vertical line passes through 8 letters: UFSAMBTG. These correspond to the starting letters of the 8 countries through which the prime meridian passes, in order from north to south:

United Kingdom, France, Spain, Algeria, Mali, Burkina Faso, Togo, Ghana.

4. The cross shape with a red dot at the centre suggests identifying the point where these two lines meet on the globe, with longitude and latitude (0,0). This is the theoretical point known as NULL ISLAND! (This spot has been 'given a special name' largely to help mapping software developers to find and correct database entries that have erroneously been assigned the coordinates (0,0)...)