Ed Pegg found in December 2019 this amazing configuration consisting of 22 points in 28 lines of 4.
On those points place 22 different positive integers such that the sum of any of the four points in a line is always the same.
¿How small can the largest of those numbers be?
I think the answer is
It is impossible, no matter the upper bound.
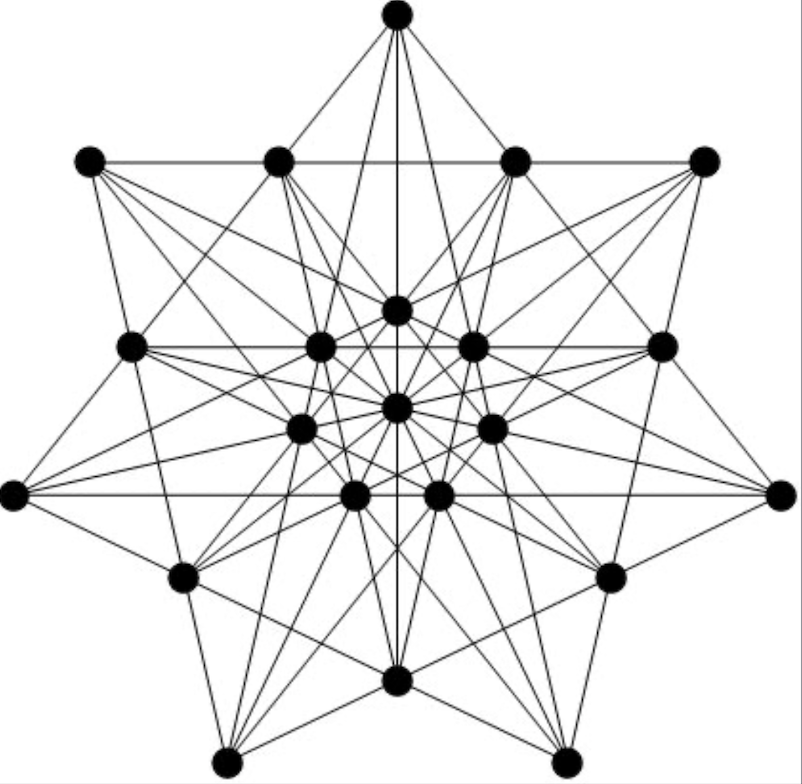
The solution is given by the image below:
Here is the GeoGebra project that produced this image. Consider the sums of five blue lines and five red lines. Other than the two points B1 on two blue lines and C1 on red, all points are counted in both sums the equal number of times. This proves that B1 = C1, which contradicts with the "distinct numbers" condition.
This was found by
trascribing the conditions into this Z3 script and studying the "unsat core", i.e. a collection of conditions that leads to contradiction. Note that the unsat core does not involve any condition related to
max.
@quarague asked about the case where some numbers can be equal but not all of them. In this case, the answer is
still impossible.
In the diagram, there are three regular heptagons that share the center. Let's call the vertices of the largest one $A_i$, the second largest $B_i$, and the smallest $C_i$, with $1 \le i \le 7$, and the center itself $D$. The vertices are numbered so that $A_i, B_i, C_i, D$ are collinear. Also, let's write the numbers written on each point in lowercase, i.e. $a_i, b_i, c_i, d$. The "solution" above contains an image with this way of labeling.
In the picture above, I proved that $b_i = c_i$. Looking at the lines that pass through two $B$s and two $C$s, we get seven sums consisting of four $b_i$s. Two of them are $b_1 + b_2 + b_4 + b_5$ and $b_1 + b_2 + b_5 + b_6$ (from the two lines starting from $B_5$), which proves that $b_4 = b_6$. By rotational symmetry, we conclude that all $b_i$s and all $c_i$s are equal.
Then we turn to the lines that pass through two $A$s and two $B$s. Since all $b_i$s are equal, we get equations like $a_1 + a_3 = a_3 + a_5$, which again proves that all $a_i$s are equal. Also, since $2a + 2b = 2b + 2c$, $a = c$. Finally any one line passing through $D$ proves that $d$ is also equal to the rest of the numbers.
