Well, let's list the available piece identifying factors in the Chess960 rules:
- There are two of each piece except the King and the Queen
- The two bishops must be on opposite colours
- The King must be between the rooks
and.. that's just about it.
We can then list stuff that cannot occur in a position where each piece type is identifiable from the position alone:
- Knights on opposite coloured squares (cannot tell knights from bishops)
- King or Queen between the knights (cannot tell rooks from knights)
- Queen between the rooks (cannot tell the King from the Queen)
- King or Queen between the bishops when the rooks are on opposing colours (cannot tell bishops from rooks)
These let us quickly solve the Easier Bonus; your position must have looked like this:
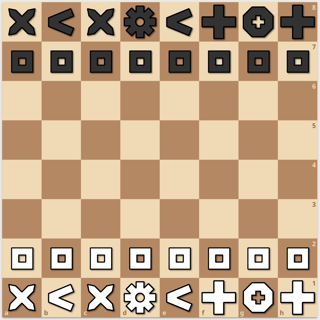
In this position, all the pieces are unambiguously identifiable: the bishops are easy to spot by the colour of their squares, and after that the King and the rooks are obvious.
To confirm that this is indeed the only position with the Queens on the d-file where all the pieces are identifiable, we can notice that:
- The King cannot be to the left of the Queen: with rooks at a1 and c1, the knights would unavoidably end up on opposite colours, making them indistinguishable from the bishops
- Neither the knights nor the rooks can straddle the queen, so when the King takes the rooks to the right side of the Queen, "a1 and c1" is the only way to place the knights on same coloured squares
- Thus, b1 can only be a bishop
- With the bishops straddling the Queen, the rooks must now be on same coloured squares, with the King in between
- Which leaves only one way (e1) to place the dark square bishop on the right side of the queen.
Then, for the difficult part, viz. counting.
Without any extra rules, legal Chess960 starting positions are easy to count, as long as you place the pieces in a correct order, for example:
Light square bishop, Dark square bishop, Queen, both knights, the rest.
The number of options at each step can then just be multiplied together to get 4 x 4 x 6 x 10 x 1 = 960.
With our convoluted restrictions, especially where the rook position affects the bishop position, we're not going to get anything so nice, I'm afraid.
Instead, we'll do a rough guesstimate, and leave enumerating all the positions (which I think is the only way to get the exact count) to someone with more time and/or a computer.
For simplicity's sake, let's assume that on average, for a pair of pieces, there's an equal amount of space to the left, in between, and to the right of them. This lets us approximate how likely it is that each of the four "forbidden combinations" listed above is going to leave our position identifiable: (the numbers below are iffy at best, even without taking into account the possibility that we may have missed another ambiguity creating feature altogether)
- Knights on opposite colours: 3/7
- King between knights: 2/3
- Queen between knights: 2/3
- Queen between rooks: 2/3
- Rooks on opposing colours and Queen between bishops: 17/21
- Rooks on opposing colours and King between bishops: 17/21
Multiplying these together we get the portion of legal starting positions that are also identifiable, and since we know there are 960 legal starting positions altogether, we end up in the vicinity of
$\frac{3\times 2\times 2 \times 2 \times 17 \times 17}{7\times 3\times 3 \times 3 \times 21 \times 21}\times 960 = \frac{6936}{83349}\times 960 \approx 80 $ positions with all the pieces identifiable.
(I may be completely off with my calculations though.)
Epilogue: turns out the someone with the computer was me all along! All the Chess960 positions where the pieces are identifiable regardless of what they look like are
BBNRNKRQ
BBQNRNKR
BBQRKNRN
BBRKNRNQ
BNRNKBRQ
BQNBNRKR
BQNRNBKR
BQRBKNRN
BRKBNRNQ
BRKRNBNQ
BRNBNKRQ
NBBRNKRQ
NBNQBRKR
NBNRBKRQ
NBNRKRBQ
NRBBNKRQ
NRNBBKRQ
NRNBKRBQ
NRNKBBRQ
NRNKBRQB
NRNKRBBQ
NRNKRQBB
QBBNRNKR
QBBRKNRN
QBNRNKBR
QBRKBNRN
QBRKRNBN
QBRNBNKR
QNBBRNKR
QNBNRBKR
QNBNRKRB
QNRBBNKR
QNRNBBKR
QNRNBKRB
QNRNKBBR
QNRNKRBB
QRBBKNRN
QRBKNBNR
QRBKNRNB
QRKBBNRN
QRKBRNBN
QRKNBBRN
QRKNBNRB
QRKNRBBN
QRKNRNBB
QRNBNKBR
RBBKNRNQ
RBKNBNRQ
RBKNRNBQ
RKBBNRNQ
RKBNRNQB
RKBRNBNQ
RKNBBRNQ
RKNBNRBQ
RKNRBBNQ
RKNRNBBQ
RKNRNQBB
RKRBQNBN
RKRNBNQB
RNBNKBRQ
For a total of
60 possible positions,
and unsurprisingly indeed, only one position (C960 position number 40, highlighted above) has the Q at the d-file.
So my earlier guesstimate wasn't very far off, but not especially close either. Going to blame it on my "probability of being in between two given pieces" estimation being abhorrently bad.

