3
$\begingroup$

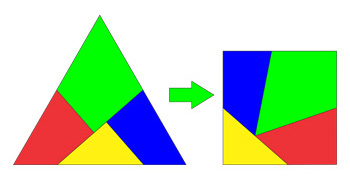
You may be familiar with Dudeney's famous dissection of the equilateral triangle into a square. (A nice physical version is demonstrated here.) His dissection uses four pieces. I believe this to be the minimum (though I have never seen a proof).

Dudeney's dissection of a triangle into a square

Now, I shall give you superpowers: you may dilate (aka resize, aka grow and shrink) any piece or pieces.

With this new ability, how few pieces are required to dissect the equilateral triangle into the square? (Said another way, you must partition the equilateral triangle and square each into pieces such that each piece of one is similar (but not necessarily congruent) to a corresponding piece in the other.)

This is a golf-style question: fewest pieces wins.

Improve this question
$\endgroup$
4
  • $\begingroup$ You can model this in the real world using shadows, I suppose. $\endgroup$
    – Akiva Weinberger
    Commented Dec 15, 2022 at 1:22
  • $\begingroup$ @bobble It's doable in 3, but I have no idea if it is optimal (I suspect yes). $\endgroup$
    – Akiva Weinberger
    Commented Dec 15, 2022 at 1:25
  • $\begingroup$ @AkivaWeinberger - would shadows be a valid real-world equivalent? Shadows can be skewed (i.e. not resized proportionally in both dimensions), is that still a "resize" per the terms of your puzzle. $\endgroup$
    – Phylyp
    Commented Dec 15, 2022 at 1:50
  • $\begingroup$ @Phylyp No, it wouldn't, you're right. This question does not allow skews. Shadows are too high-powered. (EDIT: In fact, all triangles are 'shadow-equivalent' to each other, which would make this way too easy.) $\endgroup$
    – Akiva Weinberger
    Commented Dec 15, 2022 at 1:52

1 Answer 1

Reset to default
5
$\begingroup$

It is actually rather easy to achieve

3

Just observe that the base of the triangle is more than its height. Cut vertically near both ends of the base such that the centre bit has width equal to the height (sqrt(3)/2 of the base). The centre piece is a square with two 30-60-90 triangles removed the same shape as the cut-off bits. So simply rescale them as needed.

Improve this answer
$\endgroup$
5
  • $\begingroup$ This is significantly simpler than my answer, lol! I'll wait a bit in case someone magically has a way to do it in 2, but after that I'll probably checkmark your answer. $\endgroup$
    – Akiva Weinberger
    Commented Dec 15, 2022 at 2:11
  • $\begingroup$ I wonder if a square and a 1:2 rectangle would have been a harder challenge… $\endgroup$
    – Akiva Weinberger
    Commented Dec 15, 2022 at 2:13
  • $\begingroup$ @AkivaWeinberger No, I don't think so. $\endgroup$
    – loopy walt
    Commented Dec 15, 2022 at 2:28
  • 1
    $\begingroup$ You're right: there's a normal dissection of a square into a 1:2 rectangle with 3 cuts. $\endgroup$
    – Akiva Weinberger
    Commented Dec 15, 2022 at 3:16
  • $\begingroup$ ("pieces", not "cuts") $\endgroup$
    – Akiva Weinberger
    Commented Feb 26 at 18:37

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.