16
$\begingroup$

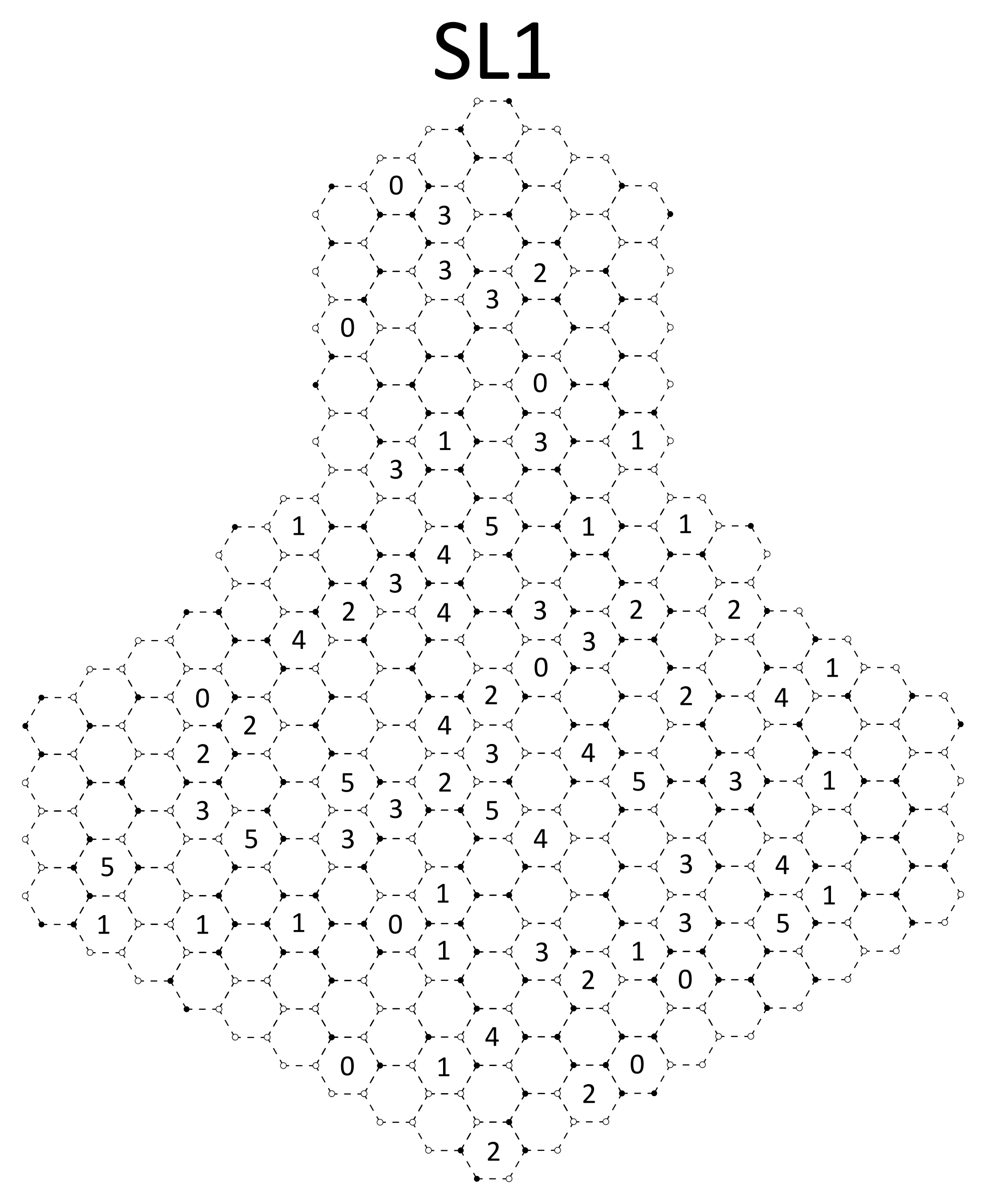
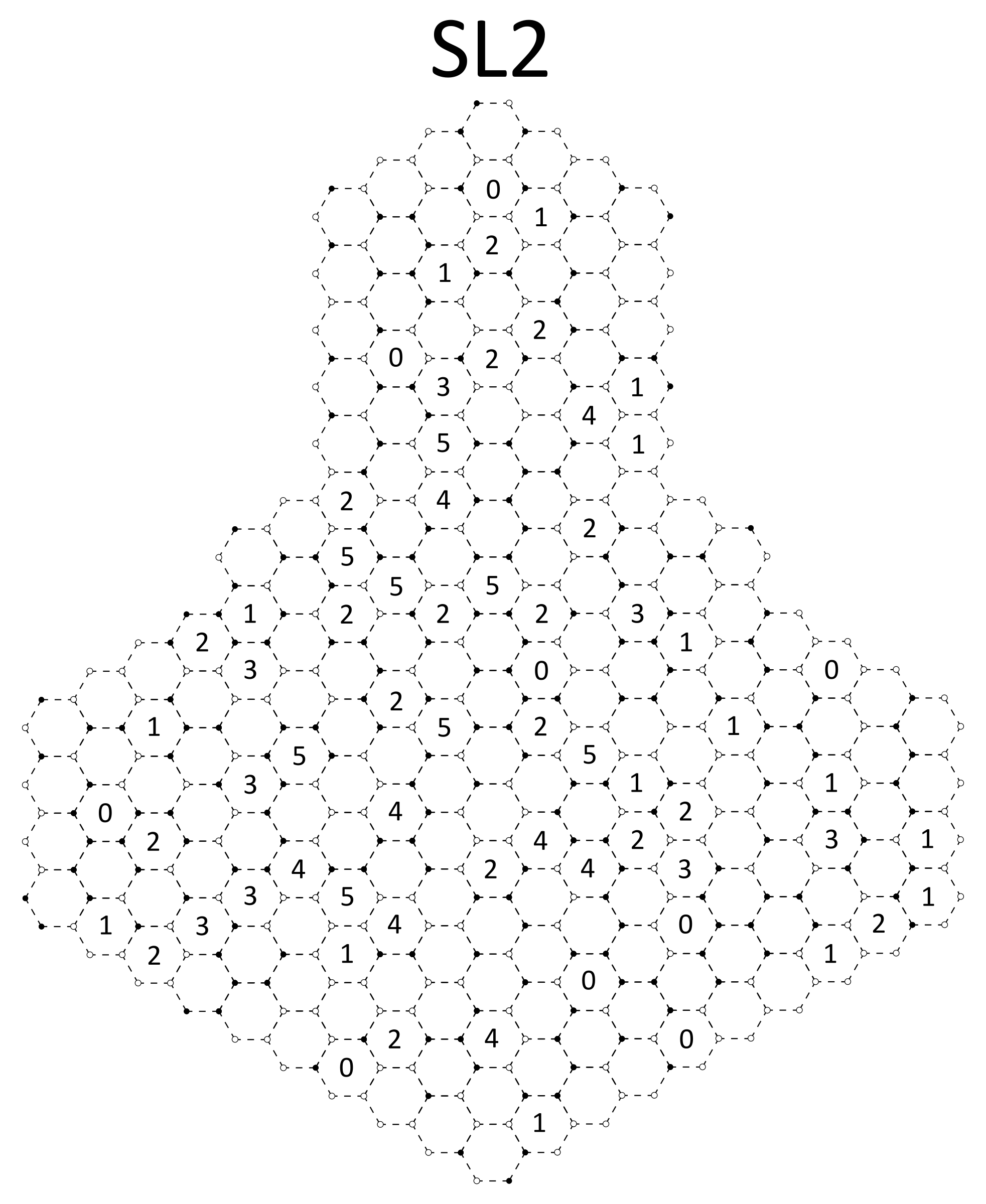
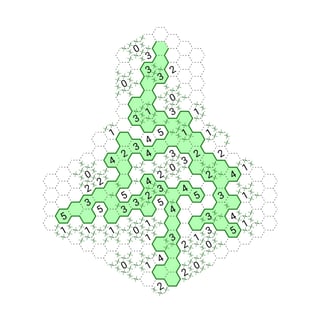
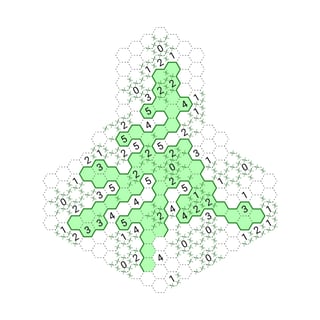
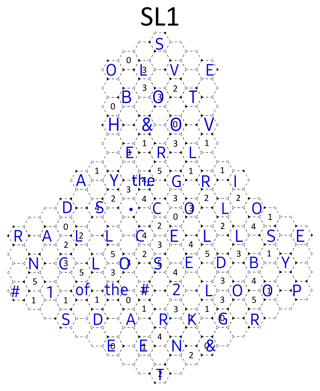
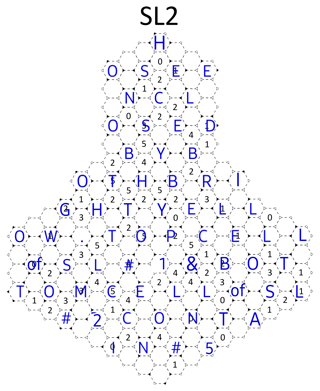
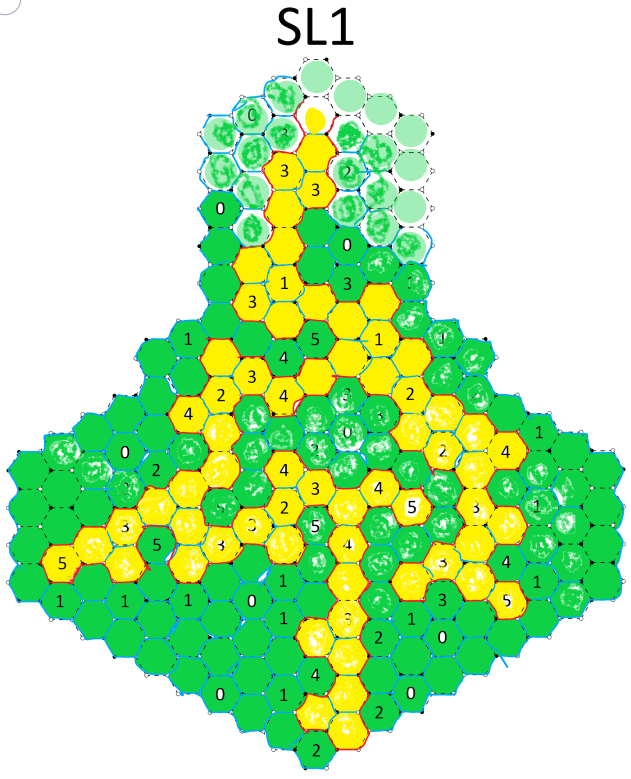
This puzzle is part of the Puzzling Stack Exchange Advent Calendar 2022. The accepted answer to this question will be awarded a bounty worth 50 reputation.

< Previous DoorNext Door >

'Twas the night before Christmas 1802, and Thomas Young was conducting his now famous double-slit experiment. It was the first demonstration of the wave behaviour of visible light. Moreover, it displays the fundamentally probabilistic nature of quantum mechanical phenomena.

All in all, the double-slit experiment has become a classic for its clarity in expressing the central puzzles of quantum mechanics.

Fast forward 220 years later, we still love puzzles!

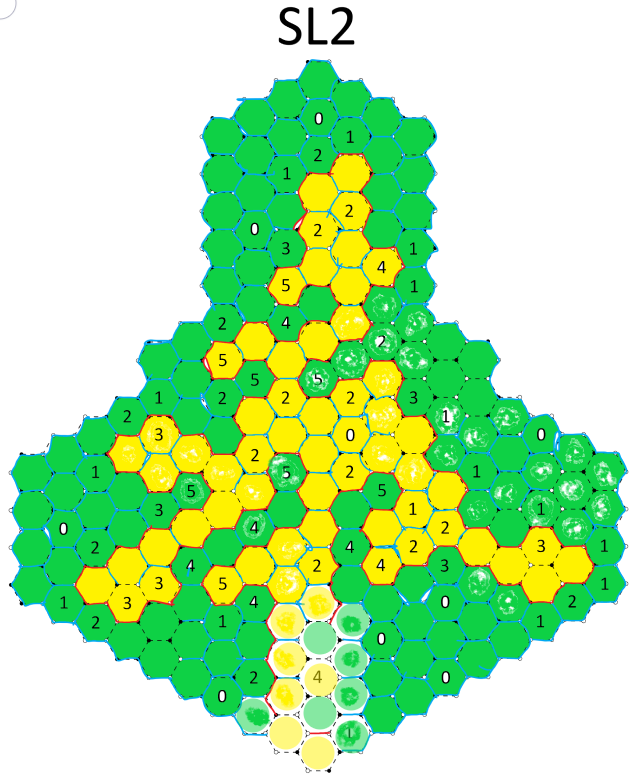
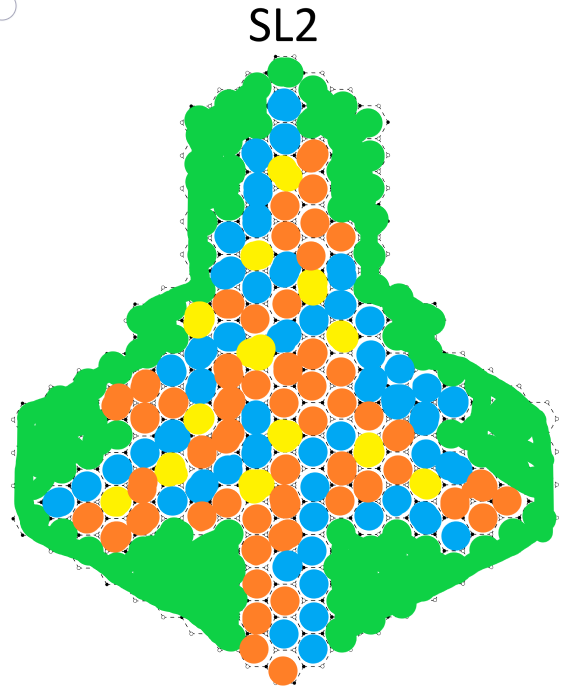
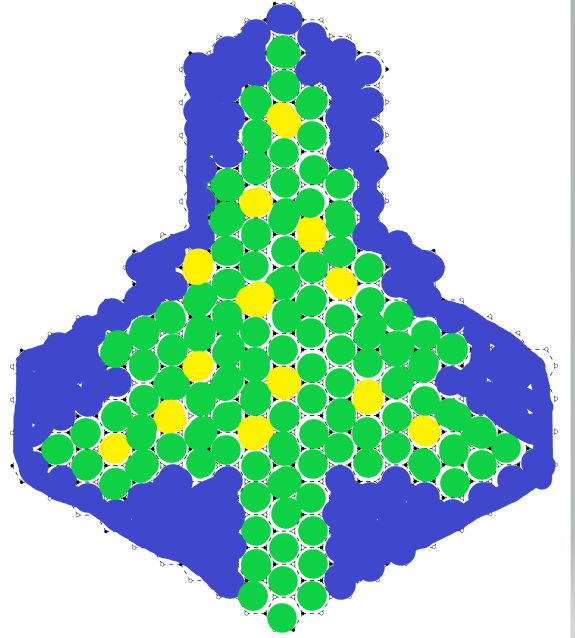
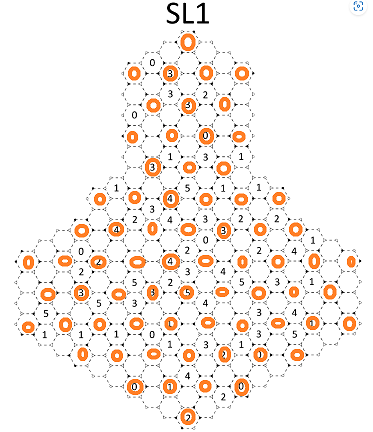
The outcome of this double-slitherlink experiment may or may not be predictable. What we do know for sure is:

  • Similar to the double-slit experiment, we start with a double path (one path in each slitherlink)
  • If all is well, we end up seeing the light
  • Basic slitherlink rules apply

If you feel like these slitherlinks are underconstrained, or just can't see what to do next: further instructions are hidden inside the grids.

sl1 sl2

Hint:

In the puzzle text, feel and can't see are hints towards finding the hidden instructions in the grids.

Improve this question
$\endgroup$
0

3 Answers 3

Reset to default
9
+50
$\begingroup$

We can solve the two slitherlinks only partially without further information.

SL1 SL2

Note that the top half of the first loop and the bottom half of the second loop cannot be uniquely determined.

Then we see

the suspicious dots in the vertices of hexagons and phrases like 'can't see', 'feel' hinting towards a Braille encoding.

Thus we decipher them and get,

SL1-Braille SL2-Braille
"Solve both and overlay the grids. Color all cells enclosed by 1 of the 2 loops dark green and those enclosed by both bright yellow. Top cell of SL1 and bottom cell of SL2 contain 5."

Following the instructions we get,

Final-image
A Christmas Tree!

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Here are the penpa+ links of the grids for you to try the slitherlinks: tinyurl.com/2netdhgm tinyurl.com/2mslptjm $\endgroup$
    – ACB
    Commented Dec 11, 2022 at 10:16
  • $\begingroup$ That's it, well done! $\endgroup$
    – sarsaparilla
    Commented Dec 11, 2022 at 11:20
8
$\begingroup$

To get unique slitherlink solutions, I had to assume that it divides the board into only 2 contiguous pieces, which I could not find as a definite rule. This leads to

enter image description here enter image description here

When comparing the two and colouring using the following rules: green+green = green yellow+yellow = yellow green+yellow=blue yellow+green=orange the result is

enter image description here

Taking that, and swapping the colours, and combining blue and orange, gives you

something that looks like a Christmas tree with lights! enter image description here

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ If you look at the puzzle text carefully (especially the line just above SL1), you shouldn't need to make any assumptions in solving it. $\endgroup$
    – sarsaparilla
    Commented Dec 5, 2022 at 15:26
  • 8
    $\begingroup$ I have no idea how to solve this puzzle but every single corner in each slitherlink is either a solid black disk or an empty white disk. I assume these patters have some meaning but as far as I see it this is not used in your solution anywhere. $\endgroup$
    – quarague
    Commented Dec 5, 2022 at 18:12
  • 1
    $\begingroup$ The hidden information discovered in the other answer give the two clues needed instead of your added assumption (very small change to you SL solution), and more direct instructions for the coloring. $\endgroup$
    – Ben Reiniger
    Commented Dec 11, 2022 at 0:12
2
$\begingroup$

Just a idea/hint/partial answer to get this going:

observation 1

Night /feel / don't see / 6 dots around a hex 'clearly' suggests braille to me

observation 2

When starting at the top and avoiding double use of dots, there are no unused black dots. enter image description here

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Yes, I think that's the way. I had the idea to use (benvyyr) but it didn't occur to me how to group them. Using your image the first part can be deciphered to '(Fbyir)'. (I am too lazy to decode the rest. :) ) [(rot13)] $\endgroup$
    – ACB
    Commented Dec 10, 2022 at 10:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.