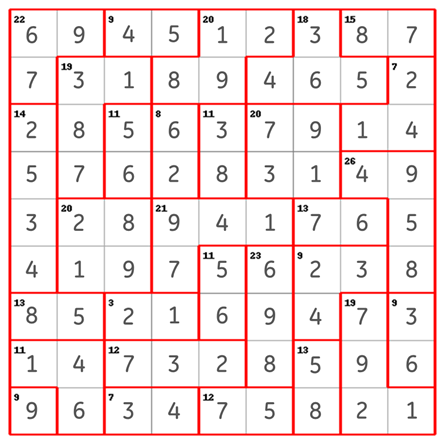
I got:
694512387
731894652
285637914
576283149
328941765
419756238
852169473
147328596
963475821
Here's how I found the first 15 or so values. (terminology note: when I say a group is (x,y,z), that means the group must contain those numbers, but we don't know in what order yet)
1. The bottom left cell is 9.
2. The "22" group in the upper-left can be either (5,8,9) or (6,7,9). There is a nine either way, which can only go in the cell not in the leftmost column.
3. In the bottom-right box, the 9 and 19 and 13 groups add up to 41. A box must sum up to 45, so the bottom cell of the "9" group must be 4.
4. In the right box, the 26 and the 13 and the 9 (minus 4) add up to 44, so the bottom right cell of the "20" group must be 1.
5. Not counting the "9" group, the groups in the top-left and middle-left boxes add up to 86. So the left cell of the "9" group must be 4, and the right cell must be 5.
6. In the upper-right box, the "15" group can be (8,7) or (9,6). But 9 is already taken in this row, so it must be (8,7).
7. In the topmost row, 9,8, and 7 are taken, so the upper left cell must be 6, and the cell below it must be 7.
8. In the bottom-right box, the "13" group can be (4,9) or (5,8) or (6,7). But 9 is already taken in that box; and 6 and 7 are already taken in the leftmost column. So the "13" group must be (8,5).
9. In the bottom-middle box, the "23" group must be (6,8,9).
10. In the bottom-left and bottom-middle boxes, 13 and 11 and 9 and 3 and 12 and 7 and 12 add up to 67. So the lower portions of the "11" and "23" groups must sum to 23. So the upper portions must sum to 11. The top cell of "23" can only be 6, 8, or 9, so the top cell of "11" can only be 5, 3, or 2, so the bottom cell of "11" can only be 6, 8, or 9. But 8 is already taken in that row. Therefore, the top cell of "23" can't be 8. The middle cell of "23" can't be 8 either, so the bottom cell of "23" must be 8.
11. The top cell of "23" can't be 9, so its middle cell must be 9. So the top cell is 6.
12. The "11" group's top cell is 5 and its bottom cell is 6.
13. In the bottom-middle box, the lower "12" group must be, from left to right, 7 and 5.
14. The "7" group straddling the bottom-middle and bottom-left boxes must be (3,4). 4 is already taken in the third column from the left, so the left cell is 3 and the right cell is 4.


9. The U cells are1and2, though the order is unknown without more information. The T cells are5and8or6and7. ...etc. $\endgroup$