Cube Connect Challenges

Question

Cube Connect is a Flow-like mobile puzzle game on the surface of a cube.

Rules of Flow (which is simply Numberlink with colors instead of numbers; copied from Nikoli rules with small modification):

• Connect pairs of the same colored dots with a continuous line.
• Lines go through the center of the cells, entering and exiting through one of the four sides, and never twice through the same cell.
• Lines cannot cross, branch off, or go through the cells with dots.

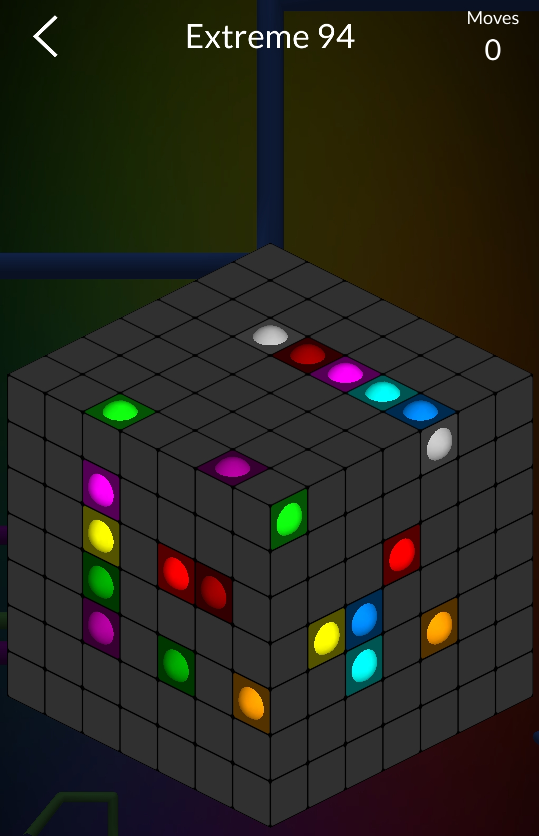
While most of the levels in the game are pretty easy for experienced Flow-ers, there were indeed a few that stumped me for a while. In the same vein of Flow Free Favourites, I wanted to share some of the most challenging and interesting levels in the game.

For each puzzle, the solution is unique and the lines cover every cell on the grid (except the single blocked cell in No. 56).

You can try solving over the images below or on Penpa (which uses letters instead of colored dots): Extreme #56, Extreme #93, Extreme #94. Please at least briefly explain how you approached each puzzle.

Answer 1

These levels are mostly solved by remembering the old "what starts on the perimeter stays on the perimeter" adage, and looking out for places where one or two lines can wrap around a node in a unique way.
Specifically, my initial break-ins were:
Left: Blue and two greens in the top corner, then working clockwise.
Middle: Red and Blue wrapping around Yellow, followed by Green and Crimson wrapping around White.
Right: White going under the four dots due to parity, followed by Yellow and Dark Green wrapping around Purple. (Dark Red's final path was a devilish twist that I'm surprised snapped into place as quickly as it did!)